Angles Of Elevation And Depression

What Is Angle Of Elevation?

The angle of elevation is the angle between a horizontal line from the observer and the line of sight to an object that is above the horizontal line.

In the diagram below, AB is the horizontal line. θ is the angle of elevation from the observer at A to the object at C.

 

What Is Angle Of Depression?

The angle of depression is the angle between a horizontal line from the observer and the line of sight to an object that is below the horizontal line.

In the diagram below, PQ is the horizontal line. θ is the angle of depression from the observer at P to the object at R.

 

How To Define An Angle Of Elevation Or An Angle Of Depression?

Find angles of depression and angles of elevation, and the relationship between them.
Angles of elevation and depression are equal.

• Show Video Lesson

Example:
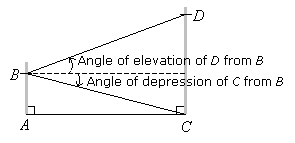
In the diagram below, AB and CD are two vertical poles on horizontal ground. Draw in the angle of elevation of D from B and the angle of depression of C from B.

Solution:

 

How To Solve Word Problems That Involve Angle Of Elevation Or Depression?

Step 1: Draw a sketch of the situation.

Step 2: Mark in the given angle of elevation or depression.

Step 3: Use trigonometry to find the required missing length.

Example:
Two poles on horizontal ground are 60 m apart. The shorter pole is 3 m high. The angle of depression of the top of the shorter pole from the top of the longer pole is 20˚. Sketch a diagram to represent the situation.

Solution:

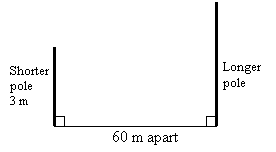
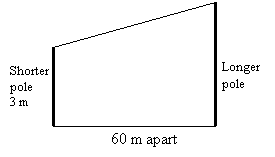
Step 1: Draw two vertical lines to represent the shorter pole and the longer pole.

Step 2: Draw a line from the top of the longer pole to the top of the shorter pole. (This is the line of sight).

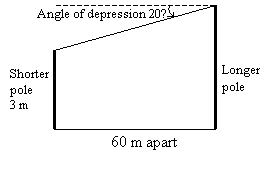
Step 3: Draw a horizontal line to the top of the pole and mark in the angle of depression.

Example:
A man who is 2 m tall stands on horizontal ground 30 m from a tree. The angle of elevation of the top of the tree from his eyes is 28˚. Estimate the height of the tree.

Solution:
Let the height of the tree be h. Sketch a diagram to represent the situation.

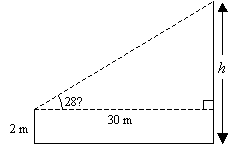
tan 28˚ =

h – 2 = 30 tan 28˚
h = (30 ´ 0.5317) + 2 ← tan 28˚ = 0.5317
h = 17.95

The height of the tree is approximately 18.0 m. (rounded)

How To Solve Word Problems Involving Angles Of Elevation And Angles Of Depression?

Examples:

1. An observer standing on top of a vertical spots a house in the adjacent valley at an angle of depression of 12°. The cliff is 60m tall. How far is the house from the base of the cliff?
2. Buildings A and B are across the street from each other, 35m apart. From a point on the roof of Building A the angle of elevation at the top of Building B is 24°, and the angle of depression of the base of Building B is 34°. How tall is each building?

• Show Video Lesson

How To Solve Word Problems Using Tangent And The Angle Of Depression?

Applications of Trig Functions: Solving for unknown distances.

Example:
How far away is a boat from the lighthouse if the lighthouse is 120° tall and the angle of depression to boat is 15°?

• Show Video Lesson

How To Solve Word Problems Using Tangent And The Angle Of Elevation?

Example:
A boat is 500 meters from the base of a cliff. Jackie, who is sitting in the boat, notices that the angle of elevation to the top of the cliff is 32°15’. How high is the cliff? (Give your answer to the nearest meter).

• Show Video Lesson

Angle Of Elevation And Depression Word Problems

Examples:

1. From a boat on the lake, the angle of elevation to the top of the cliff is 24°22’. If the base of the cliff is 747 feet from the boat, how high is the cliff (to the nearest foot)?
2. From a boat on the river below a dam, the angle of elevation to the top of the dam is 24° 8’. If the dam is 2039 feet above the level of the river, how far is the boat from the base of the dam (to the nearest foot)?
3. If a man is just about to ski down a steep mountain. He estimates the angle of depression from where he is now to the flag at the bottom of the course to be 24°. He knows that he is 800 feet higher than the base of the course. How long is the path that he will ski? (Round to the nearest foot)
4. a) A man at ground level measures the angle of elevation to the top of the building to be 67°. If at this point, he is 15 feet away from the building, what is the height of the building?
   b) The same man now stands atop a building. He measures the angle of elevation to the building across the street to be 27° and the angle of depression (to the base of the building across the street) to be 31°. If the two buildings are 50 feet apart, how tall is the taller building?

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.