Parallel Vectors
In these lessons, we will learn how to determine if the given vectors are parallel.
Related Pages
Vectors
Equal Vectors
Vector Multiplication
Vector Geometry
A vector is a quantity that has both magnitude and direction.
What Are Parallel Vectors?
Vectors are parallel if they have the same direction.
Both components of one vector must be in the same ratio to the corresponding components of the parallel vector.
Example:
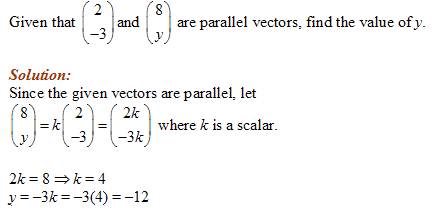
How To Define Parallel Vectors?
Two vectors are parallel if they are scalar multiples of one another.
If u and v are two non-zero vectors and u = cv, then u and v are parallel.
The following diagram shows several vectors that are parallel.

How To Determine If The Given 3-Dimensional Vectors Are Parallel?
Example: Determine which vectors are parallel to v = <-3, -2, 5>
What Are The Conditions For Two Lines To Be Parallel Given Their Vector Equations?
Lines are parallel if the direction vectors are in the same ratio.
Example: If the lines l1: \(r = \left( {\begin{array}{*{20}{c}}1\\{ - 5}\\7\end{array}} \right) + \lambda \left( {\begin{array}{*{20}{c}}{a - 1}\\{ - a - 1}\\b\end{array}} \right)\) and l2: \(r = \left( {\begin{array}{*{20}{c}}9\\3\\{ - 8}\end{array}} \right) + \mu \left( {\begin{array}{*{20}{c}}{2a}\\{3 - 5a}\\{15}\end{array}} \right)\).
Find the values of a and b.
What Is A Vector Is, How To Add And How To Prove Vectors Are Parallel And Collinear?
Examples:
- A, B, C are midpoints of their respective lines. Find the vector OB.
- N = midpoint of OB, M = midpoint of OA. Show that MN is parallel to AB.
- Given the vectors, prove that the three given points are collinear.
How To Answer A Question That Involves Vectors - Lines, Parallel, Perpendicular & Intersection?
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.