Calculus - Power Rule, Sum Rule, Difference Rule
In these lessons, we learn the Power Rule, the Constant Multiple Rule, the Sum Rule and the Difference Rule.
Related Pages
Calculus: Derivatives
Derivative Rules
Calculus: Chain Rule
Calculus: Product Rule
Calculus Lessons
The following diagram gives the basic derivative rules that you may find useful: Constant Rule, Constant Multiple Rule, Power Rule, Sum Rule, Difference Rule, Product Rule, Quotient Rule, and Chain Rule. Scroll down the page for more examples and solutions.
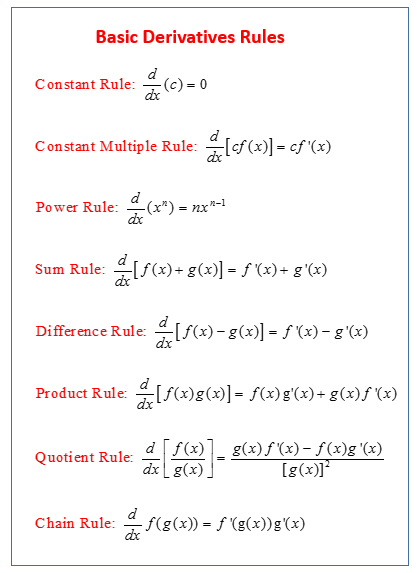
It is not always necessary to compute derivatives directly from the definition. Instead, several rules have been developed for finding derivatives without having to use the definition directly. These formulas greatly simplify the task of differentiation.
Definition of the Power Rule
The Power Rule of Derivatives gives the following:
For any real number n,
the derivative of f(x) = xn is f ’(x) = nxn-1
which can also be written as
![]()
Example:
Differentiate the following:
a) f(x) = x5
b) y = x100
c) y = t6
Solution:
a) f’’(x) = 5x4
b) y’ = 100x99
c) y’ = 6t5
We have included a Derivative or Differentiation calculator at the end of this page. It can show the steps involved including the power rule, sum rule and difference rule.
Derivative of the function f(x) = x
Using the power rule formula, we find that the derivative of the function f(x) = x would be one.
The derivative of f(x) = x is f ’(x) = 1
which can also be written as
![]()
Example:
Differentiate f(x) = x
Solution:
f ’(x) = f ’(x1) = 1x0 = 1
Derivative of a Constant Function
Using the power rule formula, we find that the derivative of a function that is a constant would be zero.
For any constant c,
The derivative of f(x) = c is f ’(x) = 0
which can also be written as
![]()
Example:
Differentiate the following:
a) f(3)
b) f(157)
Solution:
a) f ‘(3) = f ‘(3x0) = 0(3x-1) = 0
b) f ‘(157) = 0
The Constant Multiple Rule
The constant multiple rule says that the derivative of a constant value times a function is the constant times the derivative of the function.
If c is a constant and f is a differentiable function, then
![]()
Example:
Differentiate the following:
a) y = 2x4
b) y = –x
Solution:

The Sum Rule
The Sum Rule tells us that the derivative of a sum of functions is the sum of the derivatives.
If f and g are both differentiable, then
![]()
The Sum Rule can be extended to the sum of any number of functions.
For example (f + g + h)’ = f’ + g’ + h’
Example:
Differentiate 5x2 + 4x + 7
Solution:
![]()
The Difference Rule
The Difference Rule tells us that the derivative of a difference of functions is the difference of the derivatives.
If f and g are both differentiable, then
![]()
Example:
Differentiate x8 – 5x2 + 6x
Solution:
![]()
Proof of the Power Rule for Derivatives
An explanation and some examples.
How to find derivatives using rules?
Differentiation Techniques: Constant Rule, Power Rule, Constant Multiple Rule, Sum and Difference Rule
Basic Derivative Rules - The Shortcut Using the Power Rule
In this video, we look at finding the derivative of some very simple functions by using the power rule.
Power Rule and Derivatives, A Basic Example
This video uses the power rule to find the derivative of a function.
Power Rule and Derivatives, Example #2
This video uses the power rule to find the derivative of a function.
Power Rule and Derivatives, Example #3
This video uses the power rule to find the derivative of a function.
How to determine the derivatives of simple polynomials?
How to differentiate using the extended power rule?
The extended power rule involves using the chain rule with the power rule.
Examples of using the extended power rule
Derivative Calculator
The following derivative calculator can show you the steps and rules used to get the derivative of the given function. Use it to check your answers.
| Step By Step Calculus | ||
|---|---|---|
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.