Simplifying Square Roots (or Radicals)
In these lessons we will look into two methods that can be used to simplify square roots (or radicals): the perfect square method and the prime factorization method.
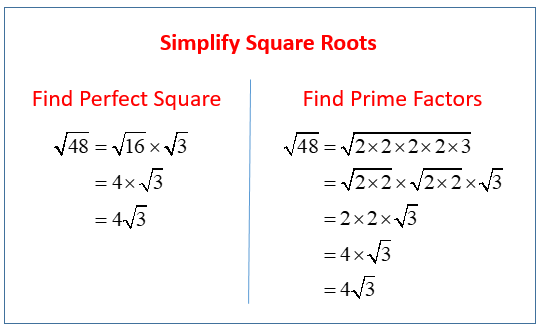
The following examples show how to simplify square roots: Find Perfect Square, Find Prime Factors. Scroll down the page for examples and solutions.
Simplify square roots using the perfect square method
The steps involved are:
- Find the perfect square(s) that will divide the number in the square root.
- Write the number as a factor of the perfect square(s).
- Reduce the perfect squares.
Example:
Simplify ![]()
Solution:
Step 1: The perfect square 16 divides 48
Step 2: Write 48 as a factor of 16
48 = 16 × 3
Step 3: Reduce square root of 16
How to simplify square roots using the perfect square method?
The following video shows more examples of simplifying square roots using the perfect square method.
The perfect square method is suitable for small numbers for example less than 1000. For bigger numbers
the prime factorization method may be better.
It would be useful for you to memorize the first five perfect squares of prime numbers.
12 = 1, 22 = 4, 32= 9, 52 = 25, 72 = 49, 112 = 121
Step 1: Factor out the perfect squares
Step 2: Separate perfect squares using product of square roots property
Step 3: Simplify
Examples:
Simplify the following square roots:
a) square root of 72
b) square root of 288
c) square root of 108
How to simplify square roots by factoring out perfect squares?
Example:
Simplify the following square roots:
a) square root of 60
b) square root of 108
Simplify square roots using the prime factorization method
The steps involved are:
1. Break the number in the square root into prime factors
2. For each pair of factors, “take one out” of the square root sign
3. The remaining factors in the square root sign are multiplied together.
Example:
Simplify ![]()
Solution:
Step 1. Break the number 12 into prime factors
12 = 2 × 2 × 3
Step 2: Take 2 out of the square root sign
![]()
Example:
Simplify ![]()
Solution:
Step 1. Break the number 90 into prime factors
90 = 2 × 3 × 3 × 5
Step 2: Take 3 out of the square root sign
Step 3: Multiply 2 and 5
How to simplifying square roots using the prime factorization method?
The following video shows more examples of simplifying square roots using the prime factorization method.
Step 1: Factor into product of primes
Step 2: Circle the pairs of factors
Step 3: Remove the pairs and multiply by each number removed.
Example:
Simplify the following square roots:
a) square root of 18
b) square root of 420
Examples of simplifying square roots using the prime factorization.
Example:
Simplify the following square roots:
a) square root of 180
b) square root of 200
How to use prime factorization to simplify square roots?
Example:
Simplify the following square roots:
a) square root of 84
b) square root of 392
How to Simplify Square Roots With Fractions?
How to deal with square roots in the denominator of a fraction.
Example:
Simplify the following square roots:
a) \(\sqrt {\frac{7}{3}} \)
b) \(\sqrt {\frac{24}{5}} \)
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.