Solving Exponential Equations With Different Bases

Related Pages
Solving Exponential Equations With The Same Base
Logs And Exponential Equations
More PreCalculus Lessons
More Algebra Lessons
Grade 10 Math Lessons

The following diagram shows the steps to solve exponential equations with different bases. Scroll down the page for more examples and solutions.

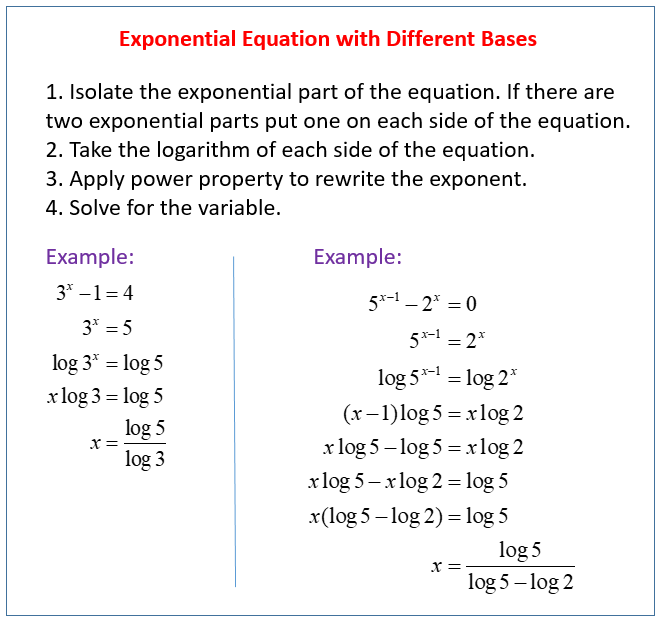
How to solve exponential equations with different bases?

Sometimes we are given exponential equations with different bases on the terms. In order to solve these equations we must know logarithms and how to use them with exponentiation. We can access variables within an exponent in exponential equations with different bases by using logarithms and the power rule of logarithms to get rid of the base and have just the exponent.

How to solve exponential equations using logarithms?

1. Isolate the exponential part of the equation. If there are two exponential parts put one on each side of the equation.
   
2. Take the logarithm of each side of the equation.
   
3. Solve for the variable.
   
4. Check your solution graphically.
   

Example:
Solve the exponential equations. Round to the hundredths if needed.
(a) 7^x - 1 = 4
(b) 3•2^x - 2 = 13
(c) (2/3)^x = 5^{3 - x}
(d) 5^{x - 3} = 3^{2x + 1}

• Show Video Lesson

How to solve exponential equations with different bases?

When it’s not convenient to rewrite each side of an exponential equation so that it has the same base, you do the following:

1. Take the log (or ln) of both sides
   
2. Apply power property
   
3. Solve for the variable
   

Example:
Solve for x.
a) 6^x = 42
b) 7^x = 20
c) 8^{2x - 5} = 5^{x + 1}
d) 3^x = 5^{x - 1}

• Show Video Lesson

How to use change of base formula to solve basic exponential equations?

Example:
Solve by writing as a log equation and then using the change of base formula. Round to 4 decimal places.
a) 5^x = 476
b) 4•3^x = 984

• Show Video Lesson

How to solve exponential and logarithmic equations?

Principle used: log_aa^g(x) = g(x)

Examples:

1. Solve for x: 8^{8x + 7} = 9
   
2. Solve for x:
   a) 6^{1 - 2x} = 3
   b) 1 + 3(4^{2x + 3}) = 8
   
3. Solve for x: log₂4x + log₂x = 2
   
4. Solve for x:
   a) log₃5 + log₂(x + 4) = 2
   b) log₄x + log₄(x + 3) = 1

• Show Video Lesson

How to solve exponential equations that are not one-to-one?

Examples:
3^{4x - 5} = 17

• Show Video Lesson

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.