Examples With Trigonometric Functions: Even, Odd Or Neither
In these lessons, we will look at how to determine whether a Trigonometric Function is Even, Odd or Neither.
Related Pages
Lessons On Trigonometry
Inverse trigonometry
Trigonometric Functions
Even And Odd Functions
What Is An Even Function?
An even function is symmetric (by reflection) about the y-axis , i.e.
f(-x) = f(x)
What Is An Odd Function?
An odd function is symmetric (by 180° rotation) about the origin, i.e.
f(-x) = -f(x)
The following table shows the Even Trigonometric Functions and Odd Trigonometric Functions. Scroll down the page for more examples and step by step solutions.
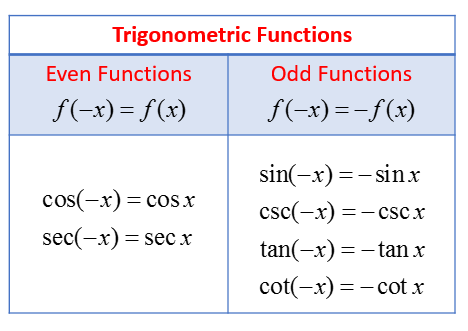
Even Trigonometric Functions And Identities
Cosine function is even. cos(-x) = cos x
Secant function is even. sec(-x) = sec x
Odd Trigonometric Functions And Identities
Sine function is odd. sin(-x) = - sin x
Cosecant function is odd. csc(-x) = - csc x
Tangent function is odd. tan(-x) = - tan x
Cotangent function is odd. cot(-x) = - cot x
Determine Whether A Trigonometric Function Is Odd, Even, Or Neither
Examples with Trigonometric Functions: Even, Odd or Neither
Cosine function, Secant function, Sine function, Cosecant function, Tangent function, and
Cotangent function
Examples With Trigonometric Functions: Even, Odd Or Neither
Example 2
Determine whether the following trigonometric function is Even, Odd or Neither
a) f(x) = sec x tan x
Example 3
b) g(x) = x4 sin x cos2x
Example 4
c) h(x) = cos x + sin x
How To Use The Even-Odd Properties Of The Trigonometric Functions?
Example: Find the exact value using even-odd properties.
(a) sin(-30°)
(b) cos(-3π/4)
(c) tan(-π/4)
How To Determine Trig Function Values Based Upon Whether The Function Is Odd Or Even?
Determine each function value.
If cos(x) = 0.5, then cos(-x) = ___.
If sin(x) = 0.15, then sin(-x) = ___.
If tan(-x) = -3, then tan(x) = ___.
If sec(-x) = 1.4, then sec(x) = ___.
How To Use Even Or Odd Properties To Evaluate Trig Functions?
Evaluate the trigonometric function by first using even/odd properties to rewrite the expression
with a positive angle. Give an exact answer Do not use a calculator.
sin(-45°)
sec(210°)
cos(-π6)
csc(-3π/2)
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.