CIE May 2024 9709 Pure Maths Paper 12 (9709/12/m/j/24)
This is part of a collection of videos showing step-by-step solutions for CIE A-Level Mathematics past papers.
This page covers Questions and Worked Solutions for CIE Pure Maths Paper 1 May/June 2024, 9709/12.
Related Pages
May 2024 Pure Maths 1 9709/12
May 2024 Pure Maths 1 9709/13
More A Levels Past Papers
9709/12/M/J/24, is a Cambridge International AS & A Level Mathematics paper from the May/June 2024 series. Specifically, it is Paper 11 from the Pure Mathematics 1 (P1) component.
Preparing for the Cambridge International AS & A Level Mathematics Paper 1 (9709/12) from the May/June 2024 exam series requires a solid understanding of Pure Mathematics topics and effective exam strategies.
This page covers the Question Paper and Mark Scheme for CIE Pure Maths Paper 1 May/June 2024, 9709/12. It includes a video that will give you a detailed answer for each question.
Jump to: Question Paper | Mark Scheme | Worked Solutions Video
Question Paper Pure Maths Paper 1 May/June 2024, 9709/12
Jump to: Question Paper | Mark Scheme | Worked Solutions Video
Mark Scheme Pure Maths Paper 1 May/June 2024, 9709/12
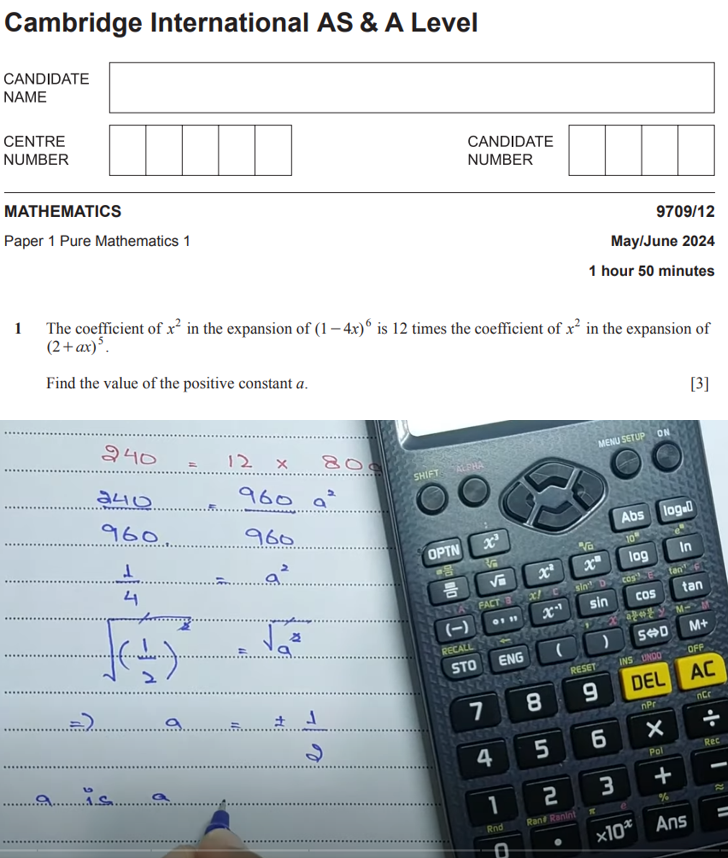
Worked Solutions Pure Maths Paper 1 May/June 2024, 9709/12
The 9709/12 paper focuses on Pure Mathematics and covers the following key topics:
- Algebra:
Quadratic equations, polynomials, and factor/remainder theorems.
Functions, domain, range, and inverse functions.
Exponential and logarithmic functions. - Coordinate Geometry:
Equations of lines, circles, and curves.
Intersection points and tangents. - Sequences and Series:
Arithmetic and geometric progressions.
Summation of series. - Trigonometry:
Trigonometric identities and equations.
Sine and cosine rules. - Differentiation:
Rules of differentiation (e.g., chain rule, product rule, quotient rule).
Applications of differentiation (e.g., gradients, tangents, stationary points). - Integration:
Indefinite and definite integrals.
Applications of integration (e.g., area under a curve).
Targeted Practice:
- Past Papers are Gold: Past papers are your best friend when it comes to exam preparation. Obtain past papers specifically for the 9709/12 exam, ideally from recent years.
- Practice Makes Perfect: Work through these past papers under timed conditions to simulate the actual exam environment. This will help you get comfortable with the format, question types, and time constraints.
- Analyze and Learn: Don’t just solve the questions; analyze your mistakes and understand the underlying concepts. Use the mark schemes to see how marks are awarded and identify areas where you need to improve.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.