Adding Indices

Related Pages
Exponents
Rules Of Exponents
More Math Lessons

The following diagrams show the rules of indices or laws of indices. Scroll down the page for more examples and solutions on how to use the rules of indices.

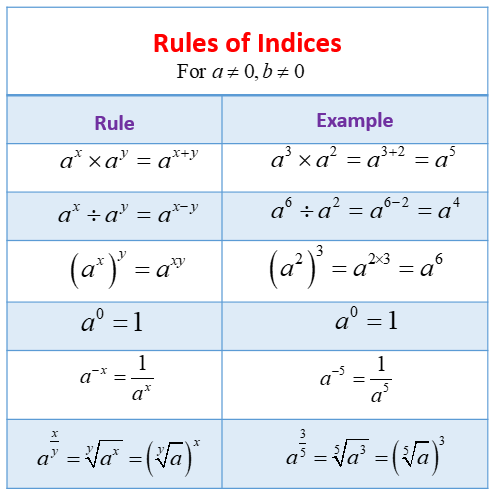
When multiplying numbers in exponent notation with the same base, we can add the exponents.

Consider:
a² × a³ = (a × a) × (a × a × a)
= a^{2 + 3}
= a⁵

This is the first law of exponents:
a^m × aⁿ = a^{m + n}

Example:
Simplify the following; give your answers in exponent form
a) 3³ × 3²
b) x⁵ × x³

Solution:
a) 3³ × 3² = 3^{3 + 2}
= 3⁵
b) x⁵ × x³ = x^{5 + 3}
= x⁸

Common Error: The first law of exponents does NOT apply to addition of numbers in exponent notation.

a^m + aⁿ ≠ a^{m + n}

Scroll down the page for more examples and solutions of the first law of exponents and also the other laws of exponents.

Index rules - add and subtract indices

Basic look at the first two index laws.

Properties of Exponents

The Product Rule: a^m × aⁿ = a^{m + n}
The Quotient Rule: a^m ÷ aⁿ = a^{m - n}
The Power Rule: (a^m)ⁿ = a^mn

Example:
Simplify
(3ab⁴)(2a²b)³
(x²y⁴/2xz)³

• Show Video Lesson

Example of adding exponents

(2a⁴)(5¹¹)

• Show Video Lesson

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.