Advanced Trigonometric Identities
Related Pages
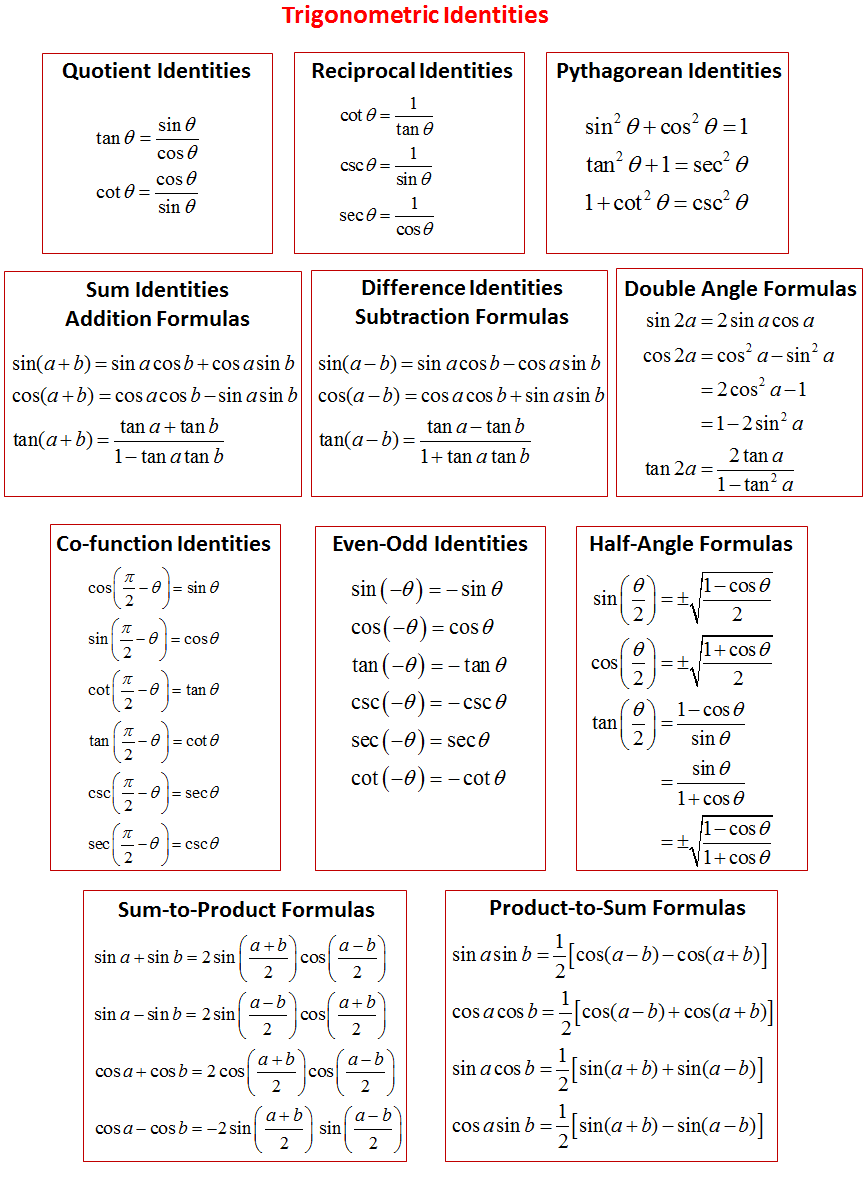
Basic Trigonometric Identities
Trigonometric Graphs
Trigonometric Functions
Lessons On Trigonometry
In these lessons, we will learn to use trigonometric identities, including cofunction identities, power reducing formulas and half-angle identities.
Trigonometric identities are powerful tools for simplifying expressions, solving equations, and proving mathematical statements.
The following tables give some Trigonometric Identities. Scroll down the page for examples and solutions on how to use the Trig Identities.
Trigonometry Worksheets
Practice your skills with the following worksheets:
Printable & Online Trigonometry Worksheets
-
Sum Identities
These identities allow you to express trigonometric functions of the sum of two angles in terms of trigonometric functions of the individual angles:
sin(A+B)=sinAcosB+cosAsinB
cos(A+B)=cosAcosB−sinAsinB
tan(A+B)=tanA+tanB1−tanAtanB -
Difference Identities
These identities allow you to express trigonometric functions of the difference of two angles in terms of trigonometric functions of the individual angles:
sin(A−B)=sinAcosB−cosAsinB
cos(A−B)=cosAcosB+sinAsinB
tan(A−B)=tanA−tanB1+tanAtanB -
Double-Angle Identities
These are special cases of the sum identities where the two angles are equal (A = B = θ):
sin(2θ)=2sinθcosθ
cos(2θ)=cos2θ−sin2θ
cos(2θ)=1−2sin2θ
cos(2θ)=2cos2θ−1
tan(2θ)=2tanθ1+tan2θ -
Half-Angle Identities
These identities express trigonometric functions of an angle that is half of another angle θ2 in terms of trigonometric functions of the full angle θ:
sin(θ2)=±√1−cosθ2
cos(θ2)=±√1+cosθ2
tan(θ2)=±√1−cosθ1+cosθ=sinθ1+cosθ=1−cosθsinθ -
Product-to-Sum Identities
These identities allow you to rewrite the product of two trigonometric functions as a sum or difference:
sinAcosB=12[sin(A+B)+sin(A−B)]
cosAsinB=12[sin(A+B)−sin(A−B)]
cosAcosB=12[cos(A+B)+cos(A−B)]
sinAsinB=12[cos(A−B)−cos(A+B)] -
Sum-to-Product Identities
These identities do the reverse of the product-to-sum identities, allowing you to rewrite the sum or difference of two trigonometric functions as a product:
sinA+sinB=2sin(A+B2)cos(A−B2)
sinA−sinB=2cos(A+B2)sin(A−B2)
cosA+cosB=2cos(A+B2)cos(A−B2)
cosA−cosB=−2sin(A+B2)sin(A−B2)
Using a Cofunction Identity
Cofunction identities and how to determine cofunctions given a function value.
Cofunction Identities - Solving Trigonometric Equations
How to use cofunction identities to solve trigonometric equations?
Example:
Find a possible acute angle solution.
a) cos(2θ + 16°) = sin(θ + 11°)
b) cot(θ) = tan(θ + π/6)
Power Reducing Formulas - Trigonometric Identities
How to use power reducing formulas to simplify trigonometric expressions?
It contains the power reducing trigonometric identities for sine, cosine, and tangent.
Examples:
sin4(x)
sin2(x)cos2(x)
sin4(x)cos2(x).
Half Angle Identities to Evaluate Trigonometric Expressions
Find the exact value of the following:
tan(105°)
How to use Half Angle Identities to Evaluate Trigonometric Expressions
Find the exact value of the following:
sin(a/2) if cos a = 3/5 for 0° ≤ a ≤ 90°
Try out our new and fun Fraction Concoction Game.
Add and subtract fractions to make exciting fraction concoctions following a recipe. There are four levels of difficulty: Easy, medium, hard and insane. Practice the basics of fraction addition and subtraction or challenge yourself with the insane level.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.