Interest Word Problems
This lesson is part of a series of lessons for the quantitative reasoning section of the GRE revised General Test. In this lesson, we will learn:
- Interest Word Problems
- Simple Interest
- Compound Interest
The following tables give the Formulas for Simple Interest, Compound Interest, and Continuously Compounded Interest. Scroll down the page for more examples and solutions.
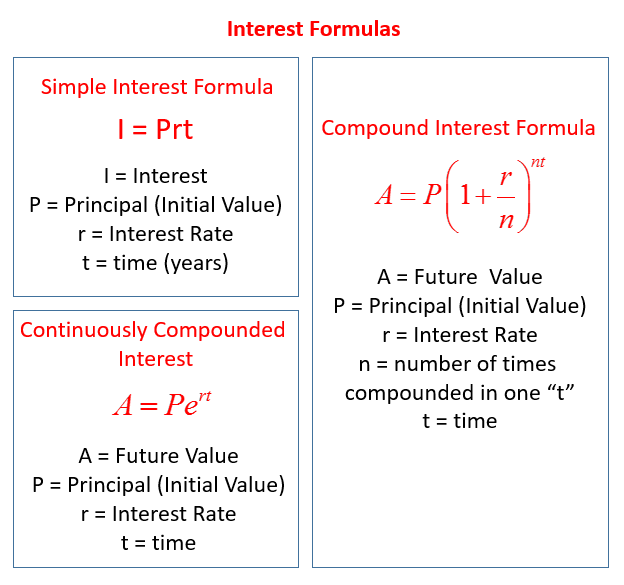
Simple Interest
Some applications involve computing interest earned on an investment during a specified time period. The interest can be computed as simple interest or compound interest.
Simple interest is based only on the initial deposit, which serves as the amount on which interest is computed, called the principal, for the entire time period. The simple interest is given by the formula
I = Prt
where I is the interest, P is the principal (starting amount), r is the rate as a decimal and t is the time in years.
The following video explains how to solve interest problems using the simple interest formula.
The following video provides an example of how to determine an account balance after t years earning simple interest.
The following video explains how to determine the amount of money in different accounts earning different simple interest given the total interest earned.
If the amount P is invested at a simple annual interest rate of r percent, then the value V of the investment at the end of t years is given by the formula
where V and P are in dollars.
Example:
If $10,000 is invested at a simple annual interest rate of 6 percent, what is the value of the
investment after two years?
According to the formula for simple interest, the value of the investment after 2 years is
V = ($10,000)(1 + 0.06(2)) = $11,200
Compound Interest
In the case of compound interest, interest is added to the principal at regular time intervals, such as annually, quarterly, and monthly. Each time interest is added to the principal, the interest is said to be compounded. After each compounding, interest is earned on the new principal, which is the sum of the preceding principal and the interest just added.
If the amount P is invested at an annual interest rate of r percent, compounded annually, then the value V of the investment at the end of t years is given by the formula
If the amount P is invested at an annual interest rate of r percent, compounded n times per year, then the value V of the investment at the end of t years is given by the formula
This video provides an example of compounded interest. Interest is compounded quarterly.
The following video shows how to find the future value of an account that earns interest compounded daily.
The following video shows how to find the future value of an account that earns interest compounded monthly. (you do not need to know about the continuous compound interest)
How much money would have to be invested at 6% interest compounded weekly to be worth $1,500 at the end of 15 years?
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.