Algebra 2 Common Core Regents Exam - June 2017
Related Topics:
More Lessons for the Regents High School Exam
More Lessons for Algebra
High School Math based on the topics required for the Regents Exam conducted by NYSED. The following are the worked solutions for the Algebra 2(Common Core) Regents High School Examination June 2017.
Algebra 2 Common Core Regents New York State Exam - June 2017, Questions 1 - 39
The following are questions from the past paper Regents High School Algebra 2, June 2017 Exam (pdf). Download the questions and try them, then scroll down the page to check your answers with the step by step solutions.
Algebra 2 - June 2017 Regents - Questions and solutions 1 - 12
1 The graph of the function p(x) is sketched below.
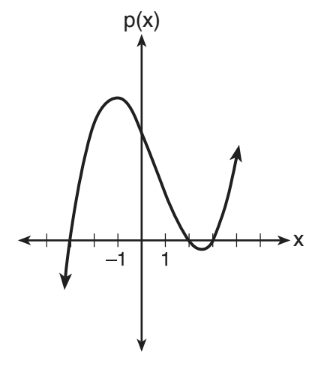
Which equation could represent p(x)?
2 What is the solution to 8(2x + 3) = 48?
3 Cheap and Fast gas station is conducting a consumer satisfaction survey. Which method of collecting data would most likely lead to a biased sample?
4 The expression 6xi3(-4xi + 5) is equivalent to
5 If f(x) = 3|x| - 1 and g(x) = 0.03x3 - x + 1, an approximate solution for the equation f(x) = g(x) is
6 Given the parent function p(x) cos x, which phrase best describes the transformation used to obtain the graph of g(x) = cos(x + a) - b, if a and b are positive constants?
7 The solution to the equation 4x2 + 98 = 0 is
8 Which equation is represented by the graph shown below?
9 A manufacturing company has developed a cost model, C(x) = 0.15x3 + 0.01x2 + 2x + 120, where x is the number of items sold, in thousands. The sales price can be modeled by S(x) = 30 - 0.01x. Therefore, revenue is modeled by R(x) = x·S(x).
The company’s profit, P(x) = R(x) - C(x), could be modeled by
10 A game spinner is divided into 6 equally sized regions, as shown in the diagram below.
For Miles to win, the spinner must land on the number 6. After spinning the spinner 10 times, and losing all 10 times, Miles complained that the spinner is unfair. At home, his dad ran 100 simulations of spinning the spinner 10 times, assuming the probability of winning each spin is 1/6. The output of the simulation is shown in the diagram below.
11 Which binomial is a factor of x4 - 4x2 - 4x + 8?
12 Given that sin2θ + cos2θ = 1 and sin θ = -√2/5, what is a possible value of cos θ?
Algebra 2 - June 2017 Regents - Questions and solutions 13 - 24
13 A student studying public policy created a model for the population of Detroit, where the population decreased 25% over a decade. He used the model P 714(0.75)d, where P is the population, in thousands, d decades after 2010. Another student, Suzanne, wants to use a model that would predict the population after y years. Suzanne’s model is best represented by
14 The probability that Gary and Jane have a child with blue eyes is 0.25, and the probability that they have a child with blond hair is 0.5. The probability that they have a child with both blue eyes and blond hair is 0.125. Given this information, the events blue eyes and blond hair are
15 Based on climate data that have been collected in Bar Harbor, Maine, the average monthly temperature, in degrees F, can be modeled by the equation B(x) = 23.914sin(0.508x - 2.116) + 55.300. The same governmental agency collected average monthly temperature data for Phoenix, Arizona, and found the temperatures could be modeled by the equation P(x) = 20.238sin(0.525x - 2.148) + 86.729. Which statement can not be concluded based on the average monthly temperature models x months after starting data collection?
15 The loudness of sound is measured in units called decibels (dB). These units are measured by first assigning an intensity I0 to a very soft sound that is called the threshold sound. The sound to be measured is assigned an intensity, I, and the decibel rating, d, of this sound is found using d = 10 log I/I0. The threshold sound audible to the average person is 1.0 × 10-12 W/m2 (watts per square meter). Consider the following sound level classifications:
16 For x ≠ 0, which expressions are equivalent to one divided by the sixth root of x?
Assuming both boys are accurate in describing the population of their ant farms, after how many months will they both have approximately the same number of ants?
17 A parabola has its focus at (1,2) and its directrix is y = -2. The equation of this parabola could be
18 The function p(t) = 110e0.03922t models the population of a city, in millions, t years after 2010. As of today, consider the following two statements:
19 Ren multiplied both sides by the least common denominator. Which statement is true?
20 Given f(9) = -2, which function can be used to generate the sequence -8,-7.25,-6.5,-5.75,…?
21 The function f(x) = 2-0.25x·sin(π/2 x) represents a damped sound wave function. What is the average rate of change for this function on the interval [-7,7], to the nearest hundredth?
22 Mallory wants to buy a new window air conditioning unit. The cost for the unit is $329.99. If she plans to run the unit three months out of the year for an annual operating cost of $108.78, which function models the cost per year over the lifetime of the unit, C(n), in terms of the number of years, n, that she owns the air conditioner?
23 The expression can be rewritten as
24 Jasmine decides to put $100 in a savings account each month. The account pays 3% annual interest, compounded monthly. How much money, S, will Jasmine have after one year?
Algebra 2 - June 2017 Regents - Questions and solutions 25 - 37
25 Given r(x) = x3 - 4x2 + 4x - 6, find the value of r(2).
What does your answer tell you about x - 2 as a factor of r(x)? Explain.
26 The weight of a bag of pears at the local market averages 8 pounds with a standard deviation of 0.5 pound. The weights of all the bags of pears at the market closely follow a normal distribution. Determine what percentage of bags, to the nearest integer, weighed less than 8.25 pounds.
27 Over the set of integers, factor the expression 4x3 - x2 + 16x - 4 completely.
28 The graph below represents the height above the ground, h, in inches, of a point on a triathlete’s bike wheel during a training ride in terms of time, t, in seconds.
Identify the period of the graph and describe what the period represents in this context.
29 Graph y = 400(.85)2x - 6 on the set of axes below.
30 Solve algebraically for all values of x:
√(x - 4) + x = 6
31 Write as a single term with a rational exponent.
32 Data collected about jogging from students with two older siblings are shown in the table below.
Using these data, determine whether a student with two older siblings is more likely to jog if one sibling jogs or if both siblings jog. Justify your answer.
33 Solve the following system of equations algebraically for all values of x, y, and z:
x + y + z = 1
2x + 4y + 6z = 2
-x + 3y - 5z = 11
34 Jim is looking to buy a vacation home for $172,600 near his favorite southern beach. The formula to compute a mortgage payment, M, is M P • where P is the principal amount of the loan, r is the monthly interest rate, and N is the number of monthly payments. Jim’s bank offers a monthly interest rate of 0.305% for a 15-year mortgage.
With no down payment, determine Jim’s mortgage payment, rounded to the nearest dollar.
Algebraically determine and state the down payment, rounded to the nearest dollar, that Jim needs to make in order for his mortgage payment to be $1100.
35 Graph y = log2(x + 3) - 5 on the set of axes below. Use an appropriate scale to include both intercepts.
Describe the behavior of the given function as x approaches 3 and as x approaches positive infinity.
36 Charlie’s Automotive Dealership is considering implementing a new check-in procedure for customers who are bringing their vehicles for routine maintenance. The dealership will launch the procedure if 50% or more of the customers give the new procedure a favorable rating when compared to the current procedure. The dealership devises a simulation based on the minimal requirement that 50% of the customers prefer the new procedure. Each dot on the graph below represents the proportion of the customers who preferred the new check-in procedure, each of sample size 40, simulated 100 times.
Assume the set of data is approximately normal and the dealership wants to be 95% confident of its results. Determine an interval containing the plausible sample values for which the dealership will launch the new procedure. Round your answer to the nearest hundredth.
Forty customers are selected randomly to undergo the new check-in procedure and the proportion of customers who prefer the new procedure is 32.5%. The dealership decides not to implement the new check-in procedure based on the results of the study. Use statistical evidence to explain this decision.
37 A radioactive substance has a mass of 140 g at 3 p.m. and 100 g at 8 p.m. Write an equation in the form A = A0(1/2)t/h that models this situation, where h is the constant representing the number of hours in the half-life, A0 is the initial mass, and A is the mass t hours after 3 p.m.
Using this equation, solve for h, to the nearest ten thousandth.
Determine when the mass of the radioactive substance will be 40 g. Round your answer to the nearest tenth of an hour.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.