AP Calculus BC 2010 Questions And Answers

AP Calculus BC is a challenging, college-level course in calculus that builds upon the concepts learned in AP Calculus AB. It covers topics typically found in the first two semesters of college calculus, providing a more comprehensive understanding of calculus.

Questions And Worked Solutions For AP Calculus BC 2010

Click on the following image to get the complete paper of AP Calculus BC 2010 Free Response Questions. Scroll down the page for step-by-step solutions.

 
AP Calculus BC 2010 Free Response Questions - Complete Paper (pdf)

AP Calculus BC 2010 Free Response Questions - Scoring Guide (pdf)

• Show Solutions for Q1

• Show Solutions for Q2

• Show Solutions for Q3

• Show Solutions for Q4

• Show Solutions for Q5

• Show Solutions for Q6

1. There is no snow on Janet’s driveway when snow begins to fall at midnight. From midnight to 9 A.M., snow accumulates on the driveway at a rate modeled by f(t) = 7te^costcubic feet per hour, where t is measured in hours since midnight. Janet starts removing snow at 6 A.M. (t = 6). The rate g(t), in cubic feet per hour, at which Janet removes snow from the driveway at time t hours after midnight is modeled by
2. A zoo sponsored a one-day contest to name a new baby elephant. Zoo visitors deposited entries in a special box between noon (t = 0) and 8 P.M. (t = 8). The number of entries in the box t hours after noon is modeled by a differentiable function E for 0 ≤ t ≤ 8. Values of E(t), in hundreds of entries, at various times t are shown in the table above.

3. A particle is moving along a curve so that its position at time t is (x(t),y(t)), where x(t) = t² -4t + 8 and y(t) is not explicitly given.
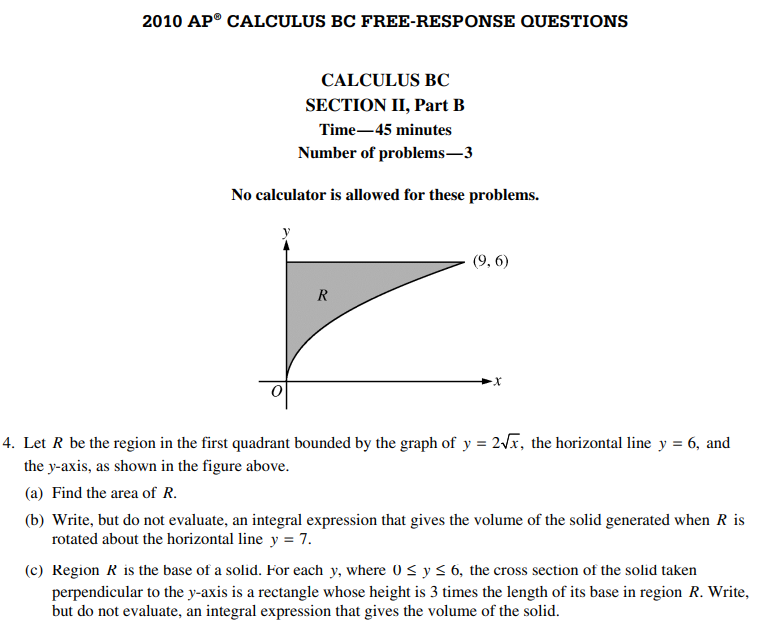
4. Let R be the region in the first quadrant bounded by the graph of y = 2√x the horizontal line y = 6, and the y-axis, as shown in the figure above.
5. Consider the differential equation dy/dx = 1. Let y = f(x) be the particular solution to this differential equation with the initial condition f(1) = 0. For this particular solution, f(x) < 1 for all values of x
6. The function f, defined above, has derivatives of all orders. Let g be the function defined by

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.