Applications of Exponential and Log Functions
Related Topics:
More Lessons for Intermediate Algebra
Math Worksheets
A series of free, online Intermediate Algebra Lessons or Algebra II lessons.
Videos, worksheets, and activities to help Algebra students.
In this lesson, we will learn
- how to calculate compound interest (finite)
- how to calculate compound interest (continuous)
- how to solve exponential growth or decay word problems
The following tables give the Formulas for Simple Interest, Compound Interest, and Continuously Compounded Interest. Scroll down the page for more examples and solutions.
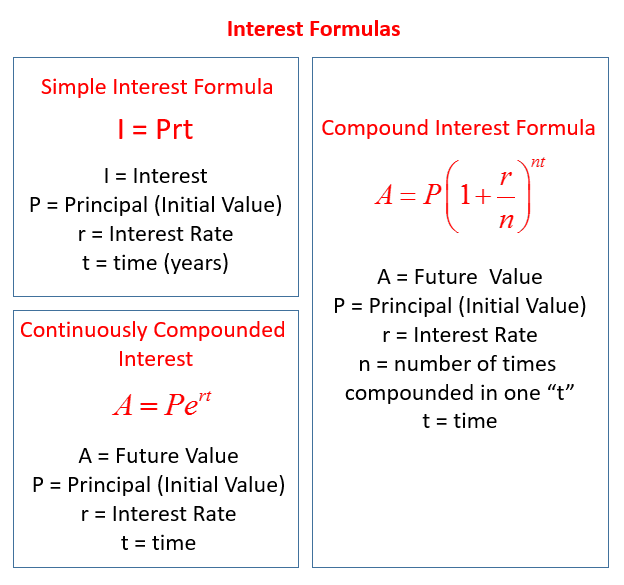
Compound Interest (Finite Number of Calculations)
One real world application of exponential equations is in compound interest. The formula for compound interest with a finite number of calculations is an exponential equation. We can solve for a parameter of this equation, and can use logarithms to access parameters in the exponent. Students may be asked to solve compound interest problems with interest compounded biannually, monthly, or daily.
Examples:
- Suppose that $1000 is invested at 8% compounded quarterly. How much is the account at the end of 3 years?
- Suppose that $1000 is invested at 8% compounded daily. How much is the account at the end of 3 years? Compare this with the previous example.
How to find the future value of an account that earns interest compounded monthly and continuously?
Examples:
- What is the accumulated balance for the following scenario? A $2000 deposit in an account at an APR of 7% with monthly compounding for 15 years.
- Determine the accumulated balance after 5 years for the following scenario:
A $3000 deposit in an account with an APR of 4% compounded continually.
Compound Interest (Continuously)
Problems that involve continuous compound interest use a different equation from problems that have finitely compounded interest, but the continuous compound interest equation is also an exponential equation. We use many of the same methods for calculating continuous compound interest as we do finitely compounded interest. To calculate compound interest, we can use logarithms and methods for solving exponential equations.
Continuously Compounded Interest
Example:
To get $1000 after 1 year at 12% compounded continuously.
Exponential Growth and Decay
Exponential decay refers to an amount of substance decreasing exponentially. Exponential decay is a type of exponential function where instead of having a variable in the base of the function, it is in the exponent. Exponential decay and exponential growth are used in carbon dating and other real-life applications.
Learn how to solve an exponential growth or decay word problem
This video shows how to solve the following word problem:
A population is growing exponentially; there were 200 bacteria 3 days ago and 1000 bacteria yesterday. How many bacteria will be present tomorrow?
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.