Arithmetic Sequences

Solutions, examples, videos, worksheets, and activities to help Algebra II students learn about arithmetic sequences.

The following figure gives the formula to find the nth term of an arithmetic sequence. Scroll down the page for more examples and solutions.

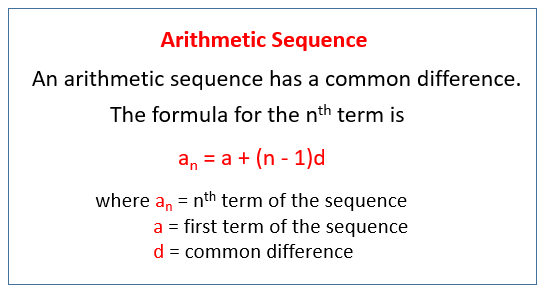
Arithmetic Sequences
A list of numbers that follows a rule is called a sequence. Sequences whose rule is the addition of a constant are called arithmetic sequences, similar to geometric sequences that follow a rule of multiplication. Homework problems on arithmetic sequences often ask us to find the nth term of a sequence using a formula. Arithmetic sequences are important to understanding arithmetic series.
How to find the general term of an arithmetic sequence?

• Show Step-by-step Solutions

Introduction to arithmetic sequences
Determine the nth term of an arithmetic sequence.
Determine the common difference of an arithmetic sequence.
Determine the formula for an arithmetic sequence.
An arithmetic sequence is a sequence that has the pattern of adding a constant to determine consecutive terms. We say arithmetic sequences have a common difference.
Examples:

1. A sequence is a function. What is the domain and range of the following sequence?
   9,6,3,0,-3,-6
   
2. Given the formula for the arithmetic sequence, determine the first 3 terms and then the 8^th term. Also state the common difference.
   a_n = -4n + 3
   
3. Given the arithmetic sequence, determine the formula and the 12th term.
   -2,1.5,5,8.5,12,15.5, …
   

• Show Step-by-step Solutions

Quick Introduction to Arithmetic Sequences
What an arithmetic sequence is with a few examples.
An arithmetic sequence is a sequence where succeeding terms in the sequence differ by a constant amount.
Example:
Determine which of the following sequences are arithmetic. If they are arithmetic, give the value of ’d'.
3,8,13,18,23,28,33, …
-.7, -1.7, -2.7, -3.7, -4.7, …
1.6,2.2,2.8,3.3,3.9,4.5, …
4/3,5/3,2,7/3,8/3,3, …

• Show Step-by-step Solutions

Arithmetic Sequences and Partial Sums
An arithmetic sequence may be thought of as a linear function whose domain is the set of natural numbers.
a_n = dn + c
The y-intercept is not the first term of the sequence.
c = a₁ - d
a₀ = dn + a₁ - d
Examples:

1. Find a formula for the nth term of the arithmetic sequence whose common difference is 3 and whose first term is 2.
   
2. The fourth term of an arithmetic sequence is 20, and the 13th term is 65. Write the first 6 terms of the sequence.
   
3. Find the 9th term of the arithmetic sequence that begins with 2 and 9.
   
4. Find the sum of the first 9 natural numbers.
   
5. Fin the sum of the finite arithmetic sequence
   1+3+5+7+9+11+13+15+17+19
   
6. Find the sum of integers from
   a) 1 to 100
   b) 1 to N
   
7. Find the sum of the first 150 terms of the sequence 5,16,27,38,49, …
   

• Show Step-by-step Solutions

Arithmetic Sequence
Example:
Find the common difference and the term named in the problem in the following arithmetic sequence.
-16, -18, -20, -22, …

• Show Step-by-step Solutions

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.