Average Speed Problems
Related Pages
Rate, Time, Distance
Solving Speed, Time, Distance Problems Using Algebra
More Algebra Lessons
In these lessons, we will learn how to solve word problems involving average speed.
There are three main types of average problems commonly encountered in school algebra:
Average (Arithmetic Mean)
Weighted Average and
Average Speed.
How to calculate Average Speed?
Let’s break down how to solve distance problems where you need to find the average speed. The crucial point is that average speed is not simply the average of the individual speeds. It’s calculated using the total distance traveled and the total time taken.
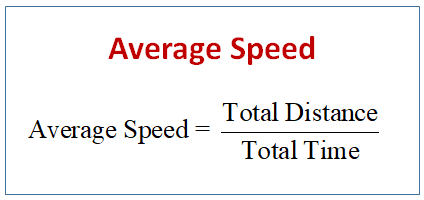
Formula: Average Speed = Total Distance / Total Time
The following diagram shows the formula for average speed. Scroll down the page for more examples and solutions on calculating the average speed.
Here’s a step-by-step approach:
- Find the distance for each part of the journey (if there are multiple parts). Use the formula Distance = Speed × Time for each segment.
- Find the time for each part of the journey (if it’s not already given). Use the formula Time = Distance / Speed.
- Calculate the total distance. Add up the distances from each part of the journey.
- Calculate the total time. Add up the times from each part of the journey.
- Divide total distance by total time. This gives you the average speed.
The following diagram gives the steps to solve a round-trip distance problem.

Types of Distance Word Problems:
Travel in Same Direction
Travel in Opposite Directions
Round Trip Problems
Average Speed Problems
Wind/Current Problems
Printable & Online Algebra Worksheets
Examples Of Average Speed Problems
Example:
John drove for 3 hours at a rate of 50 miles per hour and for 2 hours at 60 miles per hour.
What was his average speed for the whole journey?
Solution:
Step 1: The formula for distance is
Distance = Rate × Time
Total distance = 50 × 3 + 60 × 2 = 270
Step 2: Total time = 3 + 2 = 5
Step 3: Using the formula:
Answer: The average speed is 54 miles per hour.
Be careful! You will get the wrong answer if you add the two speeds and divide the answer by two.
How To Solve The Average Speed Problem?
How to calculate the average speed?
Example:
The speed paradox: If I drive from Oxford to Cambridge at 40 miles per hour and then from
Cambridge to Oxford at 60 miles per hour, what is my average speed for the whole journey?
How To Find The Average Speed For A Round Trip?
Example:
On Alberto’s drive to his aunt’s house, the traffic was light, and he drove the 45-mile trip
in one hour. However, the return trip took his two hours. What was his average trip for the
round trip?
How To Find The Average Speed Of An Airplane With Good And Bad Weather?
Example:
Mae took a non-stop flight to visit her grandmother. The 750-mile trip took three hours and
45 minutes. Because of the bad weather, the return trip took four hours and 45 minutes.
What was her average speed for the round trip?
How To Relate Speed To Distance And Time?
If you are traveling in a car that travels 80km along a road in one hour, we say that you are traveling at an average of 80kn/h.
Average speed is the total distance divided by the total time for the trip. Therefore, speed is distance divided by time.
Instantaneous speed is the speed at which an object is traveling at any particular instant.
If the instantaneous speed of a car remains the same over a period of time, then we say that the car is traveling with constant speed.
The average speed of an object is the same as its instantaneous speed if that object is traveling at a constant speed.
How To Calculate Average Speed In Word Problems?
Example:
Keri rollerblades to school, a total distance of 4.5km. She has to slow down twice to cross
busy streets, but overall the journey takes her 0.65h. What is Keri’s average speed during
the trip?
How To Use Average Speed To Calculate The Distance Traveled?
Example:
Elle drives 169 miles from Sheffield to London. Her average speed is 65 mph. She leaves
Sheffield at 6:30 a.m. Does she arrive in London by 9:00 a.m.?
How To Use Average Speed To Calculate The Time Taken?
Example:
Marie Ann is trying to predict the time required to ride her bike to the nearby beach. She
knows that the distance is 45 km and, from other trips, that she can usually average about
20 km/h. Predict how long the trip will take.
Check out many other Algebra Word Problems
Age Word Problems, Average Word Problems, Coin Word Problems, Consecutive Integer Word Problems, Digit Word Problems, Distance Word Problems, Fraction Word Problems, Geometry Word Problems, Integer Word Problems, Interest Word Problems, Lever Word Problems, Mixture Word Problems, Money Word Problems, Motion & Distance Word Problems, Number Sequence Word Problems, Proportion Word Problems, Quadratic Equation Word Problems, Ratio Word Problems, Symbol Word Problems, Variation Word Problems, Work Word Problems.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.