Illustrative Mathematics Unit 6.1, Lesson 5: Bases and Heights of Parallelograms
Learn how to find the area of a parallelogram using its base and height. After trying the questions, click on the buttons to view answers and explanations in text or video.
Return to the list of Illustrative Math lessons
Bases and Heights of Parallelograms
Let’s investigate the area of parallelograms some more.
Illustrative Math Unit 6.1, Lesson 5 (printable worksheets)
5.1 - A Parallelogram and Its Rectangles
Elena and Tyler were finding the area of this parallelogram:
Open Tyler’s solution and Elena’s solution. Drag the sliders to see the animation.
How are the two strategies for finding the area of a parallelogram the same? How they are different?
5.2 - The Right Height
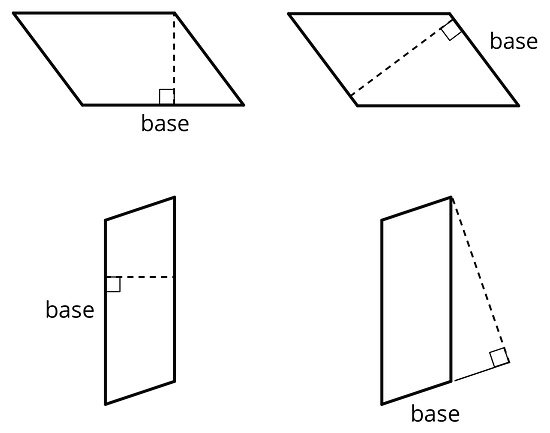
Study the following examples and non-examples of bases and heights of parallelograms.
Examples: The dashed segment in each drawing represents the corresponding height for the given base.
Non-examples: The dashed segment in each drawing does not represent the corresponding height for the given base.
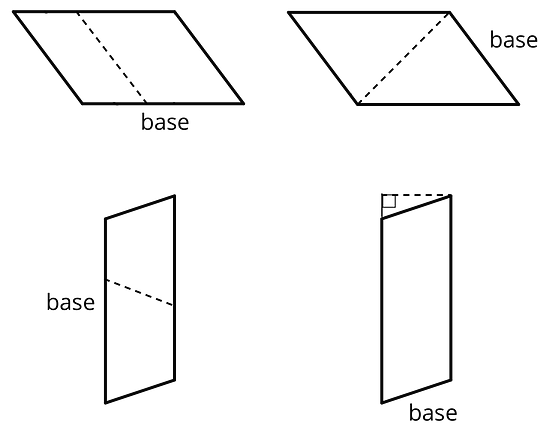
Select all statements that are true about bases and heights in a parallelogram.
A. Only a horizontal side of a parallelogram can be a base.
B. Any side of a parallelogram can be a base.
C. A height can be drawn at any angle to the side chosen as the base.
D. A base and its corresponding height must be perpendicular to each other.
E. A height can only be drawn inside a parallelogram.
F. A height can be drawn outside of a parallelogram, as long as it is drawn at a 90-degree angle to the base.
G. A base cannot be extended to meet a height.
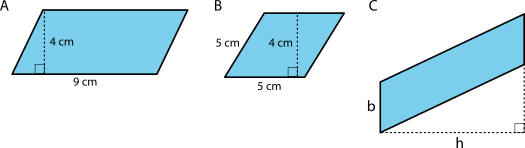
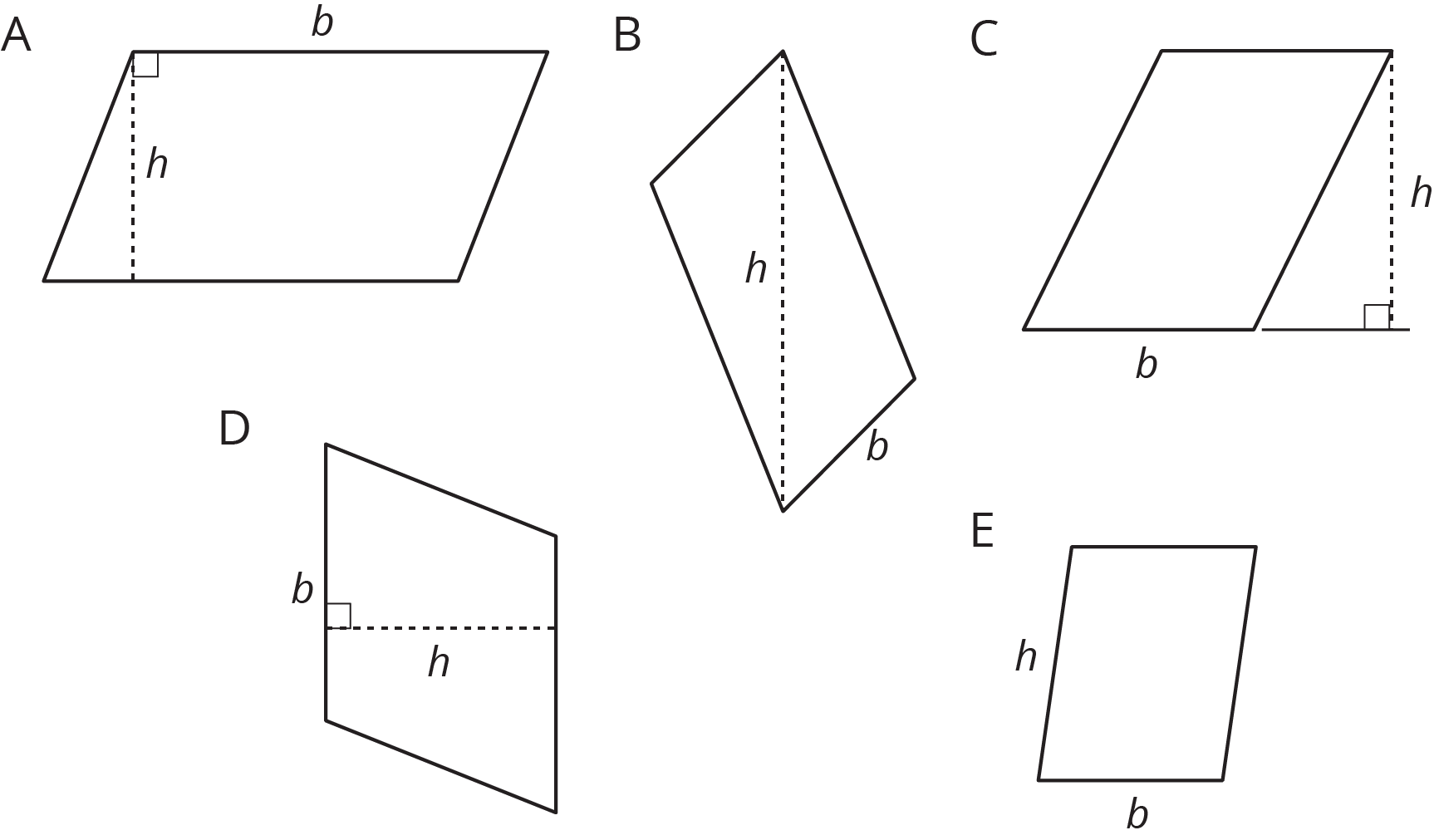
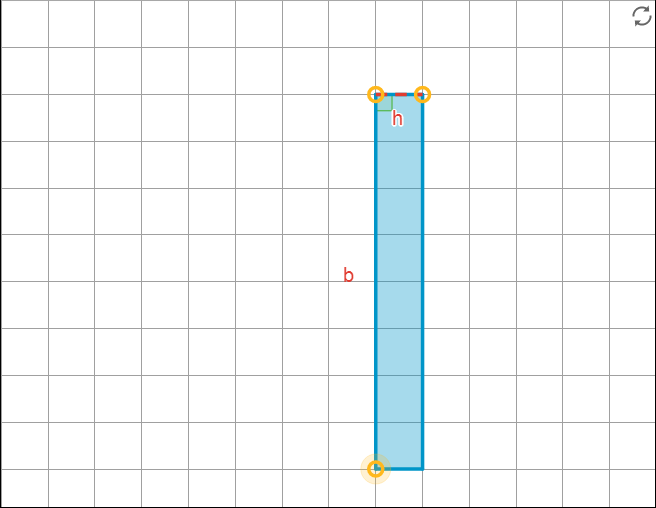
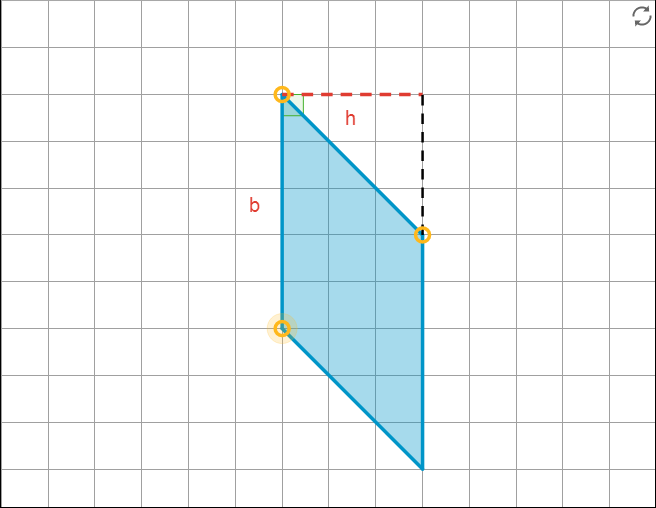
Five students labeled a base b and a corresponding height h for each of these parallelograms. Are all drawings correctly labeled? Explain how you know.
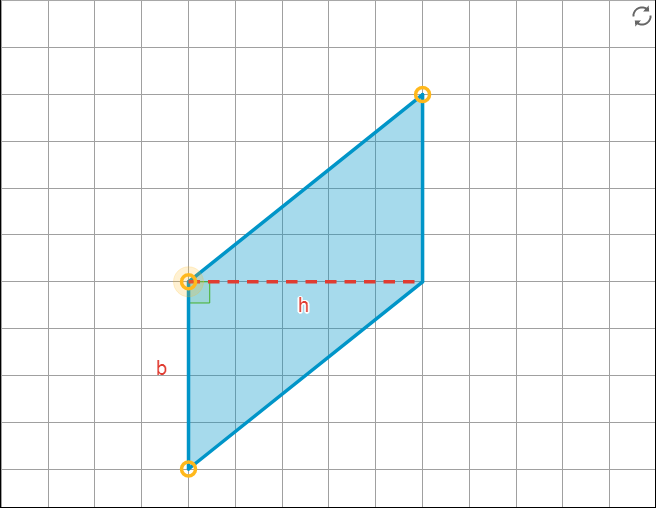
Open the applet. Experiment with dragging all of the movable points around the screen. Can you change the parallelogram so that:
- its height is in a different location?
- it has horizontal sides?
- it is tall and skinny?
- it is also a rectangle?
- it is not a rectangle, and has b = 5 and h = 3?
5.3 - Finding the Formula for Area of Parallelograms
For each parallelogram:
- Identify a base and a corresponding height, and record their lengths in the table that follows.
- Find the area and record it in the right-most column.
In the last row of the table, write an expression using b and h for the area of any parallelogram.
- What happens to the area of a parallelogram if the height doubles but the base is unchanged? If the height triples? If the height is 100 times the original?
- What happens to the area if both the base and the height double? Both triple? Both are 100 times their original lengths?
Lesson 5 Summary
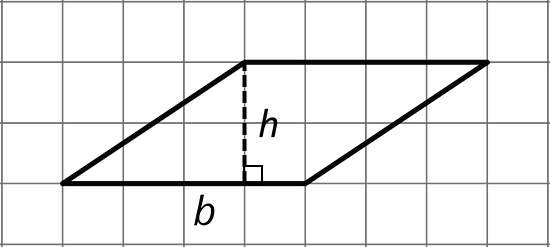
- We can choose any of the four sides of a parallelogram as the base. Both the side (the segment) and its length (the measurement) are called the base.
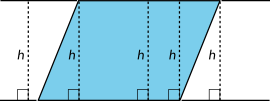
- If we draw any perpendicular segment from a point on the base to the opposite side of the parallelogram, that segment will always have the same length. We call that value the height. There are infinitely many line segments that can represent the height!
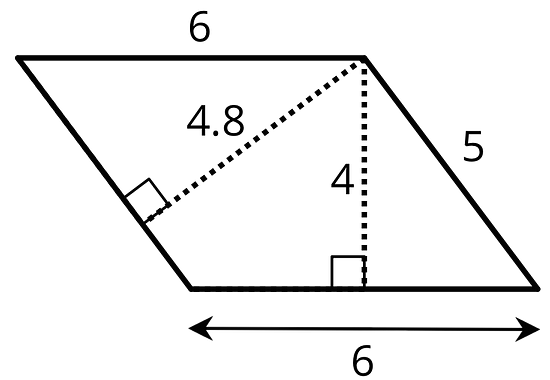
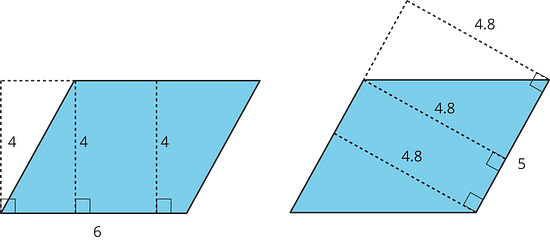
Here are two copies of the same parallelogram. On the left, the side that is the base is 6 units long. Its corresponding height is 4 units. On the right, the side that is the base is 5 units long. Its height is 4.8 units. For both, three different segments are shown to represent the height. We could draw in many more!
No matter which side is chosen as the base, the area of the parallelogram is the product of that base and its corresponding height. We can check it:
4 × 6 = 24 4.8 × 5 = 24
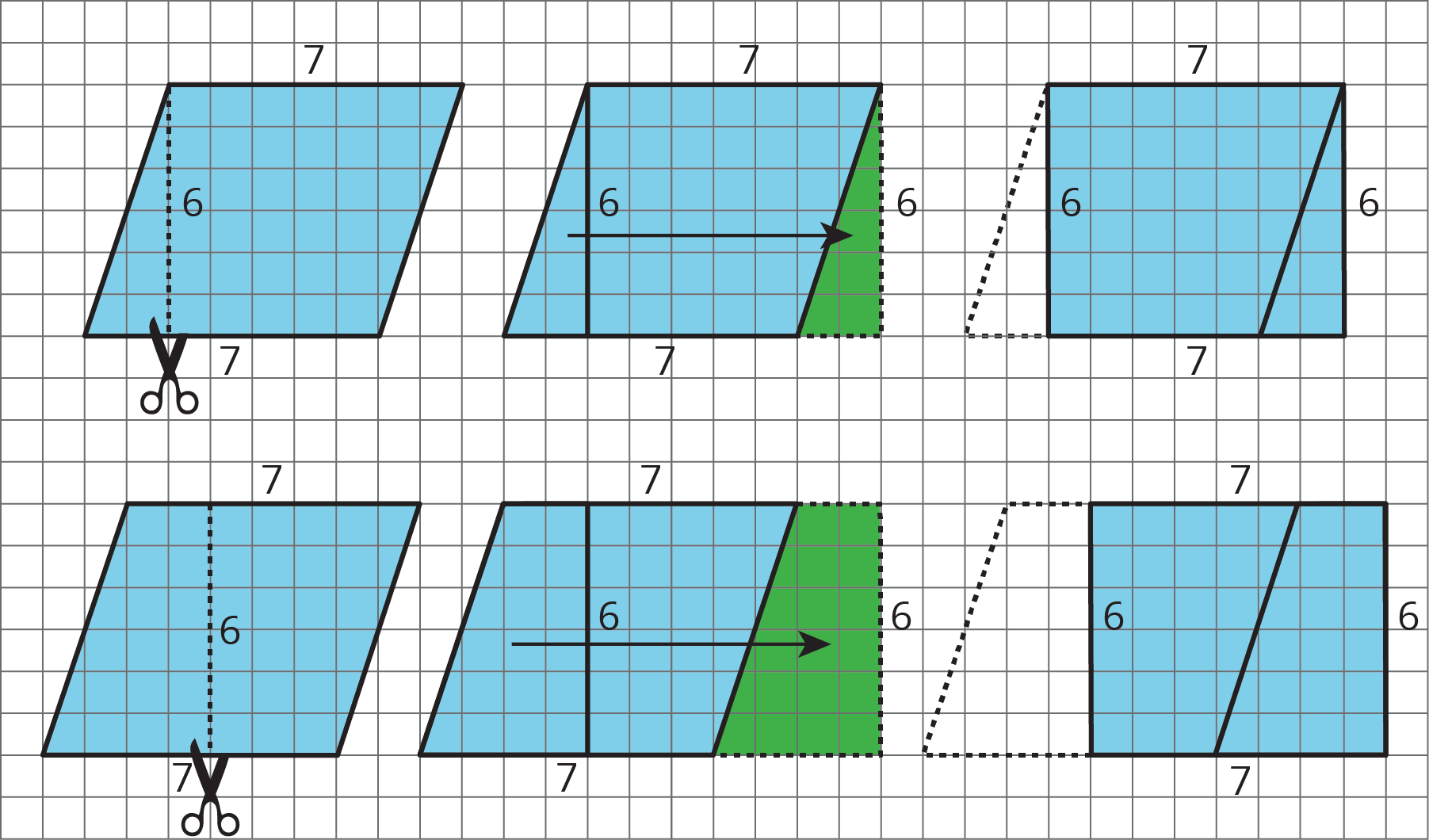
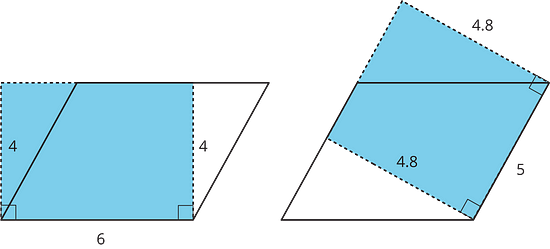
We can see why this is true by decomposing and rearranging the parallelograms into rectangles.
Notice that the side lengths of each rectangle are the base and height of the parallelogram. Even though the two rectangles have different side lengths, the products of the side lengths are equal, so they have the same area! And both rectangles have the same area as the parallelogram.
We can use letters to stand for numbers. If b is base of a parallelogram (in units), and h is the corresponding height (in units), then the area of the parallelogram (in square units) is the product of these two numbers b·h.
Notice that the multiplication symbol can be written with a small dot instead of a × symbol. This is so that we don’t get confused about whether × means multiply, or whether the letter x is standing in for a number.
In high school, you will be able to prove that a perpendicular segment from a point on one side of a parallelogram to the opposite side will always have the same length. You can see this most easily when you draw a parallelogram on graph paper or look at the diagram below. For now, we will just use this as a fact.
Practice Problems
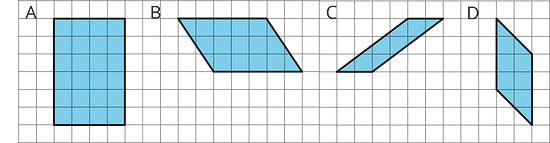
- Select all parallelograms that have a correct height labeled for the given base.
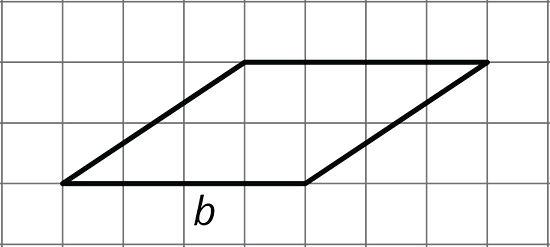
- The side labeled b has been chosen as the base for this parallelogram. Draw a segment showing the height corresponding to that base.
- Find the area of each parallelogram.
- If the side that is 6 units long is the base of this parallelogram, what is its corresponding height?
A: 6 units
B: 4.8 units
C: 4 units
D: 5 units
- Find the area of each parallelogram. Write out equations.
- Do you agree with each of these statements? Explain your reasoning.
A: A parallelogram has six sides.
B: Opposite sides of a parallelogram are parallel.
C: A parallelogram can have one pair or two pairs of parallel sides.
D: All sides of a parallelogram have the same length.
E: All angles of a parallelogram have the same measure.
- A square with an area of 1 square meter is decomposed into 9 identical small squares. Each small square is decomposed into two identical triangles.
A: What is the area, in square meters, of 6 triangles? If you get stuck, draw a diagram.
B: How many triangles are needed to compose a region that is 1½ square meters?
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.
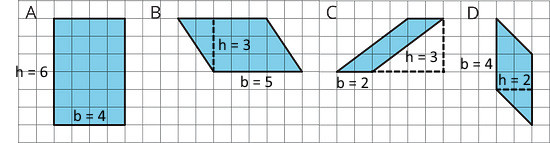

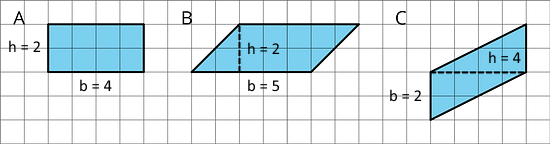 A: 4 × 2 = 8 square units
A: 4 × 2 = 8 square units