Binomial Distribution
Related Pages
Binomial Distribution Tutorial
Binomial Distribution: Critical Values
More Lessons for Statistics
Math Worksheets
These lessons, with videos, examples and step-by-step solutions, help Statistics students learn how to use the binomial distribution. The binomial distribution is a discrete probability distribution that describes the probability of obtaining a certain number of successes in a sequence of independent trials, each of which has only two possible outcomes: success or failure.
Perhaps the most widely known of all discrete distribution is the binomial distribution. The binomial distribution has been used for hundreds of years. Several assumptions underlie the use of the binomial distribution.
Assumptions of the binomial distribution:
- The experiment involves n identical trials.
- Each trial has only two possible outcomes denoted as success or failure.
- Each trial is independent of the previous trials.
- The terms p and q remain constant throughout the experiment, where p is the probability of getting a success on any one trial and q = (1 – p) is the probability of getting a failure on any one trial.
The following diagram gives the Binomial Distribution Formula. Scroll down the page for more examples and solutions.
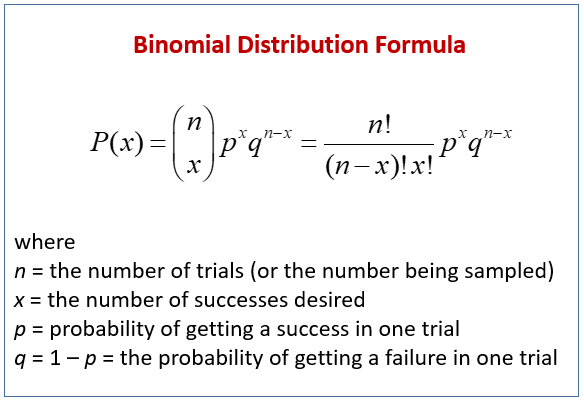
The binomial distribution is characterized by two parameters:
- n (number of trials): A positive integer representing the total number of independent trials.
- p (probability of success): A real number between 0 and 1 (inclusive) representing the probability of success on each trial.
Introduction to the binomial distribution
More Binomial Distribution
Basketball binomial distribution
Using Excel to visualize the basketball binomial distribution
Binomial Experiment
The following video will discuss what a binomial experiment is, discuss the formula for finding the probability associated with a binomial experiment, and gives an example to illustrate the concepts.
Example:
Suppose you take a multiple choice test with 10 questions, and each question has 5 answer choices (a, b, c, d, e), what is the probability you get exactly 4 quaetions correct just by guessing?
In summary, the binomial distribution is a fundamental tool in probability and statistics for analyzing situations where there are a fixed number of independent trials with only two possible outcomes and a constant probability of success. It allows us to calculate the likelihood of different numbers of successes occurring in such scenarios.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.