Circle Theorems
In these lessons, we will learn
- inscribed angles
- a circle theorem about inscribed angles which is sometimes called the Bow Theorem. It states that the inscribed angles subtended by the same arc or chord are equal.
Inscribed Angles
We will first look at what is meant by inscribed angle or angle at the circumference.
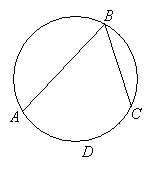
An inscribed angle has its vertex on the circle. ∠ABC, in the diagram below, is called an inscribed angle. The angle is also said to be subtended by (i.e. opposite to) arc ADC or chord AC.
∠ABC is also called an angle at the circumference.
Bow Theorem
Now we will look at the Bow Theorem.
The theorem states that:
The inscribed angles subtended by the same arc or chord are equal.
Arcs that contain equal angles are equal.
Angles in the same segment are equal.
The angles at the circumference subtended by the same arc are equal.

∠x = ∠y because they are subtended by the same arc AEC.
You can see from the above diagram that the inscribed angles form a “bow-tie”. That is why it is sometimes called the bow theorem.
Example:
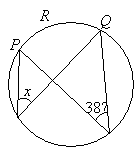
Find the value of ∠x in the figure below.
Solution:
∠x = 38˚ because they are both subtended by the same arc PRQ.
What is the inscribed angle property and how it can be used to find missing angles
(1) Inscribed angles from equal arcs are equal.
(2) Arcs that contain equal angles are equal.
Two useful properties when solving problems that involve inscribed angles
(1) The measure of the inscribed angle is half the measure of the central angle.
(2) Inscribed angles that intercept congruent arcs are congruent.
Inscribed Angles and Quadrilaterals and Arcs
What is the relationship between an inscribed angle and its intercepted arc?
The angle is half the arc (or the arc is twice the angle). Also, if a quadrilateral is inscribed in a circle, opposite angle are supplementary.
How theorems about inscribed angles can be used to solve a problem in high school geometry?
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.