Geometry: Circles
These lessons cover the various properties of circles, the parts of a circle and the terms commonly associated with circles.
Related Pages
Angles In A Circle
Area Of A Circle
Conic Sections: Circles
We shall learn about circles and the properties of circles.
- diameter,
- chord,
- radius,
- arc,
- semicircle, minor arc, major arc,
- tangent,
- secant,
- circumference and formula,
- area and formula,
- sector and formula.
The following figures show the different parts of a circle: tangent, chord, radius, diameter, arc, segment, sector. Scroll down the page for more examples and explanations.
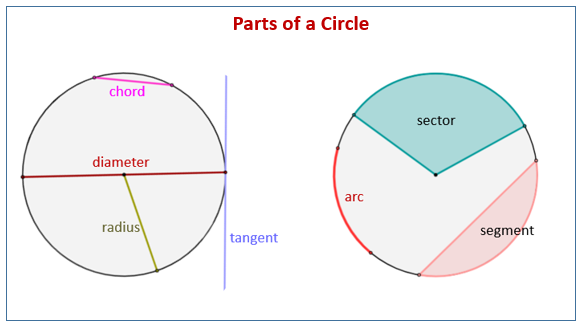
Circle
In geometry, a circle is a closed curve formed by a set of points on a planeplane that are the same distance from its center O. That distance is known as the radius of the circle.
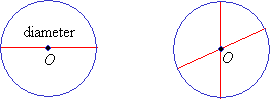
Diameter
The diameter of a circle is a line segment that passes through the center of the circle and has its endpoints on the circle. All the diameters of the same circle have the same length.
Chord
A chord is a line segment with both endpoints on the circle. The diameter is a special chord that passes through the center of the circle. The diameter would be the longest chord in the circle.
Radius
The radius of the circle is a line segment from the center of the circle to a point on the circle.
In the above diagram, O is the center of the circle and 
and 
are radii of the circle. The radii of a circle are all the same length. The radius is half the
length of the diameter. 
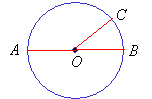
Arc
An arc is a part of a circle.
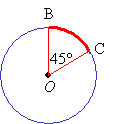
In the diagram above, the part of the circle from B to C forms an arc. It is called arc BC.
An arc can be measured in degrees. In the circle above, the measure of arc BC is equal to its central angle ∠BOC, which is 45°.
Semicircle, Minor Arc and Major Arc
A semicircle is an arc that is half a circle. A minor arc is an arc that is smaller than a semicircle. A major arc is an arc that is larger than a semicircle.
How To Identify Semicircle, Minor Arc And Major Arc?
Tangent
A tangent to a circle is a line that touches a circle at only one point. A tangent is perpendicular to the radius at the point of contact.
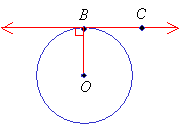
In the above diagram, the line containing the points B and C is a tangent to the circle.
It touches the circle at point B and is perpendicular to the radius
is perpendicular to i.e.
Secant
A secant is a straight line that cuts the circle at two points. A chord is the portion of a secant that lies in the circle.
Circumference
The circumference of a circle is the distance around a circle.
Calculating the circumference of a circle involves a constant called pi with the symbol π. The value of π (pi) is approximately 3.14159265358979323846… but usually rounding to 3.142 should be sufficient. (see a mnemonic for π)
The formula for the circumference of a circle is
C = πd (see a mnemonic for this formula)
or
C = 2πr
where C is the circumference, d is the diameter and r is the radius.
If you are given the diameter then use the formula C = πd
If you are given the radius then use the formula C = 2πr
Circumference Worksheet to calculate the circumference of a circle.
Area & Circumference Worksheet to calculate the area and circumference of a circle.
Example 1: Find the circumference of the circle with a diameter of 8 inches.
Solution:
| Step 1: Write down the formula: | C = πd |
| Step 2: Plug in the value: | C = 8π |
Answer: The circumference of the circle is 8π ≈ 25.163 inches.
Example 2: Find the circumference of the circle with a radius of 5 inches.
Solution:
| Step 1: Write down the formula: | C = 2πr |
| Step 2: Plug in the value: | C = 10π |
Answer: The circumference of the circle is 10π ≈ 31.42 inches.
Explore The Relationship Between The Radius, Diameter And Circumference Of A Circle
Area
The area of a circle is the region enclosed by the circle. It is given by the formula:
A = πr2 (see a mnemonic for this formula)
where A is the area and r is the radius.
Since the formula is only given in terms of radius, remember to change from diameter to radius if necessary.
Circles Worksheet to calculate the area of a circle.
Area and Circumference Worksheet
to calculate the area and circumference of a circle.
Circle Problems Worksheet to calculate problems that involve the radius, diameter, circumference and area of circle.
Example 1: Find the area the circle with a diameter of 10 inches.
Solution:
| Step 1: Write down the formula: | A = πr2 |
| Step 2: Change diameter to radius: |  |
| Step 3: Plug in the value: | A = π52 = 25π |
Answer: The area of the circle is 25π ≈ 78.55 square inches.
Example 2: Find the area the circle with a radius of 10 inches.
Solution:
| Step 1: Write down the formula: | A = πr2 |
| Step 2: Plug in the value: | A = π102 = 100π |
Answer: The area of the circle is 100π ≈ 314.2 square inches.
How To Relate The Radius And Diameter Of A Circle With Its Area?
Sector
A sector is like a "pizza slice" of the circle. It consists of a region bounded by two radii and an arc lying between the radii. The area of a sector is a fraction of the area of the circle.
The formula to calculate the area of a sector is
How To Derive The Formula To Calculate The Area Of A Sector In A Circle?
The following video lesson explains how to find the area of a sector.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.
We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.