Complex Plane: Rectangular and Polar Form
Related Topics:
Common Core (The Complex Number System)
Common Core for Mathematics
Examples, solutions, and lessons to help High School students learn how to represent complex numbers on the complex plane in rectangular and polar form (including real and imaginary numbers), and explain why the rectangular and polar forms of a given complex number represent the same number.
Suggested Learning Targets
- I can represent a complex number in a complex plane.
- I can convert a complex number from rectangular (standard) form to polar (trigonometric) form.
- I can convert a complex number from polar form to rectangular form.
Common Core: HSN-CN.B.4
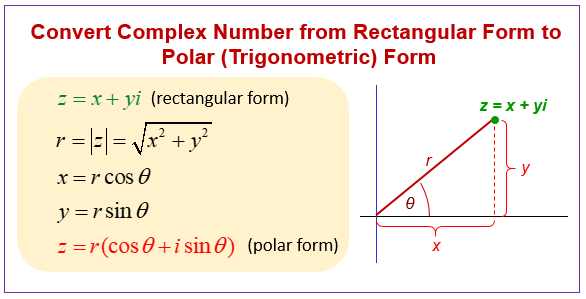
The following diagram shows how to convert complex numbers from rectangular form to polar (trigonometric) form. Scroll down the page for more examples and solutions.
The Complex Plane
Complex Numbers - The Complex Plane
Represent a complex number in the complex plane.
The complex plane, complex numbers in standard form, the difference between complex numbers and imaginary numbers, and plotting complex numbers in the complex plane.
The Polar Form of Complex Numbers
The complex plane; converting complex numbers between rectangular form and polar (trigonometric) form; the product and quotient formulas for complex numbers written in polar form.
From Rectangular to Polar Form
Expressing a Complex Number in Trigonometric or Polar Form, Ex 1.
Expressing a Complex Number in Trigonometric or Polar Form, Ex 2
Expressing a Complex Number in Trigonometric or Polar Form, Ex 3
From Polar to Rectangular Form
Complex Number in Rectangular Form
Step by step instruction in converting a complex number from its polar form to its rectangular equivalent.
Complex Numbers: Convert From Polar to Complex Form (Rectangular Form), Ex 1
We take a number in polar form and write it in complex form.
The Complex Plane and Polar Form
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.