Are All Parabolas Congruent?

Are All Parabolas Congruent?

Student Outcomes

• Students learn the vertex form of the equation of a parabola and how is arises from the definition of a parabola.
• Students perform geometric operations, such as rotations, reflections, and translations, on arbitrary parabolas to discover standard representations for their congruence classes. In doing so, they learn that all parabolas with the same distance p between the focus and the directrix are congruent to the graph of y = (1/2p)x².

New York State Common Core Math Algebra II, Module 1, Lesson 34

Worksheets for Algebra 2

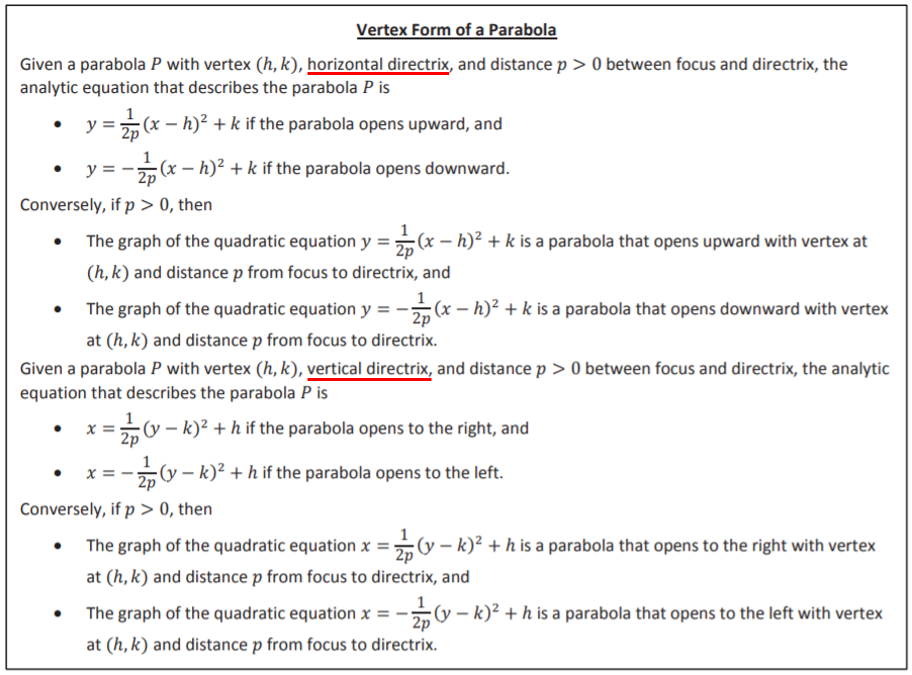
The following diagram shows the vertex form of a parabola. Scroll down the page for more examples and explanations about the vertex form of the equation of a parabola.
 

Classwork

Opening Exercise

Are all parabolas congruent? Use the following questions to support your answer.
a. Draw the parabola for each focus and directrix given below.
b. What do we mean by congruent parabolas?
c. Are the two parabolas from part (a) congruent? Explain how you know.
d. Are all parabolas congruent?
e. Under what conditions might two parabolas be congruent? Explain your reasoning

• Show Video for the Lesson

Exercises 1–5

1. Draw the parabola with the given focus and directrix.
2. Draw the parabola with the given focus and directrix.
3. What can you conclude about the relationship between the parabolas in Exercises 1–3?
4. Let 𝑝 be the number of units between the focus and the directrix, as shown. As the value of 𝑝 increases, what happens to the shape of the resulting parabola?

Example 1: Derive an Equation for a Parabola
Consider a parabola 𝑃 with distance 𝑝 > 0 between the focus with coordinates (0,1/2 𝑝), and directrix 𝑦 = −1/2 𝑝.
What is the equation that represents this parabola?

Example 2
THEOREM: Given a parabola 𝑃 given by a directrix 𝐿 and a focus 𝐹 in the Cartesian plane, then 𝑃 is congruent to the graph of 𝑦 = 1/2𝑝 𝑥², where 𝑝 is the distance from 𝐹 to 𝐿.
PROOF

Exercises 6–9: Reflecting on the Theorem
6. Restate the results of the theorem from Example 2 in your own words.
7. Create the equation for a parabola that is congruent to 𝑦 = 2𝑥². Explain how you determined your answer.
8. Create an equation for a parabola that IS NOT congruent to 𝑦 = 2𝑥². Explain how you determined your answer.
9. Write the equation for two different parabolas that are congruent to the parabola with focus point (0,3) and directrix line 𝑦 = −3.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.