Construct an Equilateral Triangle

New York State Common Core Math Geometry, Module 1, Lesson 1

Worksheets for Geometry

Student Outcomes

• Students learn to construct an equilateral triangle.
• Students communicate mathematic ideas effectively and efficiently.

Construct an Equilateral Triangle

Classwork

Opening Exercise

Joe and Marty are in the park playing catch. Tony joins them, and the boys want to stand so that the distance between any two of them is the same. Where do they stand?
How do they figure this out precisely? What tool or tools could they use?

Fill in the blanks below as each term is discussed:
a. The _______ between points 𝐴 and 𝐵 is the set consisting of 𝐴, 𝐵, and all points on the line 𝐴𝐵 between 𝐴 and 𝐵.
b. A segment from the center of a circle to a point on the circle
c. Given a point 𝐶 in the plane and a number 𝑟 > 0, the _______ with center 𝐶 and radius 𝑟 is the set of all points in the plane that are distance 𝑟 from point 𝐶.
Note that because a circle is defined in terms of a distance, 𝑟, we often use a distance when naming the radius (e.g., “radius 𝐴𝐵”). However, we may also refer to the specific segment, as in “radius AB.”

• Show Video for Whole Lesson

Example 1: Sitting Cats

You need a compass and a straightedge.

Margie has three cats. She has heard that cats in a room position themselves at equal distances from one another and wants to test that theory. Margie notices that Simon, her tabby cat, is in the center of her bed (at S), while JoJo, her Siamese, is lying on her desk chair (at J). If the theory is true, where will she find Mack, her calico cat? Use the scale drawing of Margie’s room shown below, together with (only) a compass and straightedge. Place an M where Mack will be if the theory is true.

Mathematical Modeling Exercise: Euclid, Proposition 1

Let’s see how Euclid approached this problem. Look at his first proposition, and compare his steps with yours.

 

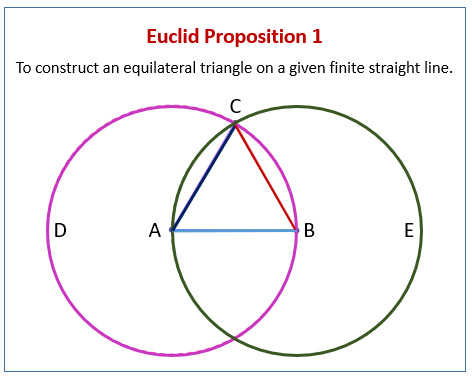
Proposition 1

To construct an equilateral triangle on a given finite straight line.

Let AB be the given finite straight line.

So, it is required to construct an equilateral triangle on the straight-line AB. Let the circle BCD with center A and radius AB have been drawn [Post. 3], and again let the circle ACE with center B and radius BA have been drawn [Post. 3]. And let the straight lines CA and CB have been joined from the point C, where the circles cut one another to the points A and B (respectively) [Post, 1].

And since the point A is the center of the circle CDB, AC is equal to AB [Def. 1.15]. Again, since the point B is the center of the circle CAE, BC is equal to BA[Def. 1.15]. But CA was also shown (to be) equal to AB. But things equal to the same thing are also equal to one another [C.N. 1]. Thus, CA is also equal to CB. Thus, the three (straight lines) CA, AB and BC are equal to one another. therefore each of the straight lines AC and BC equals AB.

Thus, the triangle ABC is equilateral, and it has been constructed on the given finite straight line AB. (Which is) the very thing that it was required to do.

Geometry Assumptions

In geometry, as in most fields, there are specific facts and definitions that we assume to be true. In any logical system, it helps to identify these assumptions as early as possible since the correctness of any proof hinges upon the truth of our assumptions. For example, in Proposition 1, when Euclid says, “Let 𝐴𝐵 be the given finite straight line,” he assumed that, given any two distinct points, there is exactly one line that contains them. Of course, that assumes we have two points! It is best if we assume there are points in the plane as well: Every plane contains at least three noncollinear points.

Euclid continued on to show that the measures of each of the three sides of his triangle are equal. It makes sense to discuss the measure of a segment in terms of distance. To every pair of points 𝐴 and 𝐵, there corresponds a real number dist(𝐴, 𝐵) ≥ 0, called the distance from 𝐴 to 𝐵. Since the distance from 𝐴 to 𝐵 is equal to the distance from 𝐵 to 𝐴, we can interchange 𝐴 and 𝐵: dist(𝐴, 𝐵) = dist(𝐵,𝐴). Also, 𝐴 and 𝐵 coincide if and only if dist(𝐴, 𝐵) = 0.

Using distance, we can also assume that every line has a coordinate system, which just means that we can think of any line in the plane as a number line. Here’s how: Given a line, 𝑙, pick a point 𝐴 on 𝑙 to be “0,” and find the two points 𝐵 and 𝐶 such that dist(𝐴, 𝐵) = dist(𝐴, 𝐶) = 1. Label one of these points to be 1 (say point 𝐵), which means the other point 𝐶 corresponds to −1. Every other point on the line then corresponds to a real number determined by the (positive or negative) distance between 0 and the point. In particular, if after placing a coordinate system on a line, if a point 𝑅 corresponds to the number 𝑟, and a point 𝑆 corresponds to the number 𝑠, then the distance from 𝑅 to 𝑆 is dist(𝑅, 𝑆) = |𝑟 −𝑠|.

History of Geometry: Examine the site http://geomhistory.com/home.html to see how geometry developed over time

Relevant Vocabulary

GEOMETRIC CONSTRUCTION: A geometric construction is a set of instructions for drawing points, lines, circles, and figures in the plane.

The two most basic types of instructions are the following:

1. Given any two points 𝐴 and 𝐵, a straightedge can be used to draw the line 𝐴𝐵 or segment 𝐴𝐵.
2. Given any two points 𝐶 and 𝐵, use a compass to draw the circle that has its center at 𝐶 that passes through 𝐵. (Abbreviation: Draw circle 𝐶: center 𝐶, radius 𝐶𝐵.)

Constructions also include steps in which the points where lines or circles intersect are selected and labeled. (Abbreviation: Mark the point of intersection of the line 𝐴𝐵 and line 𝑃𝑄 by 𝑋, etc.)

FIGURE: A (two-dimensional) figure is a set of points in a plane.

Usually the term figure refers to certain common shapes such as triangle, square, rectangle, etc. However, the definition is broad enough to include any set of points, so a triangle with a line segment sticking out of it is also a figure.

EQUILATERAL TRIANGLE: An equilateral triangle is a triangle with all sides of equal length.

COLLINEAR: Three or more points are collinear if there is a line containing all of the points; otherwise, the points are noncollinear.

LENGTH OF A SEGMENT: The length of AB is the distance from 𝐴 to 𝐵 and is denoted 𝐴𝐵. Thus, 𝐴𝐵 = dist(𝐴, 𝐵).

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.