Cross Sections and Solids of Rotation
Related Topics:
Common Core (Geometry)
Common Core for Mathematics
Videos, examples, solutions, and lessons to help High School students learn how to identify the shapes of two-dimensional cross-sections of three-dimensional objects, and identify three-dimensional objects generated by rotations of two-dimensional objects.
Examples:
What is the shape of the cross section of each of the following figures?
Cross Sections
Learn to identify cross sections of solids.
Examples:
Sketch and identify the cross section of each of the figure below.
Cross Sections & Rotations of 2D to 3D
A video that shows how you can convert Figures from 2D to 3D by cross sections and rotations. Solids of rotation (Solids in 3D)
Rotate a triangle to get a cone.
Rotate a rectangle to get a cylinder
Rotate a trapezium to get frustum
Rotate a circle (semi-circle) to get a sphere. Solids of rotation
A solid of rotation is the three-dimensional (solid) object formed by rotating a two-dimensional area around an axis. For animations to explore the shapes of two-dimensional cross-sections of three-dimensional objects and identify three-dimensional objects generated by rotations of two-dimensional objects, see:
Common Core (Geometry)
Common Core for Mathematics
Videos, examples, solutions, and lessons to help High School students learn how to identify the shapes of two-dimensional cross-sections of three-dimensional objects, and identify three-dimensional objects generated by rotations of two-dimensional objects.
Common Core: HSG-GMD.B.4
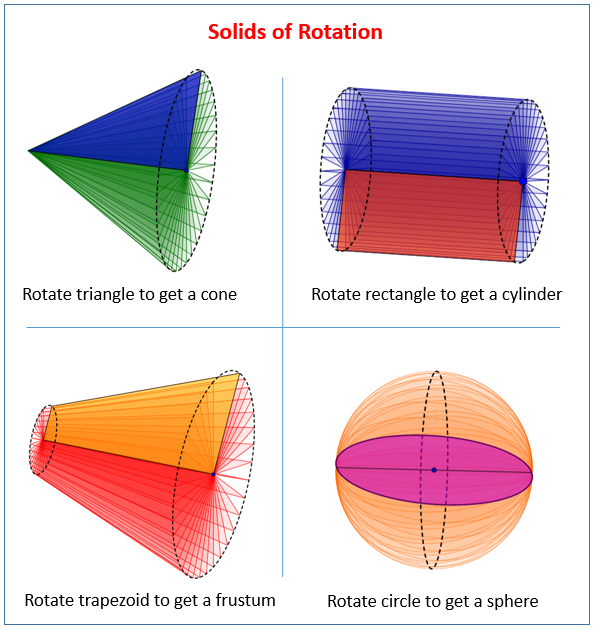
The following figures show some solids of rotation: cone, cylinder, frustum, sphere. Scroll down the page for more examples and solutions.
Examples:
What is the shape of the cross section of each of the following figures?
Cross Sections
Learn to identify cross sections of solids.
Examples:
Sketch and identify the cross section of each of the figure below.
A video that shows how you can convert Figures from 2D to 3D by cross sections and rotations. Solids of rotation (Solids in 3D)
Rotate a triangle to get a cone.
Rotate a rectangle to get a cylinder
Rotate a trapezium to get frustum
Rotate a circle (semi-circle) to get a sphere. Solids of rotation
A solid of rotation is the three-dimensional (solid) object formed by rotating a two-dimensional area around an axis. For animations to explore the shapes of two-dimensional cross-sections of three-dimensional objects and identify three-dimensional objects generated by rotations of two-dimensional objects, see:
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.
We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.