Decimals - Grade 5
More Lessons for Grade 5 Math
Grade 5 Worksheets
Decimal Worksheets
Decimal Games
In these lessons, we will learn some models for decimals, how to write decimals in expanded form, how to compare and order decimals, how to estimate and round decimals.
Model Decimals
1. Using decimals extends the place value system to represent parts of a whole.
The use of a decimal point is a symbol that separates the tenths from the ones, or the 'part from the whole'. For example, in the number 4.2, the decimal point separates the 4 ones and the 2 tenths.
2. The base ten place value system is built on symmetry around the ones place and the decimal.
3. Decimals can represent parts of a whole, as well as mixed numbers.
4. Decimals can be interpreted and read in more than one way. For example, 4.3 may be renamed 43 tenths.
5. Decimals can be renamed as other decimals or fractions. For example, 800/1000 or 0.800 can be renamed as 80/100 or 0.80. It can also be renamed as 8/10 or 0.8
Take note that
0.5 or 0.50 or 0.500 are all equal to 1/2
0.25 or 0.250 are both equal 1/4
0.75 and 0.750 are equal to 3/4
We can use a thousands grid to model decimals.
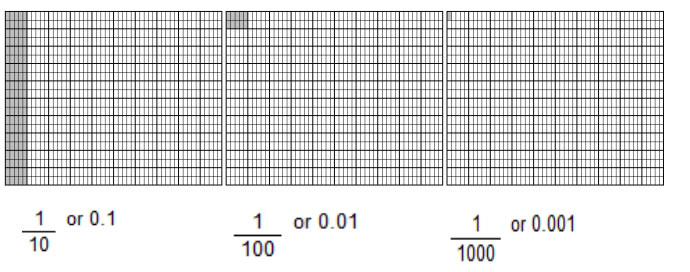
The following shaded grid can be used to represent the number 3.146
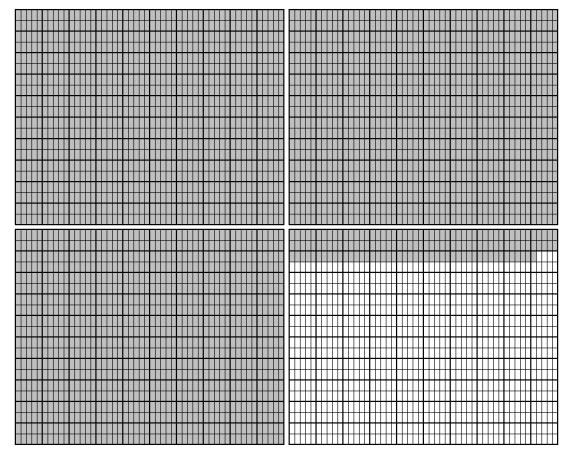
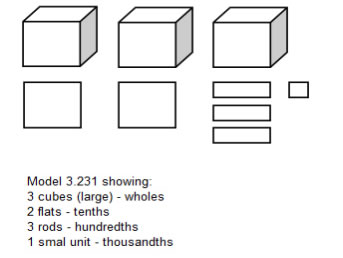
We can also use base ten blocks to model decimals.
The following can be used to represent the number 3.231
Meter sticks can also be used to represent decimals. Measuring to the nearest millimeter is one thousandths of a meter. Centimeters are hundredths of a meter and decimeters are tenths of a meter.
Model Decimals
Model decimals on grids and on number lines.
Using a model of a garden, students develop an understanding of tenths and hundredths places, as well as relate decimals to fractions and percents.
Decimals in Expanded Form
A decimal can be written in expanded form.For example:
45.23 = 40 + 5 + 0.2 + 0.03
or
45.23 = (4 × 10) + (5 × 1) + (2 × 0.1) + (3 × 0.01).
50.302 = 50 + 0.3 + 0.002
or
50.302 = (5 × 10) + (3 × 0.1) + (2 × 0.001).
Decimals - Expanded Form
Reading/writing decimals in numeric, word, and expanded form
Examples:
1. Write in expanded form:
a. 9.45
b. 0.21
c. 8.878
d. 0.3911
Compare Decimals
We can compare decimals using place value. For example:0.02 < 0.2 because 0 tenths is less than 2 tenths
0.021 > 0.01 because 2 hundredths is greater that 1 hundredths
We can compare decimals using a benchmark number. For example:
0.021 < 0.2 because 0.021 is less than 0.1 and 0.2 is greater than 0.1
0.8 > 0.423 because 0.8 is more than half and 0.423 is less than half.
We can compare using equivalent decimals with same number of digits. For example:
0.34 > 0.3 because 0.34 > 0.30 (34 hundredths > 30 hundredths)
8.302 < 8.32 because 8.302 < 8.320 (302 thousandths < 320 thousandths)
Ordering & Comparing Decimals.
Estimate and Round Decimals
We can round decimals to simpler decimals.For example, 2.9286
can be rounded to 2.929 (nearest thousandths)
can be rounded to 2.93 (nearest hundredths)
can be rounded to 2.9 (nearest tenths)
can be rounded to 3 (nearest ones)
Estimating and Rounding with Decimals
Rotate to landscape screen format on a mobile phone or small tablet to use the Mathway widget, a free math problem solver that answers your questions with step-by-step explanations.
You can use the free Mathway calculator and problem solver below to practice Algebra or other math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.
We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.