Dependent Events
Related Pages
Dependent Events
Independent Events
Tree Diagrams
More Lessons On Probability
Probability Worksheets
Theoretical And Experimental Probability
In these lessons, we will learn how to find the probability of dependent events. We will also learn the difference between the probability of dependent events and the probability of independent events.
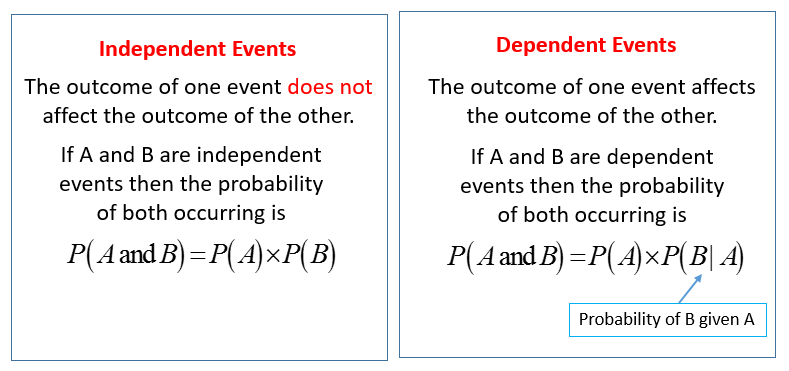
The following table gives the formulas for the probability of independent and dependent events. Scroll down the page for more examples and solutions.
Printable & Online Probability Worksheets
Events are dependent if the outcome of one event affects the outcome of another. For example, if you draw two colored balls from a bag and the first ball is not replaced before you draw the second ball then the outcome of the second draw will be affected by the outcome of the first draw.
If A and B are dependent events, then the probability of A happening AND the probability of B happening, given A, is P(A) × P(B after A).
P(A and B) = P(A) × P(B after A)
P(B after A) can also be written as P(B | A)
then P(A and B) = P(A) × P(B | A)
Example:
A purse contains four $5 bills, five $10 bills and three $20 bills. Two bills are selected
without the first selection being replaced. Find P($5, then $5).
Solution:
There are four $5 bills.
There are a total of twelve bills.
P($5) = 
The result of the first draw affected the probability of the second draw.
There are three $5 bills left.
There are a total of eleven bills left.
P($5 after $5) = 
P($5, then $5) = P($5) · P($5 after $5) = 
The probability of drawing a $5 bill and then a $5 bill is 
Example:
A bag contains 6 red, 5 blue and 4 yellow marbles. Two marbles are drawn, but the first marble
drawn is not replaced.
a) Find P(red, then blue)
b) Find P(blue, then blue)
Solution:
a) There are 6 red marbles.
There are a total of 15 marbles.
P(red) = 
The result of the first draw affected the probability of the second draw.
There are 5 blue marbles.
There are a total of 14 marbles left.
P(blue after red) = 
P(red, then blue) = P(red) · P(blue after red) = 
The probability of drawing a red marble and then a blue marble is 
b) There are 5 blue marbles.
There are a total of 15 marbles.
P(blue) = 
The result of the first draw affected the probability of the second draw.
There are 4 blue marbles left.
There are a total of 14 marbles left.
P(blue after blue) = 
P(blue, then blue) = P(blue) · P(blue after blue) = 
The probability of drawing a red marble and then a blue marble is 
Video Lessons On Calculating The Probability Of Dependent Events
Example:
We have a box with 10 red marbles and 10 blue marbles. Find P(drawing two blue marbles).
Example:
A club of 9 people wants to choose a board of 3 officers: President, Vice-President and Secretary.
Assuming that the officers are chosen at random, what is the probability that the officers are
Marsha for President, Sabita for Vice-President and Robert for Secretary?
How do you calculate probability of two dependent events?
Example:
A box contains 3 pens, 2 markers and 1 highlighter. Tara selects one item at random and does not
return it to the box. She then selects a second item at random. What is the probability that Tara
selects one pen and then one marker?
Example:
Andrea has 8 blue socks and 4 red socks in her drawer. She chooses one sock at random and puts it on.
She then chooses another sock without looking. Find the probability of the following event P(red, then red).
Compare Dependent And Independent Events
Statistics - Dependent and Independent Events
This lesson teaches the distinction between Independent and Dependent Events, and how to calculate
the probability of each.
The probability of two events is independent if what happens in the first event does not affect the probability of the second event. P(A + B) = P(A) × P(B)
The probability of two events is dependent if what happens in the first event does affect the probability the second event. P(A + B) = P(A) × P(B after A)
Example 1: If I roll a pair of dice, what is the probability that both dice land on a 6? Are these dependent or independent events?
Example 2: There are 4 puppies; two are male and two are female. If you randomly pick two puppies, what is the probability that they will both be female? Are these Independent or Dependent Events?
Example 3: You randomly choose one of the letter cubes. Without replacing it, you now choose a 2nd letter cube and place it to the right of the 1st letter cube. Then you pick a 3rd letter cube, and place it to the right of the 2nd letter cube. What is the probability that the letter cubes now spell “BIT”?
Independent vs Dependent Probability
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.
We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.