Find Determinant using Row Reduction

You can find the determinant of a square matrix using row reduction (also known as Gaussian elimination) by transforming the matrix into an upper triangular form. The determinant of an upper triangular matrix is simply the product of its diagonal entries. However, you need to keep track of how the elementary row operations you perform affect the determinant.

The following diagram shows how to find the determinant using row reduction. Scroll down the page for more examples and solutions.

 

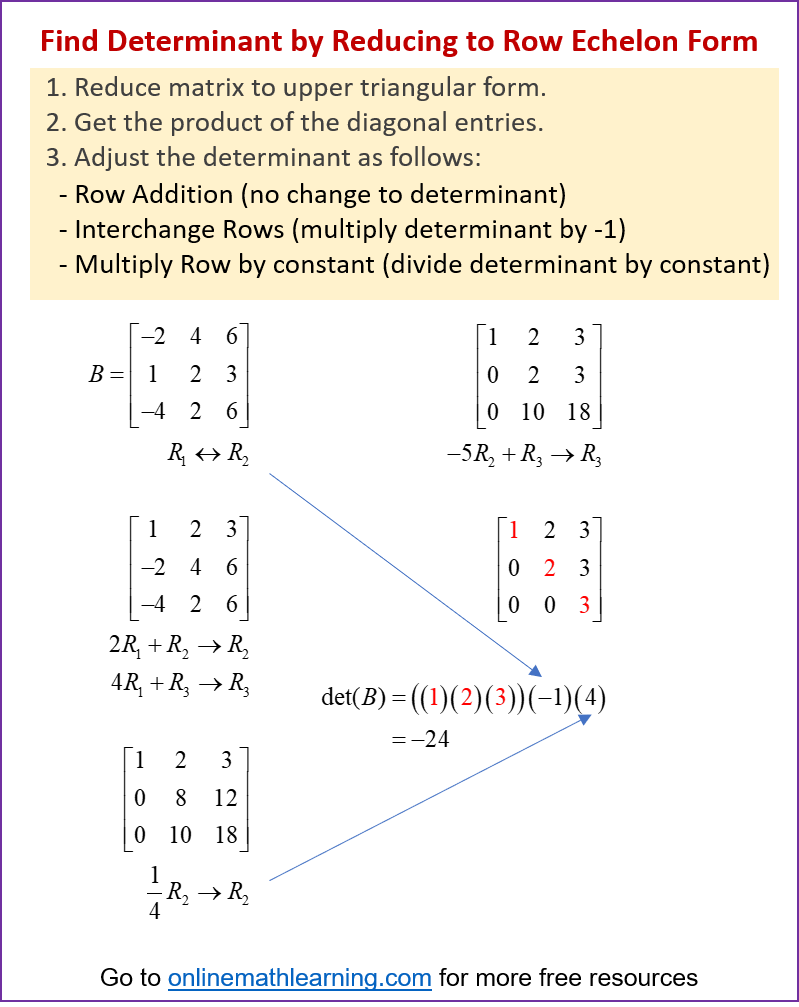
Elementary Row Operations and Their Effect on the Determinant:

1. Swapping two rows: If you swap two rows of a matrix, the determinant of the new matrix is the negative of the determinant of the original matrix.
   det(B) = -det(A)
   where B is the matrix obtained by swapping two rows of A.
   
2. Multiplying a row by a non-zero scalar (k): If you multiply a row of a matrix by a non-zero scalar k, the determinant of the new matrix is k times the determinant of the original matrix.
   det(B) = k * det(A)
   where B is the matrix obtained by multiplying a row of A by k.
   Conversely, if you factor out a scalar k from a row, the determinant is divided by k.
   
3. Adding a multiple of one row to another row: If you add a multiple of one row to another row, the determinant of the matrix does not change.
   

Steps to Find the Determinant via Row Reduction:

1. Start with the given matrix A.
   
2. Use elementary row operations to transform A into an upper triangular matrix U. An upper triangular matrix is a square matrix where all the entries below the main diagonal are zero. You should primarily use the operation of adding a multiple of one row to another, as this doesn’t change the determinant.
   
3. Keep track of any row swaps and row multiplications you perform.
   For each row swap, multiply the determinant by -1.
   For each row multiplication by a scalar k, the determinant of the resulting matrix is k times the original. To compensate,
   you’ll need to divide the final product of the diagonal entries by k.
   
4. Once you have the upper triangular matrix U, the determinant of U is the product of its diagonal entries.

Simplifying Determinants
Finding the determinant of a 3x3 square matrix or a larger square matrix can involve a lot of computation. To reduce the amount of computation, we can use methods for simplifying determinants. These methods for simplifying determinants involve using row or column operations to change some entries of the matrix to zeros.

Find Determinant=? by Reducing to Echelon Form
This video will show how to find the determinant of a 3x3 matrix by reducing the matrix to Echelon form.

Find Determinants with Row Reduction
We can calculate determinants using Gauss-Jordan elimination. If we reduced a matrix to triangular form the determinant is easy to calculate, then we can relate the determinant of the triangular matrix to the determinant of the original matrix by considering how the elementary row operations we performed must have changed the determinant.

• Show Video

Calculating a 4x4 determinant by putting in in upper triangular form first.

• Show Video

4x4 Determinant via Row Reduction
The example of 4x4 Determinant where row reduction makes computation significantly easier in comparison to direct minor method.

• Show Video

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.