Diagonals in Geometry

A series of free, online High School Geometry Video Lessons.
Examples, solutions, videos, worksheets, and activities to help Geometry students.

In this lesson, we will learn

• how to determine the number of diagonals in a polygon
• how to use mathematical model to determine the number of handshakes possible in a group of certain number of people

The following diagram gives the formula for the number of diagonals in an n-sided polygon. Scroll down the page for more examples and solutions.

 

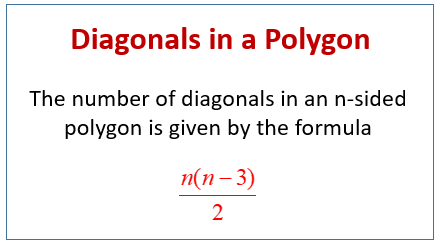
Number of Diagonals in a Polygon

A diagonal is a segment that connects two non-consecutive vertices in a polygon. The number of diagonals in a polygon that can be drawn from any vertex in a polygon is three less than the number of sides. To find the total number of diagonals in a polygon, multiply the number of diagonals per vertex (n - 3) by the number of vertices, n, and divide by 2 (otherwise each diagonal is counted twice).
How to determine the number of diagonals in a polygon?

Number of Handshakes

Mathematical modeling is used to generate a formula for the number of handshakes possible. The situation essentially asks how many sides and diagonals are in a given polygon and generates the triangle numbers: 1, 3, 6, 10, 15,
How to determine the number of handshakes possible in a group of certain number of people.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.