Factoring Difference of Squares

In some cases recognizing some common patterns in the trinomial or binomial will help you to factor it faster. For example, we could check whether the binomial is a difference of squares.

The following diagram gives examples of factoring difference of squares. Scroll down the page for more examples and solutions.

 

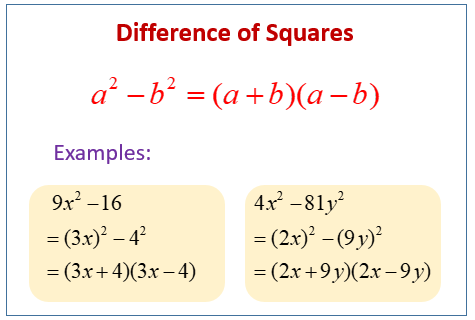
How to factor Difference of Squares?
A difference of squares is a binomial of the form:

a² – b²

Take note that the first term and the last term are both perfect squares.

When we factor a difference of two squares, we will get

a² – b² = (a + b)(a – b)

This is because (a + b)(a – b) = a²– ab + ab – b² = a²– b²

Example:
x² – 25 = 0
x² – 5² = 0
(x + 5)(x – 5) = 0

We get two values for x: x + 5 ⇒ x = –5
x – 5 ⇒ x = 5

Be careful! This method only works for difference of two squares and not for the sum of two squares:
a² + b² ≠ (a + b)(a – b)

Example:

Factor
a) x²– 9
b) 4x²– 25
c) 2x²– 32
d) πR²– πr²

Solution:

a) x²– 9
= x²– 3²
= (x + 3)(x – 3)

b) 4x²– 25
= (2x)²– (5)²
= (2x + 5)(2x – 5)

c) 2x²– 32
= 2(x²– 16)
= 2(x² – 4²)
= 2(x + 4)(x – 4)

d) πR²– πr²
= π(R²– r²)
= π(R + r)(R – r)

The following videos explain how to factor a difference of squares.

Example:
Factor
x² - 9
y² - 1
16x² - 25y²
x⁴ - 1
2x² - 72

• Show Video

Example:
Factor
w² - 81
b² - 1/4
27a² - 147

• Show Video

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.