Direct Variation
Videos, worksheets, solutions, and activities to help Algebra students learn about direct variation or direct proportion. What is direct variation and how to solve direct variation problems?
What is Direct Variation?
We often use the term direct variation to describe a form of dependence of one variable on another. An equation that makes a line and crosses the origin is a form of direct variation, where the magnitude of x increases or decreases directly as y increases or decreases. Direct variation and inverse variation are used often in science when modeling activity, such as speed or velocity.
Some real life examples of direct variation are:
The number of hours you work and the amount of your paycheck
The amount of weight on a spring and the distance the spring will stretch
The speed of a car and the distance traveled in a certain amount of time
The following diagram shows the key features of Direct Variation. Scroll down the page for examples and solutions.
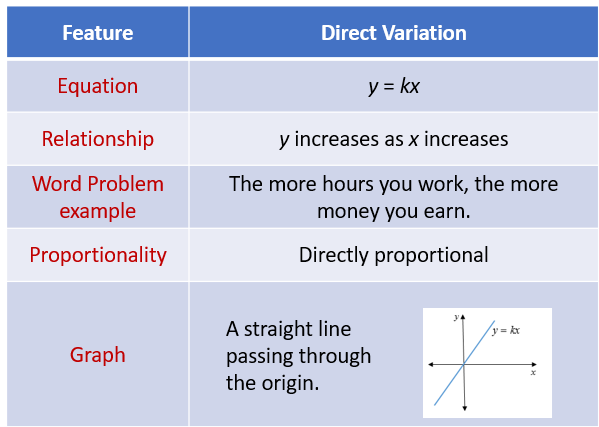
Variation Word Problems
Direct Variation Problems
Inverse Variation Problems
Joint & Combined Variations
How to solve direct variation problems?
In general, if two quantities vary directly, if one goes up the other goes up or down proportionally.
Graphically, we have a line that passed through the origin. The slope is k, which is called the constant of proportionality.
Key Points:
- In direct variation, y = kx, where k is the constant of variation.
- To solve a direct variation problem:
- Write the equation y = kx.
- Use the given values to find k.
- Substitute k and the new value of x to find y.
Examples:
- Y varies directly with x. y = 54 when x = 9. Determine the direct variation equation and then determine y when x = 3.5.
- Hooke’s Law states that the displacement, d that a spring is stretched by a hanging object caries directly as the mass, m of the object. If the distance is 10 cm when the mass is 3 kg, what is the distance when the mass is 5 kg?
- Y varies directly with with the square of x. y = 32 when x = 4. Determine the direct variation equation and then determine y when x = 6.
What is the Direct Variation or Direct Proportionality Formula?
Ever heard of two things being directly proportional? Well, a good example is speed and distance. The bigger your speed, the farther you’ll go over a given time period. So as one variable goes up, the other goes up too, and that’s the idea of direct proportionality. But you can express direct proportionality using equations, and that’s an important thing to do in algebra. See how to do that in the tutorial.
Algebra Word Problem: Variation Application
Solving a Variation problem
Example:
Art’s wages are directly proportional to the number of hours he works per week. If Art works 36 hours in a week, he earns $540. What are his wages if he works 40 hours in a week?
Direct Variation Models
Examples:
- The current standard for low-flow showerheads is 2.5 gallons per minute. Calculate how long it would take to fill a 30 gallon bathtub using such a showerhead to supply the water.
- Amen is using a hose to fill his swimming pool for the first time. He starts the hose at 10 P.M. and leaves it running all night. At 6 A.M. he measures the depth and calculates that the pool is four-sevenths full. At what time will his new pool be full?
Direct Variation Word Problems
Determine the constant of variation and find the other missing value using the given information.
Solve a direct variation equation y = kx, where k is the constant of variation.
A direct variation graph is a straight line graph that passes through the origin.
Example:
The distance required to stop a car varies directly as the square of its speed. If 200 feet are required to stop a car traveling 60 miles per hour, how many feet are required to stop a car traveling 100 miles per hour?
Check out many other Algebra Word Problems
Age Word Problems, Average Word Problems, Coin Word Problems, Consecutive Integer Word Problems, Digit Word Problems, Distance Word Problems, Fraction Word Problems, Geometry Word Problems, Integer Word Problems, Interest Word Problems, Lever Word Problems, Mixture Word Problems, Money Word Problems, Motion & Distance Word Problems, Number Sequence Word Problems, Proportion Word Problems, Quadratic Equation Word Problems, Ratio Word Problems, Symbol Word Problems, Variation Word Problems, Work Word Problems.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.