Distance of a Point from a Line
Examples, solutions, videos, activities, and worksheets that are suitable for A Level Maths.
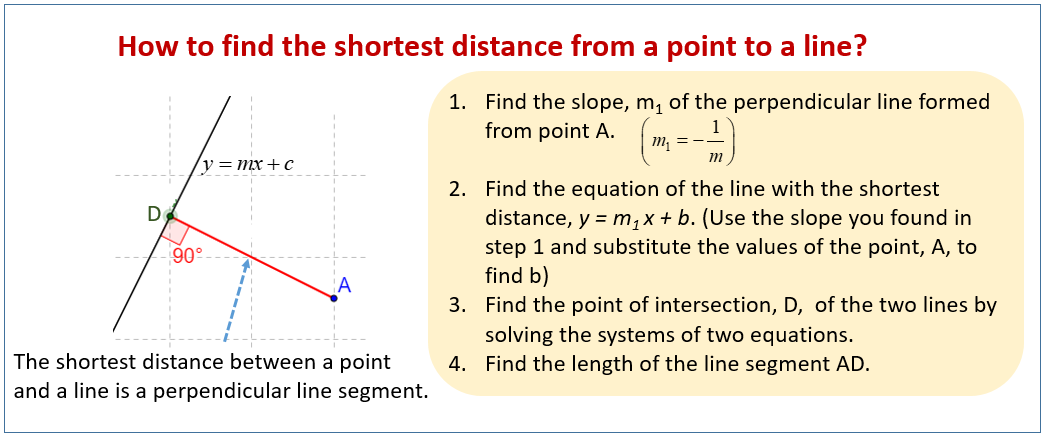
How to find the shortest distance from a point to a given line?
The shortest distance between a point and a line is a perpendicular line segment.
- Find the slope of the perpendicular line formed from the point. (Negative reciprocal from the given line)
- Find the equation of the line with the shortest distance y = mx + b. (Use the slope you found in step 1 and substitute the values of the point to find the b value)
- Find the point of intersection of the two lines by solving the systems of two equations.
- Find the length of the line segment by using the point of intersection from step 3 to the given point.
The following diagram gives the steps to find the shortest distance between a point and a line. Scroll down the page for more examples and solutions.
Shortest Distance from a Point to a Line Part 1
The following lesson looks at Shortest Distance from a Point to a Line. This is the first part of 3 videos
Shortest Distance from Point to a Line Part 2
The following lesson looks at Shortest Distance from a Point to a Line. This video continues from Part 1.
Shortest Distance From a Point to Line Part 3
The following lesson looks at Shortest Distance from a Point to a Line. This video is the final part continuing from part 2
Shortest Distance between a Point and Line using Equations of Lines
Use equations of lines
- Find equation of second line (slope is negative reciprocal)
- Find point of intersection
- Use distance formula
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.