Illustrative Mathematics Unit 6.6, Lesson 10: The Distributive Property, Part 2

Related Pages
Illustrative Math
Grade 6

Lesson 10: The Distributive Property, Part 2

Let’s use rectangles to understand the distributive property with variables.

Illustrative Math Unit 6.6, Lesson 10 (printable worksheets)

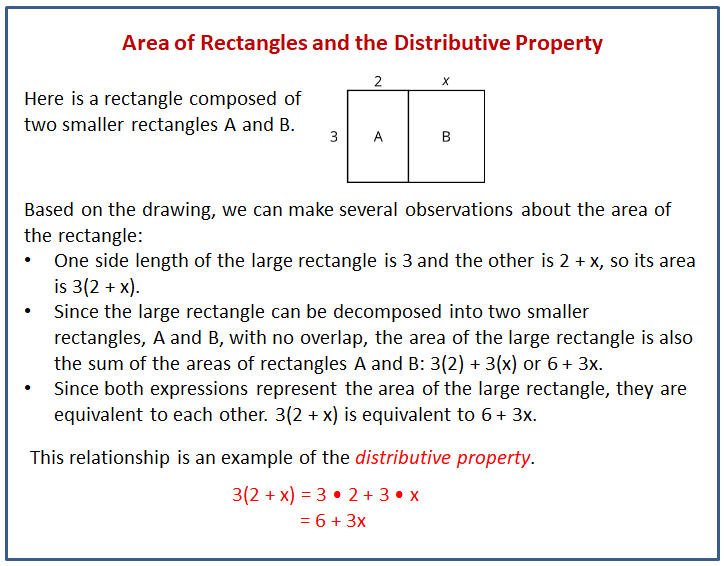
Lesson 10 Summary

The following diagram shows how to use rectangles to understand the distributive property with variables.

Lesson 10.1 Possible Areas

1. A rectangle has a width of 4 units and a length of m units. Write an expression for the area of this rectangle.
2. What is the area of the rectangle if m is 3 units? 2.2 units? 1/5 unit?
3. Could the area of this rectangle be 11 square units? Why or why not?

• See Video for Whole Lesson

Scroll down the page for the solutions to the “Are you ready for more?” section.

Lesson 10.2 Partitioned Rectangles When Lengths are Unknown

1. Here are two rectangles. The length and width of one rectangle are 8 and 5. The width of the other rectangle is 5, but its length is unknown so we labeled it x. Write an expression for the sum of the areas of the two rectangles.
2. The two rectangles can be composed into one larger rectangle as shown. What are the width and length of the new, large rectangle?
3. Write an expression for the total area of the large rectangle as the product of its width and its length.

Lesson 10.3 Areas of Partitioned Rectangles

For each rectangle, write expressions for the length and width and two expressions for the total area. Record them in the table. Check your expressions in each row with your group and discuss any disagreements.

width	length	area as a product of width times length	area as a sum of the areas of the smaller rectangles

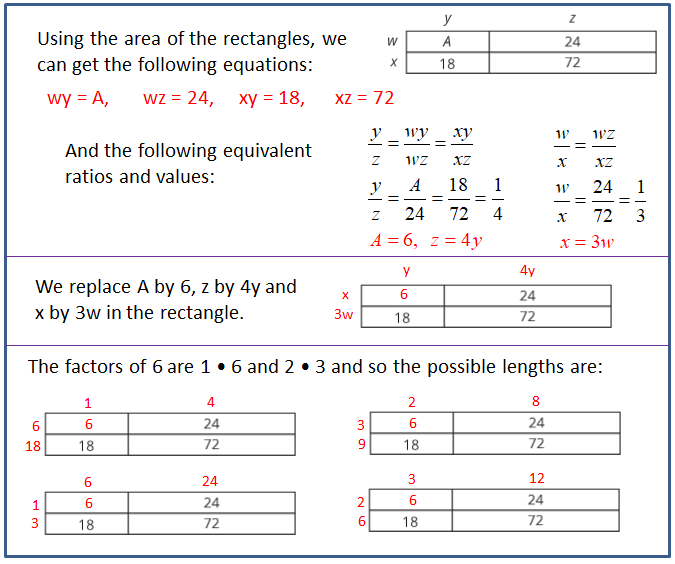
Are you ready for more?

Here is an area diagram of a rectangle.

1. Find the lengths w, x, y, and z, and the area A. All values are whole numbers.
2. Can you find another set of lengths that will work? How many possibilities are there?
   • Show Answer

     

Lesson 10 Practice Problems

1. Here is a rectangle.
   a. Explain why the area of the large rectangle is 2a + 3a + 4a.
   b. Explain why the area of the large rectangle is (2 + 3 + 4)a.
   
2. Is the area of the shaded rectangle 6(2 - m)or 6(m - 2)?
   Explain how you know.
   
3. Choose the expressions that do not represent the total area of the rectangle. Select all that apply.
   A. 5t + 4t
   B. t + 5 +4
   C. 9t
   D. 4 · 5 · t
   E. t(5 + 4)
   
4. Evaluate each expression mentally.
   a. 35 · 91 - 35 · 89
   b. 22 · 87 + 22 · 13
   c. 9/11 · 7/10 - 9/11 · 3/10
   
5. Select all the expressions that are equivalent to 4b.
   A. b + b + b + b
   B. b + 4
   C. 2b + 2b
   D. b · b · b · b
   E. b ÷ 1/4
   
6. Solve each equation. Show your reasoning.
   a. 111 = 14g
   b. 13.65 = h + 4.88
   c. k + 1/3 = 5 1/8
   d. 2/3 m = 17/4
   e. 5.16 = 4n
   
7. Andre ran 5 1/2 laps of a track in 8 minutes at a constant speed. It took Andre x minutes to run each lap. Select all the equations that represent this situation.
   A. (5 1/2)x = 8
   B. 5 1/2 + x = 8
   C. 5 1/2 - x = 8
   D. 5 1/2 ÷ x = 8
   E. x = 8 ÷ (5 /2)
   F. x = (5 1/2) ÷ 8

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.