Distributive Property

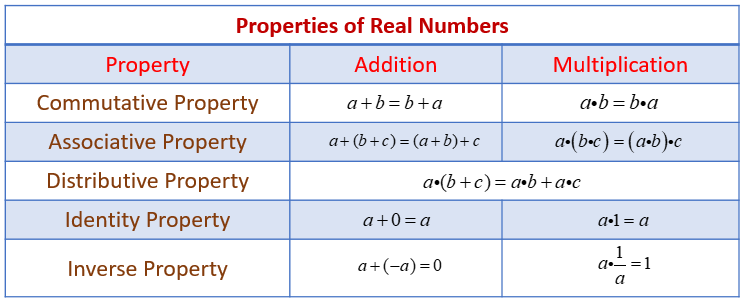
Summary of Number Properties

The following table summarizes the number properties for addition and multiplication: Commutative, Associative, Distributive, Identity and Inverse. Scroll down the page for examples, explanations and solutions.

Distributive property allows you to remove the parenthesis (or brackets) in an expression. Multiply the value outside the brackets with each of the terms in the brackets.

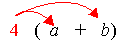
For example:	4(a + b) = 4a + 4b
	7(2c – 3d + 5) = 14c – 21d + 35

What happens if you need to multiply (a – 3)(b + 4)?

You do the same thing but with one value at a time.

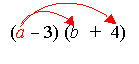
For example:

Multiply a with each term to get a × b + 4 × a = ab + 4a

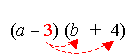
Then, multiply 3 with each term to get “ –3b – 12” (take note of the sign operations).

Put the two results together to get “ab + 4a – 3b – 12”

Therefore, (a – 3)(b + 4) = ab + 4a – 3b – 12

The following video shows more examples of the distributive property.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.