Dividing Polynomials and the Remainder Theorem

Dividing polynomials using long division and synthetic division are two methods for simplifying polynomial division. While both methods achieve the same result, they differ in their approach, ease of use, and applicability.

The Remainder Theorem is a useful tool in algebra that provides a quick way to find the remainder when a polynomial f(x) is divided by a linear divisor of the form x − c. It is closely related to polynomial division and synthetic division.

The following diagrams show how to divide polynomials using long division and synthetic division. Scroll down the page for more examples and solutions.

 

• Printable & Online Polynomial Worksheets

  Add & Subtract Polynomials
  Multiply Polynomials
  Divide Polynomials
  

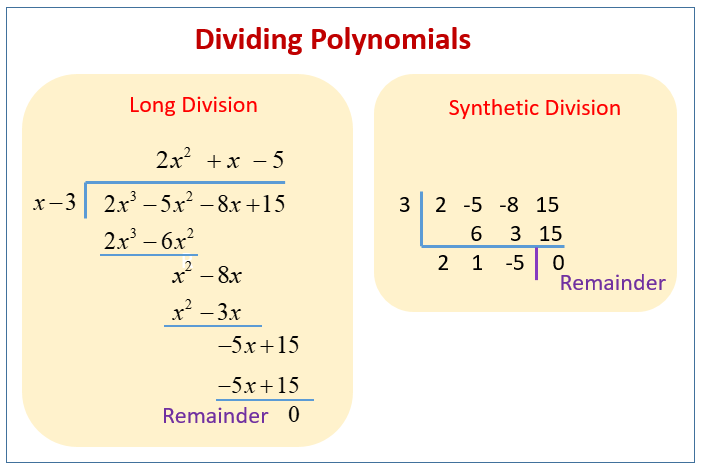
Polynomial Long Division
Polynomial long division is a method similar to numerical long division, used to divide one polynomial (the dividend) by another polynomial (the divisor). It can be used for any polynomial division, regardless of the degree of the divisor. It is particularly useful when the divisor is not linear

Steps:

1. Arrange the polynomials in descending order of exponents.
   
2. Divide the first term of the dividend by the first term of the divisor.
   
3. Multiply the result by the entire divisor and subtract from the dividend.
   
4. Repeat the process until the degree of the remainder is less than the degree of the divisor.
   

Synthetic Division
A shortcut method for dividing a polynomial by a linear divisor of the form x − c. It is ideal for quick calculations when the divisor is linear.

Steps:

1. Identify c from the divisor x − c.
   
2. Write the coefficients of the dividend.
   
3. Perform the synthetic division process:
   Bring down the first coefficient.
   Multiply by c and add to the next coefficient.
   Repeat until all coefficients are used.
   
4. The last number is the remainder, and the other numbers are the coefficients of the quotient.
   

Remainder Theorem Statement
If a polynomial f(x) is divided by x − c, the remainder is equal to f(c).

Steps to Use the Remainder Theorem:

1. Identify c from the divisor x − c, the value of c is used to evaluate f(c).
2. Evaluate f(c) by substituting x = c into the polynomial f(x).
3. The value of f(c) is the remainder when f(x) is divided by x − c.

Dividing Polynomials and The Remainder Theorem Part 1
This lesson shows how to divide a polynomial by a binomial using both long division and synthetic division. The lesson also discusses the Remainder Theorem and shows how to use it to find remainders in algebraic divisions

• Show Video

Dividing Polynomials and the Remainder Theorem Part 2
This lesson shows how to divide a polynomial by a binomial using both long division and synthetic division. The lesson also discusses the Remainder Theorem and shows how to use it to find remainders in algebraic divisions

• Show Video

Dividing Polynomials and the Remainder Theorem Part 3
This lesson shows how to divide a polynomial by a binomial using both long division and synthetic division. The lesson also discusses the Remainder Theorem and shows how to use it to find remainders in algebraic divisions

• Show Video

Try out our new and fun Fraction Concoction Game.

Add and subtract fractions to make exciting fraction concoctions following a recipe. There are four levels of difficulty: Easy, medium, hard and insane. Practice the basics of fraction addition and subtraction or challenge yourself with the insane level.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.