Dividing Polynomials

In this lesson, we will look at how to divide polynomials by monomials.
To divide a polynomial by a monomial, each term of the polynomial is divided by the monomial. Be careful with the sign (+ or –) of each term in your answer.

You may want to look at the lessons on dividing polynomials by polynomials (also called long division) and synthetic division (a simplified form of long division)

The following diagram shows how to divide a polynomial by a monomial. Scroll down the page for more examples of dividing a polynomial by a monomial.

 

• Printable & Online Polynomial Worksheets

  Add & Subtract Polynomials
  Multiply Polynomials
  Divide Polynomials
  

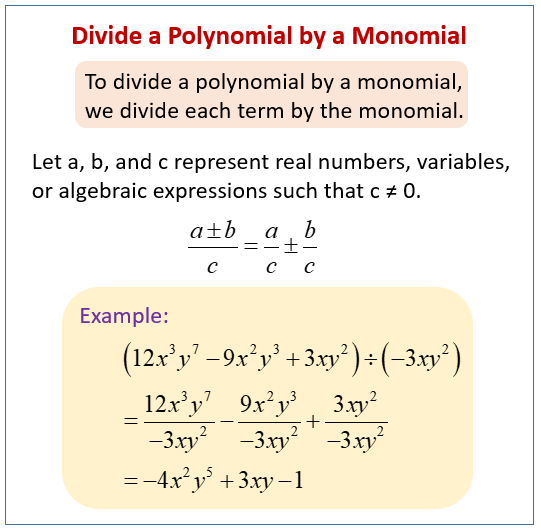
Example:

Evaluate (x² + 8x) ÷ x

Solution:

(x² + 8x) ÷ x
= [x² ÷ x] + [8x ÷ x]
= x + 8

Example:

Evaluate (4y⁴ – y³ + 2y²) ÷ (–y²)

Solution:

(4y⁴– y³ + 2y²) ÷ (–y²)
= [4y⁴ ÷ –y²] + [– y3 ÷ –y²] + [2y² ÷ –y²]
= –4y² + y – 2

Polynomial Division: Dividing by a Monomial

• Show Video

Explains and shows examples of how to divide a polynomial by a monomial

• Show Video

Dividing Polynomials by Monomials.

• Show Video

Try out our new and fun Fraction Concoction Game.

Add and subtract fractions to make exciting fraction concoctions following a recipe. There are four levels of difficulty: Easy, medium, hard and insane. Practice the basics of fraction addition and subtraction or challenge yourself with the insane level.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.