Illustrative Mathematics Unit 6.6, Lesson 15: Equivalent Exponential Expressions

Related Pages
Illustrative Math
Grade 6

Lesson 15: Equivalent Exponential Expressions

Let’s investigate expressions with variables and exponents.

Illustrative Math Unit 6.6, Lesson 15 (printable worksheets)

Lesson 15 Summary

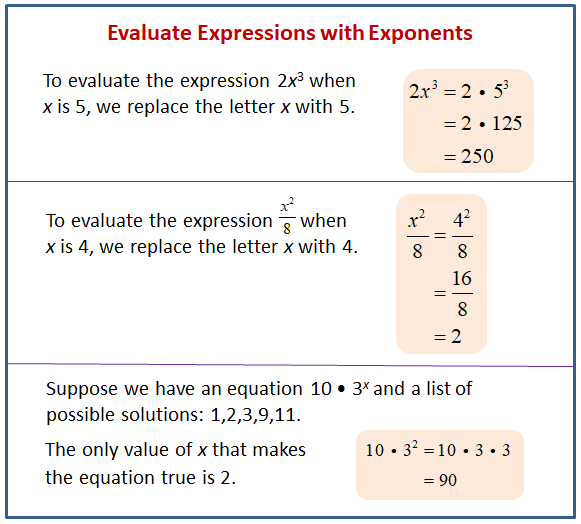
In this lesson, we saw expressions that used the letter x as a variable. We evaluated these expressions for different values of x.
The following diagram gives some examples of evaluating expressions with exponents by substituting values for x.

Lesson 15.1 Up or Down?

1. Find the values of 3^x and (⅓)^x for different values of x.
   
   

x	3x	(⅓)x
1
2
3
4

2. What patterns do you notice?

• See Video for Whole Lesson

Scroll down the page for the solutions to the “Are you ready for more?” section.

Lesson 15.2 What’s the Value?

Evaluate each expression for the given value of x.

1. 3x² when x is 10.
   
2. 3x² when x is 1/9.
   
3. x³/4 when x is 4.
   
4. x³/4 when x is 1/2.
   
5. 9 + x⁷ when x is 1.
   
6. 9 + x⁷ when x is 1/2.

Lesson 15.3 Exponent Experimentation

Find a solution to each equation in the list that follows. (Numbers in the list may be a solution to more than one equation, and not all numbers in the list will be used.)

Are you ready for more?

This fractal is called a Sierpinski Tetrahedron. A tetrahedron is a polyhedron that has four faces. (The plural of tetrahedron is tetrahedra.)
The small tetrahedra form four medium-sized tetrahedra: blue, red, yellow, and green. The medium-sized tetrahedra form one large tetrahedron.

1. How many small faces does this fractal have? Be sure to include faces you can’t see as well as those you can. Try to find a way to figure this out so that you don’t have to count every face.
   
2. How many small tetrahedra are in the bottom layer, touching the table?
   
3. To make an even bigger version of this fractal, you could take four fractals like the one pictured and put them together. Explain where you would attach the fractals to make a bigger tetrahedron.
   
4. How many small faces would this bigger fractal have? How many small tetrahedra would be in the bottom layer?
   
5. What other patterns can you find?
   
   • Show Answer

     

Lesson 15 Practice Problems

1. Evaluate the following expressions if x = 3.
   A. 2^x
   B. x²
   C. 1^x
   D. x¹
   E. (½)^x
   
2. Evaluate each expression for the given value of x.
   a. 2 + x³, x is 3
   b. x², x is ½
   c. 3x², x is 5
   d. 10 - x², x is 6
   
3. Decide if the expressions have the same value. If not, determine which expression has the larger value.
   a. 2³ and 3²
   b. 1³¹and 31¹
   c. 4²and 2⁴
   d. (½)³and (⅓)²
   
4. Match each equation to its solution.
   a. 7 + x² = 16
   b. 5 - x² = 1
   c. 2 · 2³
   d. 3⁴/3^x = 27
   
5. An adult pass at the amusement park costs 1.6 times as much as a child’s pass.
   a. How many dollars does an adult pass cost if a child’s pass costs:
   $5?
   $10?
   w
   b. A child’s pass costs $15. How many dollars does an adult pass cost?
   
6. Jada reads 5 pages every 20 minutes. At this rate, how many pages can she read in 1 hour? a. Use a double number line to find the answer.
   b. Use a table to find the answer.
   c. Explain which strategy you thinks works better in finding the answer.

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.