Illustrative Mathematics Grade 7, Unit 2, Lesson 1: One of These Things Is Not Like the Others

Related Pages Illustrative Math
Grade 7

Lesson 1: One of These Things Is Not Like the Others

Let’s remember what equivalent ratios are.

Illustrative Math Unit 7.2, Lesson 1 (printable worksheets)

Lesson 1 Summary

The following diagram shows how to use equivalent ratios for recipes and to describe scaled copies of shapes.

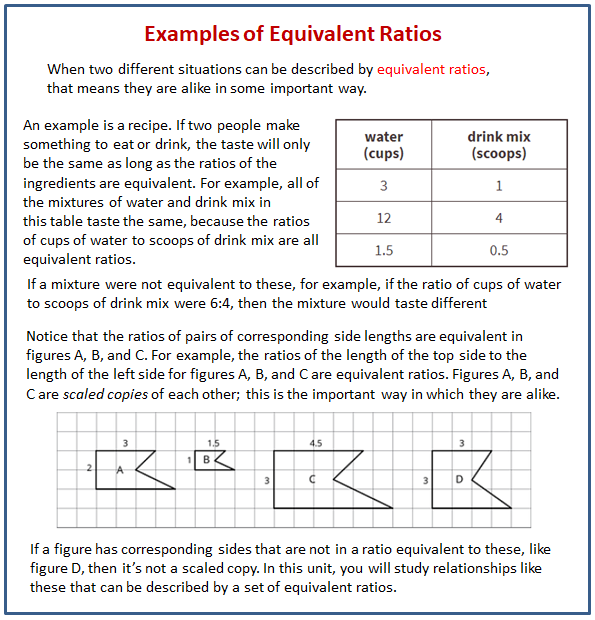
Lesson 1.1 Remembering Double Number Lines

1. Complete the double number line diagram with the missing numbers.
2. What could each of the number lines represent? Invent a situation and label the diagram.
3. Make sure your labels include appropriate units of measure.

• See Video for Whole Lesson

Lesson 1.2 Mystery Mixtures

Your teacher will show you three mixtures. Two taste the same, and one is different.

1. Which mixture tastes different? Describe how it is different.
   
2. Here are the recipes that were used to make the three mixtures:

• 1 cup of water with 1 1/2 teaspoons of powdered drink mix
• 2 cups of water with 1/2 teaspoon of powdered drink mix
• 1 cup of water with 1/4 teaspoon of powdered drink mix
  Which of these recipes is for the stronger tasting mixture? Explain how you know.
  

Are you ready for more?

Will any of these mixtures taste exactly the same?

• Mixture A: 2 cups water, 4 teaspoons salt, 0.25 cup sugar
• Mixture B: 1.5 cups water, 3 teaspoons salt, 0.2 cup sugar
• Mixture C: 1 cup water, 2 teaspoons salt, 0.125 cup sugar
  • Show Answers

    Mixture A: 2 : 4 : 0.25 = 2 : 4 : 1/4 = 8 : 16 : 1
    Mixture B: 1.5 : 3 : 0.2 = 15 : 30 : 2
    Mixture C: 1 : 2 : 0.125 = 1 : 2 : 1/8 = 8 : 16 : 1
    Mixture A and C will taste the same.

Lesson 1.3 Crescent Moons

Here are four different crescent moon shapes.
Open Applet

1. What do moons A, B, and C all have in common that the other one doesn’t?
2. Use numbers to describe how moons A, B, and C are different from moon D.
3. Use a table and a double number line diagram to represent how moons A, B, and C are different from moon D.

Are you ready for more?

Can you make one moon cover another by changing its size? What does that tell you about its dimensions?
Open Applet

Glossary Terms

equivalent ratios
Two ratios are equivalent if you can multiply each of the numbers in the first ratio by the same factor to get the numbers in the second ratio. For example, 8:6 is equivalent to 4:3, because 8 · 1/2 = 4 and 6 · 1/2 = 3.
A recipe for lemonade says to use 8 cups of water and 6 lemons. If we use 4 cups of water and 3 lemons, it will make half as much lemonade. Both recipes taste the same, because 8:6 and 4:3 are equivalent ratios.

Lesson 1 Practice Problems

1. Which one of these shapes is not like the others? Explain what makes it different by representing each width and height pair with a ratio.
2. In one version of a trail mix, there are 3 cups of peanuts mixed with 2 cups of raisins. In another version of trail mix, there are 4.5 cups of peanuts mixed with 3 cups of raisins. Are the ratios equivalent for the two mixes? Explain your reasoning.
3. For each object, choose an appropriate scale for a drawing that fits on a regular sheet of paper. Not all of the scales on the list will be used.
4. Which scale is equivalent to 1 cm to 1 km?
   A. 1 to 1000
   B. 10,000 to 1
   C. 1 to 100,000
   D. 100,000 to 1
   E. 1 to 1,000,000
   
5. a. Find 3 different ratios that are equivalent to 7:3.
   b. Explain why these ratios are equivalent.
   

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.