Exponents with Negative Bases

In this lesson, we will learn how to evaluate negative numbers raise to whole number exponents. The placement of parentheses is crucial when dealing with negative numbers raised to powers. It determines whether the negative sign is part of the base or not, leading to different results.

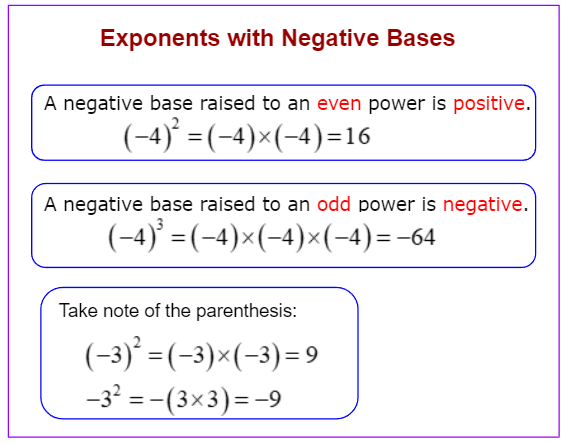
The following diagram shows how to evaluate exponents with negative bases. Scroll down the page for more examples and solutions.

 

Exponent Worksheets
Practice your skills with the following exponent worksheets:
Printable & Online Exponent Worksheets

When you have an expression like (-b)ⁿ, the sign of the final answer depends entirely on whether the exponent (n) is even or odd.

• Negative base raised to an even exponent: The result is positive. For example, (-2)⁴ = (-2) × (-2) × (-2) × (-2) = 16.
• Negative base raised to an odd exponent: The result is negative. For example, (-3)³ = (-3) × (-3) × (-3) = -27.

Important Note on Parentheses:
Case 1: Negative Number Inside Parentheses: (-b)ⁿ
When a negative number is enclosed in parentheses and then raised to a power, the entire number, including the negative sign, is the base.
(-b)ⁿ = (-b) × (-b) × (-b) × … (n times)
If n is even, the result will be positive. This is because the negative signs will pair up and multiply to positive values.
Example: (-3)² = (-3) × (-3) = 9
If n is odd, the result will be negative. This is because after pairing up as many negative signs as possible, one negative sign will be left over.
Example: (-3)³ = (-3) × (-3) × (-3) = 9 × (-3) = -27

Case 2: Negative Sign Without Parentheses: -bⁿ
When a negative sign appears in front of a base that is raised to a power, and there are no parentheses enclosing the negative sign with the base, the exponentiation operation is performed before the negation (the application of the negative sign). -bⁿ = -(b × b × b × … (n times))
In this case, you first calculate bⁿ (treating b as positive), and then you apply the negative sign to the result.
If n is even:
Example: -3² = -(3 × 3) = -9
If n is odd:
Example: -3³ = -(3 × 3 × 3) = -27

Key Difference Summarized:
The parentheses are crucial when dealing with negative bases.

• (-b)ⁿ means the entire negative number (-b) is raised to the power of (n).
  
• -bⁿ means the positive base (b) is raised to the power of (n), and then the negative sign is applied to the result.
  

Caution:
This distinction is a common source of errors. Always pay close attention to the presence and placement of parentheses when dealing with negative bases and exponents.

Evaluating Negative Numbers Raised to Powers
This video provides examples of evaluating negative numbers raise to powers with and without parentheses.
Examples:
Evaluate:
(-4)²
-4²
(-3)⁴
-3⁴
(-2)³
-2³

• Show Step-by-step Solutions

Exponents with negative bases and exponents with positive bases
ExamplesL
Simplify:
a. -2⁴
b. (-2)⁴

• Show Step-by-step Solutions

Nagative Bases
We can use the rules of the multiplication of negative numbers to determine the sign of a negative base raised to an exponent.
Remember that a negative number times a positive number is a negative number and that two negative numbers multiplied together is a positive number.
When negative bases are raised to odd numbered powers, they give us negative results.
When negative bases are raised to even numbered powers, they give us positive results.

• Show Step-by-step Solutions

Exponents with Negative Bases
Rules:
In negative base with brackets,
When the exponent is even, the answer is positive and
when the exponent is odd, the answer is negative.

In negative bases without brackets, the answer is always negative
Examples:
-1⁵ + 1²
(-2)³ + (-2)²
-2(-2)⁴ + (-3)³

• Show Step-by-step Solutions

Evaluate Powers
Learn how to evaluate powers.
Examples:

1. Evaluate.
   a) 3⁴
   b) -11²
   c) (-7)³
2. Express 81 in powers of 3.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.