Factor Trinomials by GCF
Related Topics:
More lessons for factoring and other Grade 9 topics
Examples, videos, worksheets, solutions, and activities to help students learn how to factor trinomials using the GCF.
When factoring trinomials, the first step would be to try to find the greatest common factor (GCF). We can then pull out the GCF by using the distributive property in reverse.
The following diagram shows how to factor trinomials using the Greatest Common Factor (GCF). Scroll down the page for more examples and solutions of factoring trinomials using GCF.
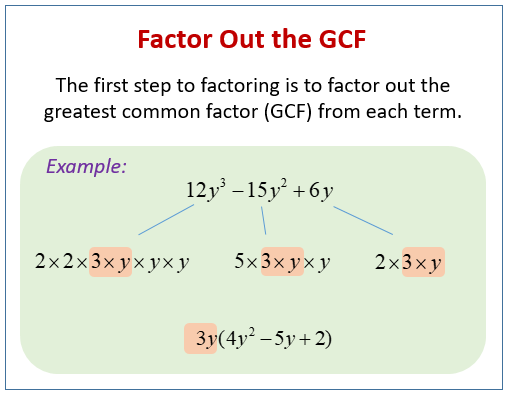
Steps to Find the GCF Using Prime Factors
- Express each term as a product of prime factors.
- Find the prime factors that are common to all the terms.
- Multiply the common prime factors to get the GCF of the terms.
- Factor out the GCF.
Different Methods to Factor Trinomials
Factor Trinomials Worksheets
Practice your skills with the following Algebra Worksheets.
Printable & Online Algebra Worksheets
Find the Greatest Common Factor - GCF
We can factor trinomials by first looking for factors that are common (that is the GCF)
Example:
Factor the following trinomials:
a) ad + dc + df
b) 2pq + 6p2q - 4 p3q
Solution:
a) ad + dc + df = d(a + c + f ) ← extract GCF d
b) 2pq + 6p2q – 4p3q = 2pq(1 + 3p – 2p2) ← extract GCF 2pq
How to factor trinomials with a negative leading coefficient?
Factor trinomial with negative in front.
Example:
Factor: -30x2 + 7x + 4
How to factor a trinomial with negative leading coefficient?
Example:
Factor: -6x2 - x + 7
How to find common factors as a first step in factoring a quadratic equation?
Factor: 5w2 - 20w - 160
How to factor the greatest common factor (gcf) from a polynomial?
Factor: 4x3 - 2x2 + 6x
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.