Factorial Notation
The expression 6 × 5 × 4 × 3 × 2 × 1 = can be written as 6!, which is read as “six factorial.”
In general, n! is the product of all the counting numbers beginning with n and counting backwards to 1. We define 0! to be 1.
The following diagram describes the factorial notation and gives some examples using factorials. Scroll down the page for more examples and solutions using factorials.
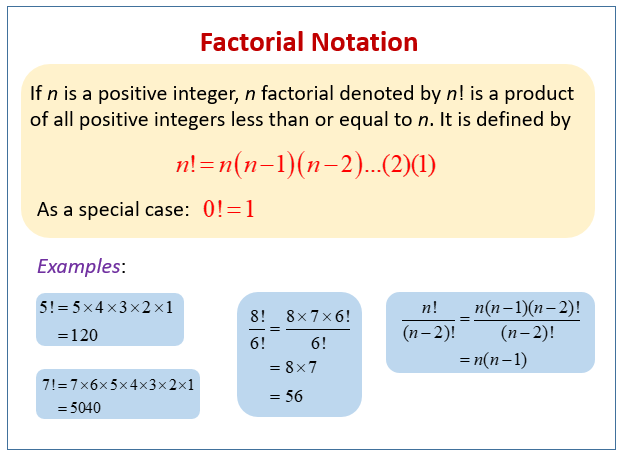
Example:
Find the value of each expression:
a) 3!
b) 0!
c) 5!
d) 1!
e) 3! + 2!
f) ![]()
Solution:
a) 3! = 3 × 2 × 1 = 6
b) 0! = 1
c) 5! = 5 × 4 × 3 × 2 × 1 = 120
d) 1! = 1
e) 3! + 2! = (3 × 2 × 1) + (2 × 1) = 8
f) ![]()
Factorials Explained!
Some basic information on factorials and shows how to evaluate some factorial examples.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.