Factoring by Common Factors & by Grouping

Related Pages
More Factoring and Algebra Lessons
Algebra Worksheets
Algebra Games

The following diagram shows the steps to factor a polynomial with four terms using grouping. Scroll down the page for examples and solutions.

 

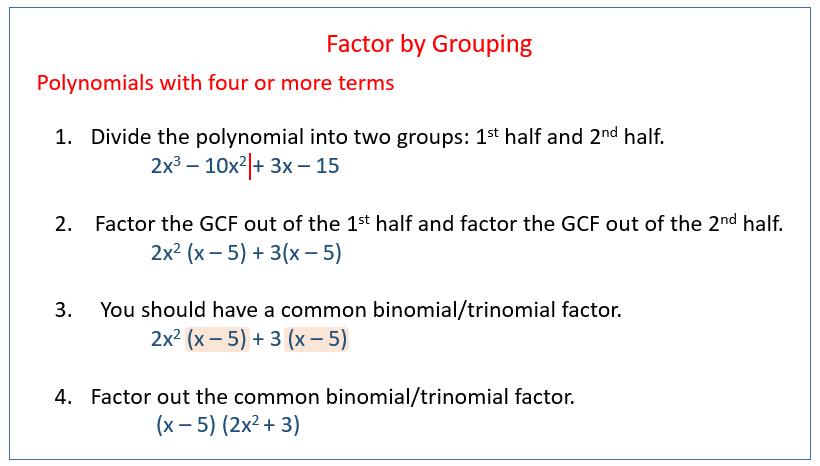
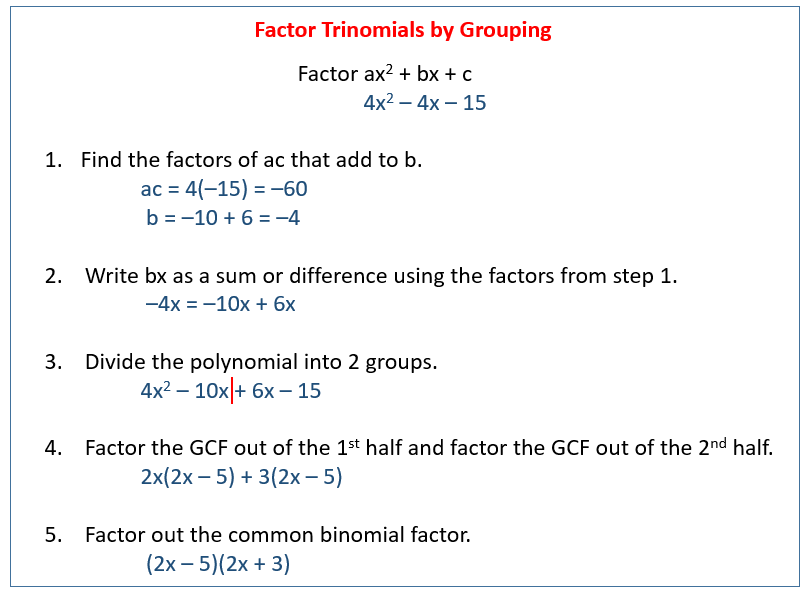
The following diagram shows the steps to factor a trinomial using grouping. Scroll down the page for examples and solutions.

 

Factoring By Common Factors

The first step in factorizing is to find and extract the GCF of all the terms.

Example:
Factorize the following algebraic expressions:
a) xyz – x²z
b) 6a²b + 4bc

Solution:
a) xyz – x²z = xz(y – x)
b) 6a²b + 4bc = 2b(3a² + 2c)

Factoring Out The Greatest Common Factor
Factoring is a technique that is useful when trying to solve polynomial equations algebraically.
We begin by looking for the Greatest Common Factor (GCF) of a polynomial expression.
The GCF is the largest monomial that divides (is a factor of) each term of of the polynomial.
The following video shows an example of simple factoring or factoring by common factors.
To find the GCF of a Polynomial

1. Write each term in prime factored form
   
2. Identify the factors common in all terms
   
3. Factor out the GCF

Examples:
Factor out the GCF

1. 2x⁴ - 16x³
2. 4x²y³ + 20xy² + 12xy
3. -2x³ + 8x² - 4x
4. -y³ - 2y² + y - 7

• Show Video Lesson

Factoring Using the Great Common Factor, GCF - Example 1
Two examples of factoring out the greatest common factor to rewrite a polynomial expression.

Example:
Factor out the GCF:
a) 2x³y⁸ + 6x⁴y² + 10x⁵y¹⁰
b) 6a¹⁰b⁸ + 3a⁷b⁴ - 24a⁵b⁶

• Show Video Lesson

Factoring Using the Great Common Factor, GCF - Example 2

Example:
Factor out binomial expressions.
a) 3x²(2x + 5y) + 7y²(2x + 5y)
b) 5x²(x + 3y) - 15x³(x + 3y)

• Show Video Lesson

Factoring Polynomials with Common Factors
This video provides examples of how to factor polynomials that require factoring out the GCF as the first step. Then other methods are used to completely factor the polynomial.

Example:
Factor
4x² - 64
3x² + 3x - 36
2x² - 28x + 98

• Show Video Lesson

Factoring By Grouping

When an expression has an even number of terms and there are no common factors for all the terms, we may group the terms into pairs and find the common factor for each pair:

Example:
Factorize the following expressions:

a) ax + ay + bx + by
b) 2x + 8y – 3px –12py
c) 3x – 3y + 4ay – 4ax

Solution:
a) ax + ay + bx + by
= a(x + y) + b(x + y)
= (a + b)(x + y)

b) 2x + 8y – 3px –12py
= 2(x + 4y) –3p(x + 4y)
= (2 – 3p)(x + 4y)

c) 3x – 3y + 4ay – 4ax
= 3(x – y) + 4a(y – x)
= 3(x – y) – 4a(x – y)
= (3 – 4a)( x – y)

How to Factor by Grouping?
3 complete examples of solving quadratic equations using factoring by grouping are shown.

Examples:

1. Factor x(x + 1) - 5(x + 1)
   
2. Solve 2x² + 5x + 2 = 0
   
3. Solve 7x² + 16x + 4 = 0
   
4. Solve 6x² - 17x + 12 = 0

• Show Video Lesson

Factoring by Grouping - Ex 1

Example:
Factor:
a) 2x² + 7x² + 2x + 7
b) 10x² + 2xy + 15xy + 3y²

• Show Video Lesson

Factoring By Grouping - Ex 2

Example:
Factor:
12u² + 15uv + 24uv² + 30v³

• Show Video Lesson

Factoring Trinomials: Factor by Grouping - ex 1

Example:
Factor 12x² + 34x + 10

• Show Video Lesson

Factoring Trinomials: Factor by Grouping - ex 2

Example:
Factor 6x² + 15x - 21

• Show Video Lesson

Factoring by grouping - Prime Factorization

Example:
12a³ - 9a²b - 8ab² + 6b³

• Show Video Lesson

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.