Factoring Polynomials - GCF
Related Topics:
More Lessons for Grade 9 Math
Math Worksheets
Examples, videos, worksheets, solutions, and activities to help Algebra 1 students learn how to factor polynomials using the GCF.
Factoring polynomials is the process of breaking down a polynomial into simpler polynomials (called factors) that, when multiplied together, give the original polynomial. Factoring is a key skill in algebra and is used to simplify expressions, solve equations, and analyze functions.
The following diagram shows how to factor polynomials using the Greatest Common Factor (GCF). Scroll down the page for more examples and solutions of factoring polynomials using the GCF.
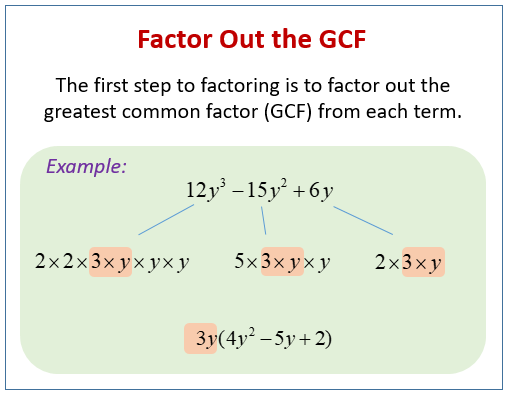
Different Methods to Factor Trinomials & Polynomials
Factor Polynomials Worksheets
Practice your skills with the following Algebra Worksheets.
Printable & Online Algebra Worksheets
Here are some common factoring techniques for polynomials:
- Greatest Common Factor (GCF):
Identify the GCF: Find the largest factor that divides evenly into all terms.
Factor Out the GCF: Divide each term by the GCF and write the expression in factored form.
Example: 4x³ + 8x² - 12x = 4x(x² + 2x - 3) - Factoring by Grouping:
Used for polynomials with four or more terms.
Group Terms: Group the terms into pairs.
Factor Each Group: Factor out the GCF from each group.
Factor the Common Binomial: If both groups share a common binomial factor, factor it out.
Example: x³ + 3x² + 2x + 6 = (x³ + 3x²) + (2x + 6) = x²(x + 3) + 2(x + 3) = (x + 3)(x² + 2) - Factoring Trinomials (ax² + bx + c):
Simple Trinomials (a = 1):
Find two numbers that multiply to ‘c’ and add to ‘b’.
Write the factored form as (x + number 1)(x + number 2).
Example: x² + 5x + 6 = (x + 2)(x + 3)
Trinomials (a ≠ 1):
“ac” Method:
Multiply ‘a’ and ‘c’.
Find two numbers that multiply to ‘ac’ and add to ‘b’.
Rewrite the middle term (‘bx’) using these two numbers.
Factor by grouping.
Example: 2x² + 7x + 3
ac = 2 × 3 = 6
Numbers: 1 and 6
2x² + 1x + 6x + 3 = x(2x + 1) + 3(2x + 1) = (2x + 1)(x + 3)
Trial and Error:
Try different combinations of factors of ‘a’ and ‘c’ until you find the correct combination. - Difference of Squares (a² - b²):
Pattern: a² - b² = (a + b)(a - b)
Example: x² - 9 = (x + 3)(x - 3) - Sum and Difference of Cubes (a³ ± b³):
Sum of Cubes: a³ + b³ = (a + b)(a² - ab + b²)
Difference of Cubes: a³ - b³ = (a - b)(a² + ab + b²)
Example: x³ + 8 = (x + 2)(x² - 2x + 4) - Perfect Square Trinomials (a² ± 2ab + b²):
Pattern: a² + 2ab + b² = (a + b)²
Pattern: a² - 2ab + b² = (a - b)²
Example: x² + 6x + 9 = (x + 3)²
Factoring Polynomials - GCF (part 1) Factoring Polynomials
A little background information and an introduction to factoring out the greatest common factor.
Factor:
5x + 10
6x + 12
Factoring Polynomials - GCF (part 2) Factoring Polynomials
More examples for factoring out the greatest common factor.
Factor:
60x3 - 50 x2
84x3y4 - 28x5y2 + 14x2y2
Factoring Polynomials - GCF (part 3)
More examples and information for factoring out the greatest common factor.
Factor:
-8x - 12
6x - 15
32x3 - 20x
36x4y2 - 24xy3 + 44x2y2
Factoring Using the Greatest Common Factor, GCF
Factor out the GCF:
a) 2x3y8 + 6x4y2 + 10x5y10
b) 6a10b8 + 3a7b4 - 24a5b6
Try out our new and fun Fraction Concoction Game.
Add and subtract fractions to make exciting fraction concoctions following a recipe. There are four levels of difficulty: Easy, medium, hard and insane. Practice the basics of fraction addition and subtraction or challenge yourself with the insane level.
We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.