Factoring Quadratic Equations
The following diagram shows how to factor and solve quadratic equations. Scroll down the page for more examples and solutions of factoring and solving quadratic equations.
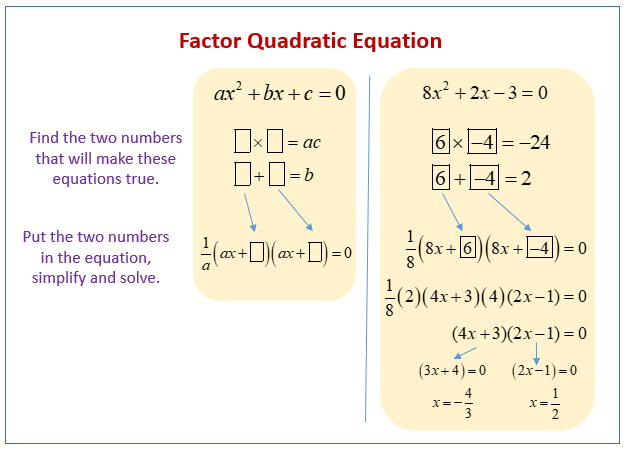
How to factor quadratic equations with no guessing and no trial and error?
Quadratic Equations (Factoring and solving when a ≠ 1)
When factoring Quadratic Equations, of the form:
ax2* + bx + c* = 0 where *a*, *b* and *c* are numbers and *a* ≠ 0.
we try to find common factors, and then look for patterns that will help you to factorize the quadratic equation. For example: Square of Sum, Square of Difference and Difference of Two Squares.
In other cases, you will have to try out different possibilities to get the right factors for quadratic equations.
If the Coefficient Of x2 Is 1
To factorize quadratic equations of the form: x2 + bx + c, you will need to find two numbers whose product is c and whose sum is b.

Example 1:(b and c are both positive)
Solve the quadratic equation: x2 + 7x + 10 = 0
Step 1: List out the factors of 10:
1 × 10, 2 × 5
Step 2: Find the factors whose sum is 7:
1 + 10 ≠ 7
2 + 5 = 7 ![]()
Step 3: Write out the factors and check using the distributive property.
(x + 2)(x + 5) = x2 + 5x + 2x + 10 = x2 + 7x + 10
The factors are (x + 2)(x + 5)
Step 4: Going back to the original quadratic equation
x2 + 7x + 10 = 0 Factorize the left side of the quadratic equation
(x + 2) (x + 5) = 0
x + 2 = 0 ⇒ x = -2, or
x + 5 = 0 ⇒ x = -5
Example 2:(b is positive and c is negative)
Get the values of x for the equation: x2 + 4x – 5 = 0
Step 1: List out the factors of – 5:
1 × –5, –1 × 5
Step 2: Find the factors whose sum is 4:
1 – 5 ≠ 4
–1 + 5 = 4 ![]()
Step 3: Write out the factors and check using the distributive property.
(x – 1)(x + 5)= x2 + 5x – x – 5 = x2 + 4x – 5
Step 4: Going back to the original quadratic equation
x2 + 4x – 5 = 0 Factorize the left hand side of the equation
(x – 1)(x + 5) = 0
x - 1 = 0 ⇒ x = 1, or
x + 5 = 0 ⇒ x = -5
Example 3:(b and c are both negative)
Get the values of x for the equation: x2 – 5x – 6
Step 1: List out the factors of – 6:
1 × –6, –1 × 6, 2 × –3, –2 × 3
Step 2: Find the factors whose sum is –5:
1 + ( –6) = –5 ![]()
Step 3: Write out the factors and check using the distributive property.
(x + 1) (x – 6) = x2 – 6 x + x – 6 = x2 – 5x – 6
Step 4: Going back to the original quadratic equation
x2 – 5x – 6 = 0 Factorize the left hand side of the equation
(x + 1) (x – 6) = 0
x + 1 = 0 ⇒ x = -1, or
x - 6 = 0 ⇒ x = 6
Example 4:* (b is negative and c is positive)*
Get the values of x for the equation: x2 – 6x + 8 = 0
Step 1: List out the factors of 8:
We need to get the negative factors of 8 to get a negative sum.
–1 × – 8, –2 × –4
Step 2: Find the factors whose sum is – 6:
–1 + ( –8) ≠ –6
–2 + ( –4) = –6 ![]()
Step 3: Write out the factors and check using the distributive property.
(x – 2) (x – 4) = x2 – 4 x – 2x + 8 = x2 – 6x + 8
Step 4: Going back to the original quadratic equation
x2 – 6x + 8 = 0 Factorize the left hand side of the equation
(x – 2) (x – 4) = 0
x - 2 = 0 ⇒ x = 2, or
x - 4 = 0 ⇒ x = 4
If the Coefficient of x2 Is Greater Than 1
Sometimes the coefficient of x in quadratic equations may not be 1 but the expression can be simplified by finding common factors.
When the coefficient of x2 is greater than 1 and we cannot simplify the quadratic equation by finding common factors, we would need to consider the factors of the coefficient of x2 and the factors of c in order to get the numbers whose sum is b. If there are many factors to consider you may want to use the quadratic formula instead.
Example 1: Get the values of x for the equation 2x2 – 14x + 20 = 0
Step 1: Find common factors if you can.
2x2 – 14x + 20 = 2(x2 – 7x + 10)
Step 2: Find the factors of (x2 – 7x + 10)
List out the factors of 10:
We need to get the negative factors of 10 to get a negative sum.
–1 × –10, –2 × –5
Step 3: Find the factors whose sum is – 7:
1 + (–10) ≠ –7
–2 + (–5) = –7 ![]()
Step 4: Write out the factors and check using the distributive property.
2(x – 2) (x – 5) = 2(x2 – 5 x – 2x + 10)
= 2(x2 – 7x + 10) = 2x2 – 14x + 20
Step 5: Going back to the original equation
2x2 – 14x + 20 = 0 Factorize the left hand side of the equation
2(x – 2) (x – 5) = 0
x - 2 = 0 ⇒ x = 2, or
x - 5 = 0 ⇒ x = 5
Example 2: Get the values of x for the equation 7x2 + 18x + 11 = 0
Step 1: List out the factors of 7 and 11
Factors of 7:
1 × 7
Factors of 11:
1 × 11
Since 7 and 11 are prime numbers there are only two possibilities to try out.
Step 2: Write down the different combinations of the factors and perform the distributive property to check.
(7x + 1)(x + 11) ≠ 7x2 + 18x + 11
(7x + 11)(x + 1) = 7x2 + 18x + 11 ![]()
Step 3: Write out the factors and check using the distributive property.
(7x + 11)(x + 1) = 7x2 + 7x + 11x + 11 = 7x2 + 18x + 11
Step 4: Going back to the original equation
7x2 + 18x + 11= 0 Factorize the left hand side of the equation
(7x + 11)(x + 1) = 0

Example 3: Get the values of x for the equation 4x2 + 26x + 12 = 0
Step 1: List out the factors of 4 & 12
Factors of 4:
1 × 4, 2 × 2
Factors of 12:
1 × 12, 2 × 6, 3 × 4
Step 2: Write down the different combinations of the factors and perform the distributive property to check. When there are many factors to check, this becomes a tedious method to solve such quadratic equations, so you may want to try the quadratic formula instead.
(4x + 12)(x + 1) ≠ 4x2 + 26x + 12
(4x + 12)(x + 12) ≠ 4x2 + 26x + 12
(4x + 2)(x + 6) ≠ 4x2 + 26x + 12
(4x + 6)(x + 2) ≠ 4x2 + 26x + 12
(4x + 3)(x + 4) ≠ 4x2 + 26x + 12
(4x + 4)(x + 3) ≠ 4x2 + 26x + 12
(2x + 12)(2x + 1) = 4x2 + 26x + 12 ![]()
(2x + 2)(2x + 6) ≠ 4x2 + 26x + 12
(2x + 3)(2x + 4) ≠ 4x2 + 26x + 12
Step 3: Going back to the original quadratic equation
4x2 + 26x + 12 = 0 Factorize the left side of the equation
(2x + 12)(2x + 1) = 0

Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.