Techniques For Factoring Polynomials
Related Pages
Factor Theorem
Solving Quadratic Equations
More Algebra Lessons
In these lessons, we will learn the different basic techniques for factoring polynomials.
This is part of a series of free Basic Algebra Lessons. Here, we shall cover
- how to factor a polynomial by factoring out the greatest common factor.
- how to factor a polynomial by grouping.
- how to factor difference of perfect squares.
- how to factor trinomials with a = 1
- how to factor trinomials with a > 1
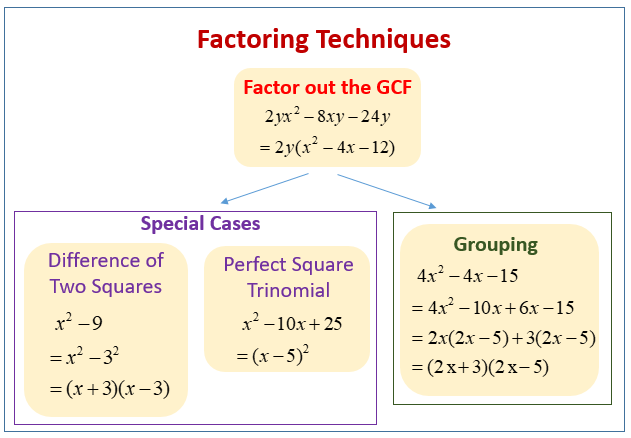
The following diagram shows some examples of Factoring Techniques. Scroll down the page for more examples and solutions of factoring techniques.
Printable & Online Algebra Worksheets
Greatest Common Factors
When working with polynomials and complex fractions, it’s important to understand and be able to find greatest common factors. Being able to find greatest common factors will help when factoring trinomials by grouping. When factoring, we can either find the greatest common factors of two integers or find the greatest common factors of two complex expressions.
Factoring out the greatest common factor.
Factor By Grouping
Difference Of Perfect Squares
An important special case when trying to factor polynomials is a identifying the difference of perfect squares. We learn to recognize a difference of perfect squares because they have a special, easily factored form. It’s also important to recognize the factored form to make the multiplication of the binomials easier. Easily recognizing the difference of perfect squares is useful when factoring quadratics that are not a difference of perfect squares.
Factoring The Difference Of Two Squares - Ex 1
Factoring The Difference Of Two Squares - Ex 2
Factoring The Difference Of Two Squares - Ex 3
Factoring Trinomials, a = 1
When given a trinomial, or a quadratic, it can be useful for purposes of canceling and simplifying to factor it. Factoring trinomials is easiest when the leading coefficient (the coefficient on the squared term) is one. A more complex situation is factoring trinomials when the leading coefficient is not one. To factor trinomials we use methods that involve finding the factors of their coefficients.
Factoring a Trinomial with Leading Coefficient of 1 - The Basics
Factoring Basic Trinomials, with a = 1
Factoring Trinomials With “a” Not Equal To One
One of the final steps in learning to factor trinomials is factoring trinomials with “a” not equal to one. In this case, “a” is the leading coefficient, or the coefficient of the squared term. When factoring trinomials with “a” not equal to one, in addition to using the methods used when “a” is one we must take the factors of “a” into account when finding the terms of the factored binomials.
This video provides examples of how to factor a trinomial when the leading coefficient is not equal to 1 by using the grouping method.
How To Factor A Trinomial When The Leading Coefficient Is Not Equal To 1 By Using The Trial And Error Method?
Examples of how to factor a trinomial when the leading coefficient is not equal to 1 by using the bottoms up method
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.