Fraction Equivalence Using Multiplication

Examples, solutions, and videos to help Grade 4 students learn how to use the area model and multiplication to show the equivalence of two fractions.

Common Core Standards: 4.NF.1, 4.NF.3b

New York State common Core Grade 4, Module 5, Lesson 7

Worksheets for Grade 4

The following diagram shows how to use the area model and multiplication to show the equivalence of two fractions. Scroll down the page for more examples and solutions.

 

Lesson 7 Concept Development
Problem 1: Determine that multiplying the numerator and denominator by n results in an equivalent fraction.
Problem 2: Given an area model, determine an equivalent fraction for the area selected.
Problem 3: Express an equivalent fraction using multiplication and verify by drawing an area model.

• Show Step-by-step Solutions

Lesson 7 Problem Set
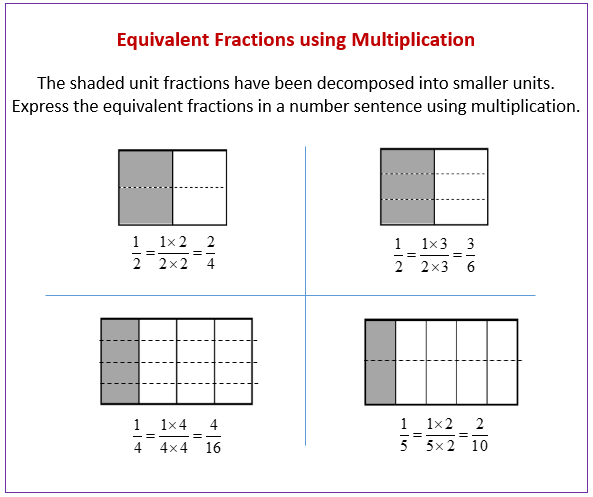
Each rectangle represents 1 whole.

1. The shaded unit fractions have been decomposed into smaller units. Express the equivalent fractions in a number sentence using multiplication. The first one has been done for you.
2. Decompose the shaded fractions into smaller units using the area models. Express the equivalent fractions in a number sentence using multiplication.
   e. What happened to the size of the fractional units when you decomposed the fraction?
   f. What happened to the total number of units in the whole when you decomposed the fraction?
3. Draw three different area models to represent 1 third by shading. Decompose the shaded fraction into (a) sixths, (b) ninths, and (c) twelfths. Use multiplication to show how each fraction is equivalent to 1 third.

• Show Step-by-step Solutions

Lesson 7 Homework
Each rectangle represents 1 whole.

1. The shaded unit fractions have been decomposed into smaller units. Express the equivalent fractions in a number sentence using multiplication. The first one has been done for you.
2. Decompose the shaded fractions into smaller units using the area models. Express the equivalent fractions in a number sentence using multiplication.
3. Draw three different area models to represent 1 fourth by shading.
   Decompose the shaded fraction into (a) eighths, (b) twelfths, and (c) sixteenths.
   Use multiplication to show how each fraction is equivalent to 1 fourth.

• Show Step-by-step Solutions

• Show Step-by-step Solutions

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.