Illustrative Mathematics Unit 6.4, Lesson 12: Fractional Lengths

Related Pages
Illustrative Math
Grade 6

Lesson 12: Fractional Lengths

Let’s solve problems about fractional lengths.

Illustrative Math Unit 6.4, Lesson 12 (printable worksheets)

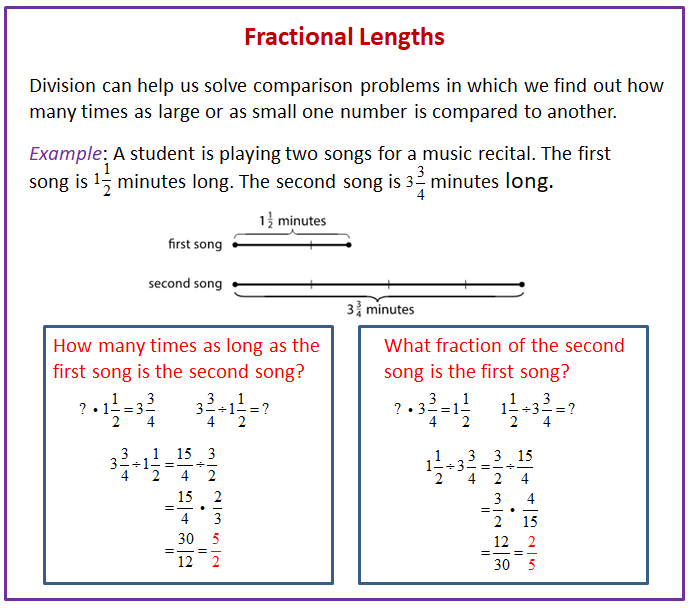
Lesson 12 Summary

The following diagram shows how to use division and multiplication to solve problems involving fractional lengths.

Lesson 12.1 Number Talk: Multiplication Strategies

Find the product mentally.
19 ÷ 14

• See Video for Whole Lesson

Scroll down the page for the answer to the “Are you ready for more?” section.

Lesson 12.2 How Many Would It Take? (Part 1)

1. Jada was using square stickers with a side length of ¾ inch to decorate the spine of a photo album. The spine is 10½ inches long. If she laid the stickers side by side without gaps or overlaps, how many stickers did she use to cover the length of the spine?
2. How many ⅝-inch binder clips, laid side by side, make a length of 11¼ inches?
3. It takes exactly 26 paper clips laid end to end to make a length of 17 7/8 inches.
   a. Estimate the length of each paper clip.
   b. Calculate the length of each paper clip. Show your reasoning.
   

Are you ready for more?

Lin has a work of art that is 14 inches by 20 inches. She wants to frame it with large paper clips laid end to end.

1. If each paper clip is 1¾ inch long, how many paper clips would she need? Show your reasoning and be sure to think about potential gaps and overlaps. Consider making a sketch that shows how the paper clips could be arranged.
   • Show Answer

     On one side, we can put 14 ÷ 1¾ = 8 clips
     On the other side, we can put 20 ÷ 1¾ = 11 clips with a gap of 3/7 inch. Altogether, we can arrange 8 + 11 + 8 + 11 = 38 clips.

2. How many paper clips are needed if the paper clips are spaced ¼ inch apart? Describe the arrangement of the paper clips at the corners of the frame.
   • Show Answer

     If we leave a gap of ¼ then each clip will take a space of 1¾ + ¼ = 2 inches
     On one side, we can put 14 ÷ 2 = 7 clips
     On the other side, we can put 20 ÷ 2 = 10 clips
     Altogether, we can arrange 7 + 10 + 7 + 10 = 34 clips.

Lesson 12.3 How Many Times as Tall or as Far?

1. A second-grade student is 4 feet tall. Her teacher is 5⅔ feet tall.
   a. How many times as tall as the student is the teacher?
   b. What fraction of the teacher’s height is the student’s height?
   
2. Find each quotient. Show your reasoning and check your answer.
   a. 9 ÷ ⅗
   b. 1⅞ ÷ ¾
   
3. Write a division expression that can help answer each of the following questions. Then answer the question. If you get stuck, draw a diagram.
   a. A runner ran 1⅘ miles on Monday and 6 3/10 miles on Tuesday. How many times her Monday’s distance was her Tuesday’s distance?
   b. A cyclist planned to ride 9½ miles but only managed to travel 3⅞ miles. What fraction of his planned trip did he travel?

Lesson 12.4 Comparing Paper Rolls

The photo shows a situation that involves fractions.

• Show Paper Rolls

  

1. Use the photo to help you complete the following statements. Explain or show your reasoning for the second statement.
   a. The length of the long paper roll is about ______ times the length of the short paper roll.
   b. The length of the short paper roll is about ______ times the length of the long paper roll.
   
2. If the length of the long paper roll is 11¼ inches, what is the length of each short paper roll?
   Use the information you have about the paper rolls to write a multiplication equation or a division equation for the question. Note that 11¼ = 45/4.
   
3. Answer the question. If you get stuck, draw a diagram.

Lesson 12 Practice Problems

1. One inch is around 2 11/20 centimeters.
   a. How many centimeters long is 3 inches? Show your reasoning.
   b. What fraction of an inch is 1 centimeter? Show your reasoning.
   c. What question can be answered by finding 10 ÷ 2 11/20?
   
2. A zookeeper is 6¼ feet tall. A young giraffe in his care is 9⅜ feet tall.
   a. How many times as tall as the zookeeper is the giraffe? ​
   b. What fraction of the giraffe’s height is the zookeeper’s height?
   
3. A rectangular bathroom floor is covered with square tiles that are 1½ feet by 1½ feet. The length of the bathroom floor is 10½ feet and the width is 6½ feet.
   a. How many tiles does it take to cover the length of the floor?
   b. How many tiles does it take to cover the width of the floor?
   
4. The Food and Drug Administration (FDA) recommends a certain amount of nutrient intake per day called the “daily value.” Food labels usually show percentages of the daily values for several different nutrients—calcium, iron, vitamins, etc.
   In ¾ cup of oatmeal, there is 1/10 of the recommended daily value of iron. What fraction of the daily recommended value of iron is in 1 cup of oatmeal?
   Write a multiplication equation and a division equation to represent the question, and then answer the question. Show your reasoning.
   
5. What fraction of ½ is ⅓ ? Draw a tape diagram to represent and answer the question. Use graph paper if needed.
   
6. Noah says, “There are 2½ groups of ⅘ in 2.” Do you agree with his statement? Draw a tape diagram to show your reasoning. Use graph paper, if needed.
   
   
   
   

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.