GCF, Distributive Property (Grade 6)
Related Pages
Greatest Common Factor
Number Properties
Common Core for Grade 6
Grade 6 Math
In these lessons, Grade 6 students learn to find the greatest common factor of two whole numbers less than or equal to 100 and the least common multiple of two whole numbers less than or equal to 12.
Use the distributive property to express a sum of two whole numbers 1–100 with a common factor as a multiple of a sum of two whole numbers with no common factor.
For example, express 36 + 8 as 4 (9 + 2).
Common Core: 6.NS.4
Suggested Learning Targets
- I can find the greatest common factor and least common multiple.
- I can use the distributive property for whole numbers with no common factor.
- I can apply the Distributive Property to rewrite addition problems by factoring out the Greatest Common Factor.
Component Skills from Previous Grades
| 3.OA.5 Apply properties of operations as strategies to multiply and divide. For example, write 8 × 7 as 8 × (5 + 2) = (8 × 5) + (8 × 2) = 40 + 16 = 56. (Distributive property.) |
GCF & LCM Find the greatest common factor of two whole numbers less than or equal to 100 and the least common multiple of two whole numbers less than or equal to 12. |
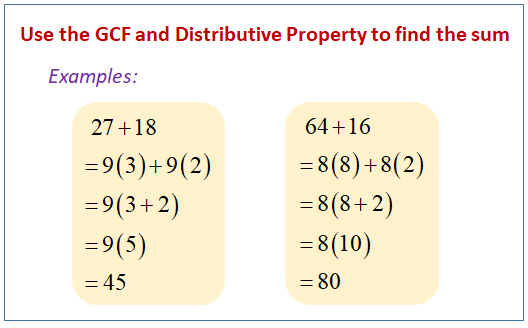
The following diagram shows how to use the GCF and Distributive Property to find the sum of two numbers. Scroll down the page for more examples and solutions on GCF and distributive property.
Printable & Online Factor Worksheets
Greatest Common Factor (GCF)
GCF and Distributive Property
6NS4 - Distributive Property
Example:
3(2 + 6)
6NS4 - Multiple of a Sum
How to write a sum of two whole numbers as a multiple of a sum of two whole numbers?
GCF and Distributive Property
Example:
Use the GCF and the distributive property to express a sum as a product.
20 + 35
Example:
Use the GCF and the Distributive Property to express the sum as a product
18 + 27
GCF and the Distributive Property
Step 1: Find the GCF of the 2 numbers
Step 2: Re-write using the distributive property.
Example:
Use the GCF and the Distributive Property to express the sum.
36 + 8
27 + 18
25 + 60
Example:
Apply the distributive property to the algebraic expression.
24x + 18y
10x + 15
16x + 32y
GCF And Distributive Property Word Problems
Learn how to use solve problems using the GCF and Distributive Property
Example:
Bryan is setting chairs for a graduation ceremony. He has 50 black chairs and 60 white chairs.
Each row will have the same number of chairs and and each row will have the same color chair.
What is the greatest number of chairs that he can fit in each row?
How many rows of each color chair will there be?
Use the GCF and Distributive Property to solve word problems
Example:
A store clerk is bagging spices. He has 18 teaspoons of cinnamon and 30 teaspoons of nutmeg.
Each bag need to contain the same number of teaspoons and each bag can contain only one spice.
How many teaspoons of spice should the clerk put in each bag?
How many bags of each spice would there be?
GCF and Distributive Property Problem Solving
Learn how to use the GCF in problem solving. Distinguish how to use the distributive property in problem solving.
Example:
Madison has 56 roses and 42 daisies to use in floral centerpieces for a party. Each centerpiece
will have the same number of flowers and will contain only roses or only daisies.
What is the greatest number of flowers that Madison can use in each centerpiece?
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.
We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.