Illustrative Mathematics Grade 8, Unit 5, Lesson 6: Even More Graphs of Functions

Related Pages
Illustrative Math
Grade 8

Lesson 6: Even More Graphs of Functions

Let’s draw a graph from a story.

Illustrative Math Unit 8.5, Lesson 6 (printable worksheets)

Lesson 6 Summary

The following diagram shows how to interpret the graph of a function.

Lesson 6.1 Dog Run

Here are five pictures of a dog taken at equal intervals of time. Diego and Lin drew different graphs to represent this situation: They both used time as the independent variable. What do you think each one used for the dependent variable? Explain your reasoning.

• See Video for Whole Lesson

Lesson 6.2 Which Graph is It?

For each situation,

• name the independent and dependent variables
• pick the graph that best fits the situation, or sketch the graph if one isn’t provided label the axes
• answer the question: which quantity is a function of which? Be prepared to explain your reasoning.

1. Jada is training for a swimming race. The more she practices, the less time it takes for her to swim one lap.
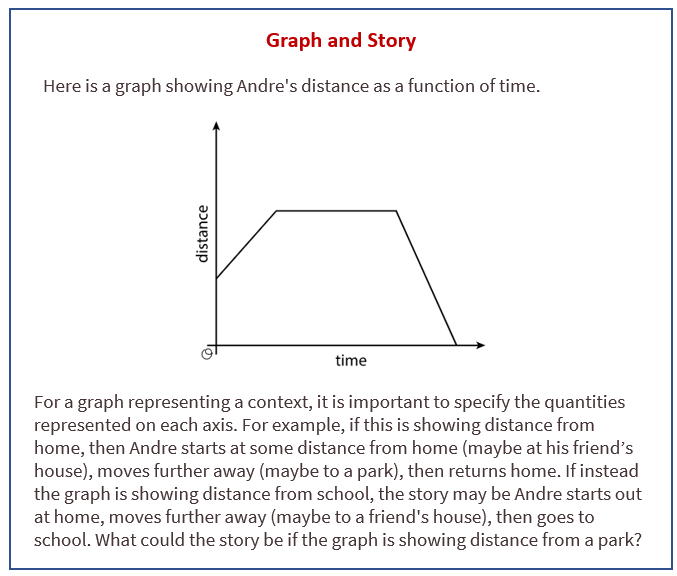
2. Andre adds some money to a jar in his room each week for 3 weeks and then takes some out in week 4.

Lesson 6.3 Sketching a Story about a Boy and a Bike

You will use the tools in the applet for creating a visual display that shows your response to each question.
Here is a story: “Noah was at home. He got on his bike and rode to his friend’s house and stayed there for awhile. Then he rode home again. Then he rode to the park. Then he rode home again.”

1. Sketch a graph of this story.
2. What are the two quantities? Label the axes with their names and units of measure. (For example, if this were a story about pouring water into a pitcher, one of your labels might say “volume (liters).”)
3. Which quantity is a function of which? Explain your reasoning.
4. Based on your graph, is his friend’s house or the park closer to Noah’s home? Explain how you know.
5. Read the story and all your responses again. Does everything make sense? If not, make changes to your work.
   Open Applet

Are you ready for more?

It is the year 3000. Noah’s descendants are still racing around the park, but thanks to incredible technological advances, now with much more powerful gadgets at their disposal. How might their newfound access to teleportation and time-travel devices alter the graph of stories of their daily adventures? Could they affect whether or not the distance from home is a function of the time elapsed?

Lesson 6 Practice Problems

1. Match the graph to the following situations (you can use a graph multiple times). For each match, name possible independent and dependent variables and how you would label the axes.
   a. Tyler pours the same amount of milk from a bottle every morning.
   b. A plant grows the same amount every week.
   c. The day started very warm but then it got colder.
   d. A carnival has an entry fee of $5 and tickets for rides cost $1 each.
2. Jada fills her aquarium with water.
   The graph shows the height of the water, in cm, in the aquarium as a function of time in minutes. Invent a story of how Jada fills the aquarium that fits the graph.
3. Recall the formula for area of a circle.
   a. Write an equation relating a circle’s radius, r, and area, A.
   b. Is area a function of the radius? Is radius a function of the area?
   c. Fill in the missing parts of the table
4. The points with coordinates (4,6), (2,10), and (5,7) all lie on the line 2x + 2y = 24.
   a. Create a graph, plot the points, and sketch the line.
   b. What is the slope of the line you graphed?
   c. What does this slope tell you about the relationship between lengths and widths of rectangles with perimeter 24?

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.