Illustrative Mathematics Grade 8, Unit 5, Lesson 4: Tables, Equations, and Graphs of Functions

Related Pages
Illustrative Math
Grade 8

Lesson 4: Tables, Equations, and Graphs of Functions

Let’s connect equations and graphs of functions.

Illustrative Math Unit 8.5, Lesson 4 (printable worksheets)

Lesson 4 Summary

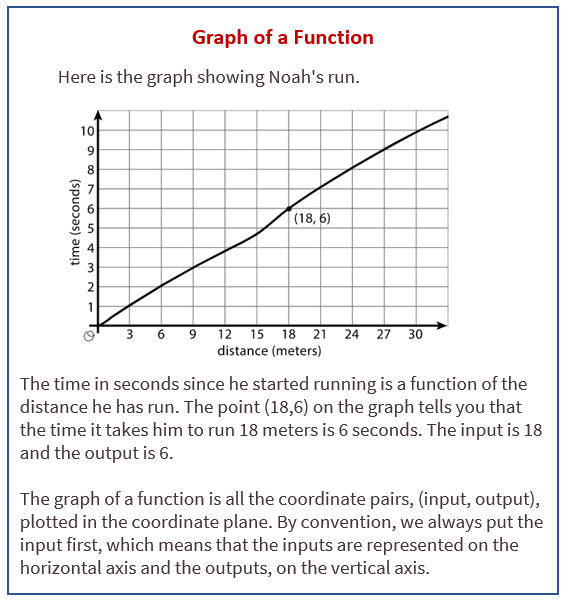
The following diagram shows how to name the independent and dependent variables for a given function and represent the function with an equation.

Lesson 4.1 Notice and Wonder: Doubling Back

What do you notice? What do you wonder?

• See Video for Whole Lesson

Lesson 4.2 Equations and Graphs of Functions

The graphs of three functions are shown.

1. Match each of these equations to one of the graphs. a. d = 60t, where d is the distance in miles that you would travel in t hours if you drove at 60 miles per hour.
   b. q = 50 - 0.4d, where is the number of quarters, and is the number of dimes, in a pile of coins worth $12.50.
   c. A = πr², where A is the area in square centimeters of a circle with radius r centimeters.
2. Label each of the axes with the independent and dependent variables and the quantities they represent.
3. For each function: What is the output when the input is 1? What does this tell you about the situation? Label the corresponding point on the graph.
4. Find two more input-output pairs. What do they tell you about the situation? Label the corresponding points on the graph.

Are you ready for more?

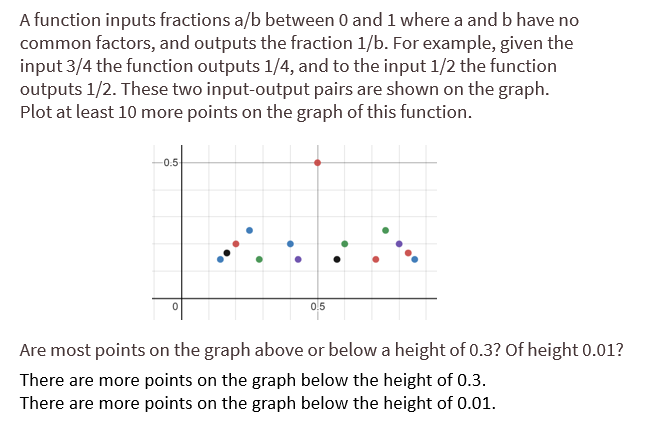
A function inputs fractions a/b between 0 and 1 where a and b have no common factors, and outputs the fraction 1/b. For example, given the input 3/4 the function outputs 1/4, and to the input 1/2 the function outputs 1/2. These two input-output pairs are shown on the graph.
Plot at least 10 more points on the graph of this function. Are most points on the graph above or below a height of 0.3? Of height 0.01?

• Show Answer

  

Lesson 4.3 Running around a Track

1. Kiran was running around the track. The graph shows the time, t, he took to run various distances, d. The table shows his time in seconds after every three meters.
   a. How long did it take Kiran to run 6 meters?
   b. How far had he gone after 6 seconds?
   c. Estimate when he had run 19.5 meters.
   d. Estimate how far he ran in 4 seconds.
   e. Is Kiran’s time a function of the distance he has run? Explain how you know.
2. Priya is running once around the track. The graph shows her time given how far she is from her starting point.
   a. What was her farthest distance from her starting point?
   b. Estimate how long it took her to run around the track.
   c. Estimate when she was 100 meters from her starting point.
   d. Estimate how far she was from the starting line after 60 seconds.
   e. Is Priya’s time a function of her distance from her starting point? Explain how you know.

Lesson 4 Practice Problems

1. The graph and the table show the high temperatures in a city over a 10-day period. a. What was the high temperature on Day 7?
   b. On which days was the high temperature 61 degrees?
   c. Is the high temperature a function of the day? Explain how you know.
   d. Is the day a function of the high temperature? Explain how you know.
2. The amount Lin’s sister earns at her part-time job is proportional to the number of hours she works. She earns $9.60 per hour.
   a. Write an equation in the form y=kx to describe this situation, where x represents the hours she works and y represents the dollars she earns.
   b. Is y a function of x? Explain how you know.
   c. Write an equation describing x as a function of y.
3. Use the equation 2m + 4s = 16 to complete the table, then graph the line using s as the dependent variable.
4. Solve the system of equations:

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.