Illustrative Mathematics Grade 7, Unit 4, Lesson 4: Half as Much Again

Related Pages
Illustrative Math
Grade 7

Lesson 4: Half as Much Again

Let’s use fractions to describe increases and decreases.

Illustrative Math Unit 7.4, Lesson 4 (printable worksheets)

Lesson 4 Summary

The following diagram shows what “half as much again” means and how to use the distributive property to rewrite an expression.

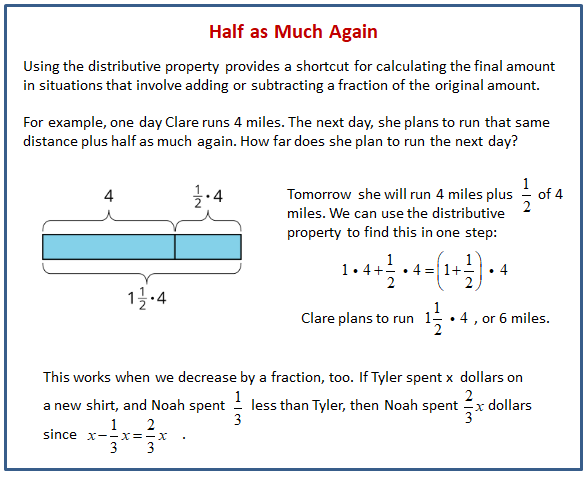
Lesson 4.1 Notice and Wonder: Tape Diagrams

What do you notice? What do you wonder?

• See Video for Whole Lesson

Lesson 4.2 Walking Half as Much Again

1. Complete the table to show the total distance walked in each case.
   a. Jada’s pet turtle walked 10 feet, and then half that length again.
   b. Jada’s baby brother walked 3 yards, and then half that length again.
   c. Jada’s hamster walked 4.5 km, and then half that length again.
   d. Jada’s robot walked 1 mile, and then half that length again.
   e. A person walked x meters and then half that length again.
   
2. Explain how you computed the total distance in each case.
   Two students each wrote an equation to represent the relationship between the initial distance walked (x) and the total distance walked (y).
   Mai wrote y = x + 1/2 x.
   Kiran wrote y = 3/2 x.
   Do you agree with either of them? Explain your reasoning.
   

Are you ready for more?

Zeno jumped 8 meters. Then he jumped half as far again (4 meters). Then he jumped half as far again (2 meters). So after 3 jumps, he was 8 + 4 + 2 = 14 meters from his starting place.

1. Zeno kept jumping half as far again. How far would he be after 4 jumps? 5 jumps? 6 jumps?
   • Show Answer

     4 jumps: 8 + 4 + 2 + 1 = 15 meters
     5 jumps: 8 + 4 + 2 + 1 + 1/2 = 15 1/2 meters
     6 jumps: 8 + 4 + 2 + 1 + 1/2 + 1/4 = 15 3/4 meters
     

2. Before he started jumping, Zeno put a mark on the floor that was exactly 16 meters from his starting place. How close can Zeno get to the mark if he keeps jumping half as far again? (Consider researching Zeno’s Paradox.)
   • Show Answer

     He will get closer and closer to 16 meters but will never reach 16 meters.

Lesson 4.3 More and Less

1. Match each situation with a diagram. A diagram may not have a match.
   a. Han ate x ounces of blueberries. Mai ate 1/3 less than that.
   b. Mai biked x miles. Han biked 2/3 more than that.
   c. Han bought x pounds of apples. Mai bought 2/3 of that.
   
2. For each diagram, write an equation that represents the relationship between x and y.
   a. Diagram A:
   b. Diagram B:
   c. Diagram C:
   d. Diagram D:
   
3. Write a story for one of the diagrams that doesn’t have a match.
   

Lesson 4.4 Card Sort: Representations of Proportional Relationships

Your teacher will give you a set of cards that have proportional relationships represented 3 different ways: as descriptions, equations, and tables. Mix up the cards and place them all face-up.

1. Take turns with a partner to match a description with an equation and a table.
   a. For each match you find, explain to your partner how you know it’s a match.
   b. For each match your partner finds, listen carefully to their explanation, and if you disagree, explain your thinking.
   
2. When you agree on all of the matches, check your answers with the answer key. If there are any errors, discuss why and revise your matches.
   

Lesson 4 Practice Problems

1. Match each situation with a diagram.
   
2. Elena walked 12 miles. Then she walked that distance. How far did she walk all together? Select all that apply.
   A. 12 ÷ 1/4
   B. 12 · 1/4
   C. 12 + 1/4 · 12
   D. 12 (1 + 1/4)
   E. 12 · 3/4
   F. 12 · 5/4
   
3. Write a story that can be represented by the equation y = x + 1/4 x.
   
4. Select all ratios that are equivalent to 4:5.
   A: 2:2.5
   B. 2:3
   C. 3:3.75
   D. 7:8
   E. 8:10
   F. 14:27.5
   
5. Jada is making circular birthday invitations for her friends. The diameter of the circle is 12 cm. She bought 180 cm of ribbon to glue around the edge of each invitation. How many invitations can she make?

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.