Illustrative Mathematics Grade 6, Unit 8, Lesson 8: Describing Distributions on Histograms

Related Pages
Illustrative Math
Grade 6

Lesson 8: Describing Distributions on Histograms

Let’s describe distributions displayed in histograms.

Illustrative Math Unit 6.8, Lesson 8 (printable worksheets)

Lesson 8 Summary

The following diagram describes the shape and features of a histogram and explain what they mean in the context of the data.


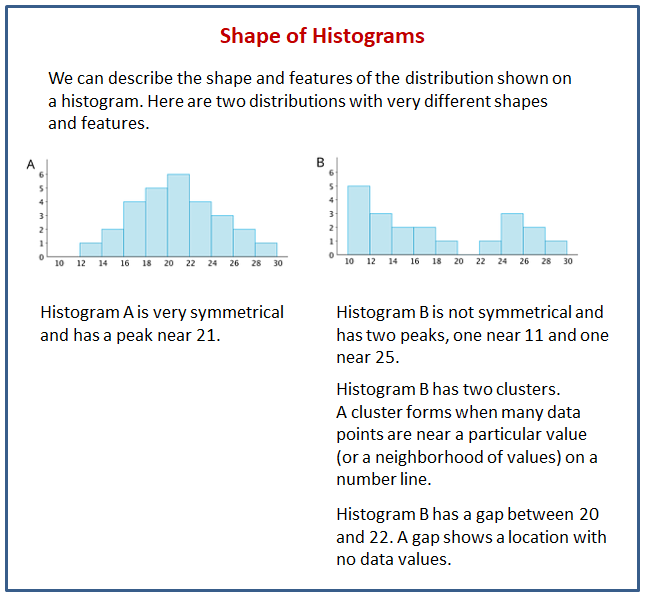
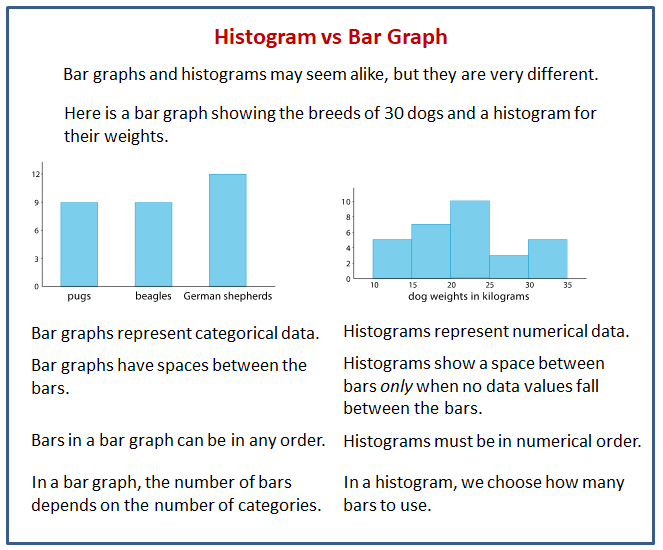
The following diagram shows the differences between a histogram and a bar graph.

Lesson 8.1 Which One Doesn’t Belong: Histograms

Which histogram does not belong? Be prepared to explain your reasoning.

• See Video for Whole Lesson

Lesson 8.2 Sorting Histograms

1. Your teacher will give your group a set of histogram cards. Sort them into two piles—one for histograms that are approximately symmetrical, and another for those that are not.
   
2. Discuss your sorting decisions with another group. Do both groups agree which cards should go in each pile? If not, discuss the reasons behind the differences and see if you can reach agreement. Record your final decisions.

• Histograms that are approximately symmetrical:
  
• Histograms that are not approximately symmetrical:
  

3. Histograms are also described by how many major peaks they have. Histogram A is an example of a distribution with a single peak that is not symmetrical.
   Which other histograms have this feature?
   
4. Some histograms have a gap, a space between two bars where there are no data points. For example, if some students in a class have 7 or more siblings, but the rest of the students have 0, 1, or 2 siblings, the histogram for this data set would show gaps between the bars because no students have 3, 4, 5, or 6 siblings.
   Which histograms do you think show one or more gaps?
   
5. Sometimes there are a few data points in a data set that are far from the center. Histogram A is an example of a distribution with this feature.
   Would you describe any of the other histograms as having this feature? If so, which ones?
   

Lesson 8.3 Getting to School

Your teacher will provide the data that your class collected on how students travel to school and their travel times.

1. Use the data to draw a histogram that shows your class’s travel times.
   
2. Write a couple of sentences to describe the distribution of travel times. Comment on the center and spread of the data, as well as the shape and features.
   
3. Use the data on methods of travel to draw a bar graph. Include labels for the horizontal axis.
   
4. Write 2–3 sentences to describe what you learned about your class’s methods of transportation to school. Comment on any patterns you noticed.
   
5. Compare the histogram and the bar graph that you drew. Discuss your thinking with your partner:

• How are they alike?
• How are they different?
  

Are you ready for more?

Use one of these suggestions (or make up your own). Research data to create a histogram. Then, describe the distribution.

• Heights of 30 athletes from multiple sports
• Heights of 30 athletes from the same sport
• High temperatures for each day of the last month in a city you would like to visit
• Prices for all the menu items at a local restaurant
  

Lesson 8 Practice Problems

1. The histogram summarizes the data on the body lengths of 143 wild bears. Write a few sentences describing the distribution of body lengths.
   Be sure to comment on the shape, center, and spread of the distribution.
   
2. Which data set is more likely to produce a histogram with a symmetric distribution? Explain your reasoning.
   Data on the number of seconds on a track of music in a pop album.
   Data on the number of seconds spent talking on the phone yesterday by everyone in the school.
   
3. Decide if each data set might produce one or more gaps when represented by a histogram. For each data set that you think might produce gaps, briefly describe or give an example of how the values in the data set might do so.
   a. The ages of students in a sixth-grade class.
   b. The ages of people in an elementary school.
   c. The ages of people eating in a family restaurant.
   d. The ages of people who watch football.
   e. The ages of runners in a marathon.
   
4. Evaluate the expression 4x³ for each value of x.
   a. 1
   b. 2
   c. 1/2
   
5. Jada drank 12 ounces of water from her bottle. This is 60% of the water the bottle holds.
   a. Write an equation to represent this situation. Explain the meaning of any variables you use.
   b. How much water does the bottle hold?
   

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.